Before I start, we have to agree on what Huygens’ principle says. The Wikipedia page you cite does a poor job at presenting Huygens’ original idea, instead entirely focusing on Fresnel’s addition to it (the article is titled Huygens-Fresnel principle after all). You may know what I am going to explain but it may be still be useful to clarify for other readers.
If we go down the route of Fresnel modifications, it is rather pointless to stop there as Fresnel’s theory is just a bunch of heuristic guesses to make Huygens’ idea work for diffraction: instead we should go directly to Kirchhoff’s formula which is grounded in the rigorous treatment of the wave equation. As for Huygens’ original principle, it states
- that every point on the wave front of a propagating wave (the primary wave) is the source of secondary spherical wavelets; and
- that the envelope of the wave fronts of all those secondary waves is the wave front of the primary wave.
A key point is that all those waves propagate at the same velocity. This is clearer on a drawing.
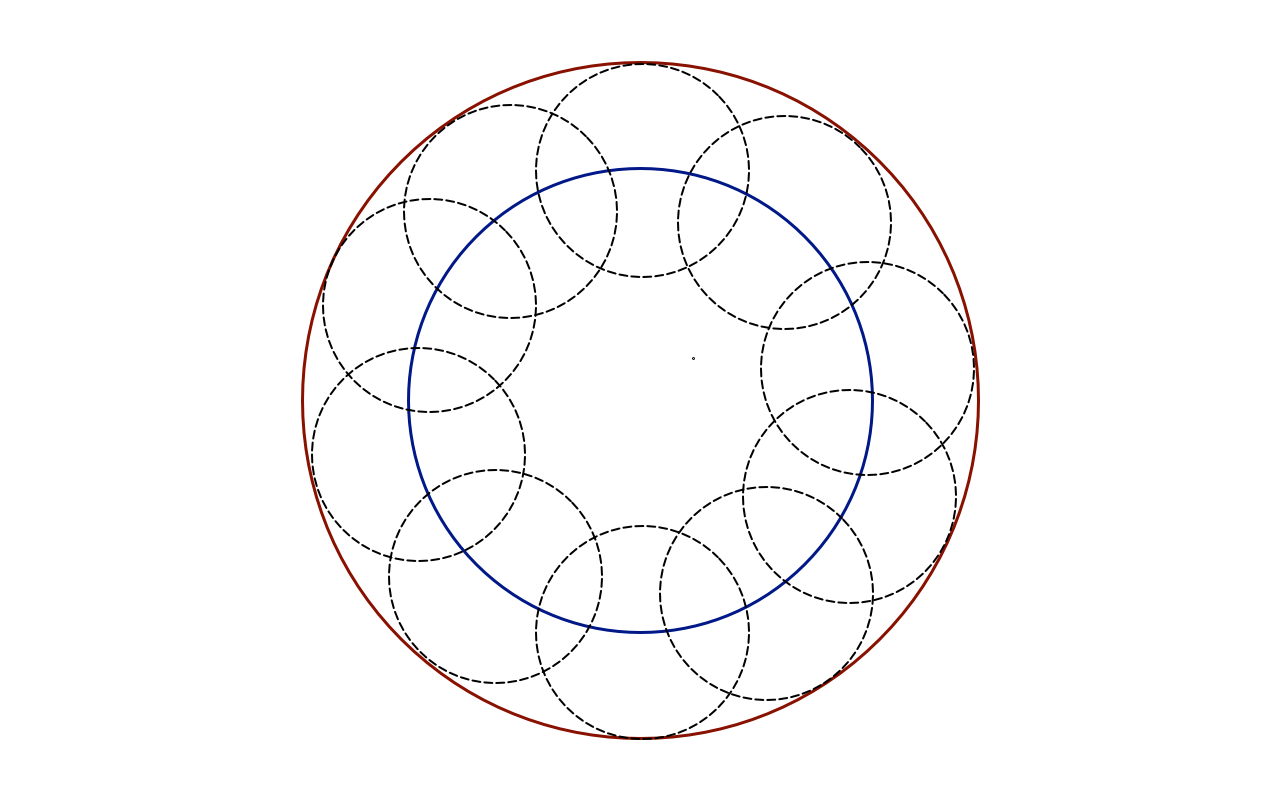
The blue circle is the primary wave front, the dotted circles are the wave fronts of the secondary wavelets and the red circle is the envelope. With this idea, Huygens was able to explain the laws of reflection and refraction but this method fails at explaining diffraction, not without Fresnel’s additions at least.
However we have a big problem: you considered surface waves but Huygens’ principle is not true in 2 dimensions! It is only true in odd dimensions. The only explanation of that I know of requires to study the spherical wave equation. I won’t elaborate on that but a nice exposition can be found on Kevin Brown’s famous mathpages. At least the first half is understandable with a bit of knowledge in calculus. There was also this question on our beloved physics.stackexchange, with answers at various levels of math.
Thus, I shall consider sound waves propagating in the atmosphere instead, in the presence of a steady uniform wind, so that I can work in 3D. In effect, since I will not write any math, it won’t make much difference to the exposition but at least you can feel assured that this is correct!
Now let’s consider an observer, moving at the same velocity as the wind, who claps his hands. By the principle of relativity, what he sees should then be the same as what he would have seen standing still on the ground if there was no wind: the wave fronts are concentric sphere expanding outward and the original Huygens' principle is valid. But now what we are interested in is what another observer standing still on the ground will see.
The essential point is that a wave front of the primary wave is still a sphere but one that is moving with the velocity of the wind. Similarly, the wave front of the secondary wavelets emitted on the primary wave front are also still spheres but moving with the wind speed. This is just a simple Galilean transformation from the frame of the observer moving along with the wind to the frame of the ground. As a result, the envelope of those secondary wave fronts will be the primary wave front further away, and we can conclude that the original Huygens’ principle hold for that observer standing still on the ground.
Owing to the discussion at the beginning of my answer, there is still the question of whether the improved Huygens' principles hold in the presence of the wind, specifically, whether Kirchhoff's formula does. The answer is that the standard form of it does not but that a tweaked version of it can be proven to work [Mor30].
[Mor30] W.R. Morgans. XIV. the Kirchhoff formula extended to a moving surface. The London, Edinburgh, and Dublin Philosophical Magazine and Journal of Science, 9(55):141–161, 1930.

