Huygens' principle: Its correct interpretation, and why there is no destructive interference in every wave? (unsatisfied with current answers)

Question

My understanding of Huygens' principle is, that it describes the way how a wave moves: Instead of moving straightforward, it propagates in all directions via producing secondary wavelets. 'Secondary wavelets' and 'wave's propagation' are synonyms.

But I realized that many others assume that waves move straight, and Huygens' principle doesn't say anything about the original wave, just that in addition to the original wave that moves straight, there are also secondary wavelets which are spherical. And these wavelets just continue straight, because Huygens' principle only applies to waves, not wavelets. I wonder if it could be the correct interpretation, because it seems to void most of Huygens' principle. For example, if refraction occurs due to Huygens' principle, refracted waves shouldn't be able to refract again, because they are composed of those wavelets, since the original wave just continues straight. So it's not a 'real' wave, and Huygens' principle doesn't apply to it.

Now to my main question (with my understanding of Huygens' principle): Let's start with the most simple case: propagation of waves in free space. Suppose we have a planar wavefront of troughs followed by a wavefront of crests of the same waves (½ wavelength behind). After one revolution, we have a lot of semicircular secondary wavefronts superimposed on each other all along the initial wavefront. (We should really have full spherical wavefronts, but for now let's ignore backward waves, and focus on 2 dimensions only, considering only the forward semicircles. It's enough complicated as is.) The result is a thick wavefront starting from the location where the wavefront initially was, and ending one wavelength ahead. After another revolution, we have a wavefront twice as thick. The starting point never changes (as long as we ignore backward waves), but the endpoint does, so the wavefront is just growing. The same is true for the wave crests (and everything in between), just their start and end points are a half wavelength behind. So behind the very first part of the waves - where the troughs have no crests to compete with - we should have destructive interference in every wave.

Here is how it should look after one revolution: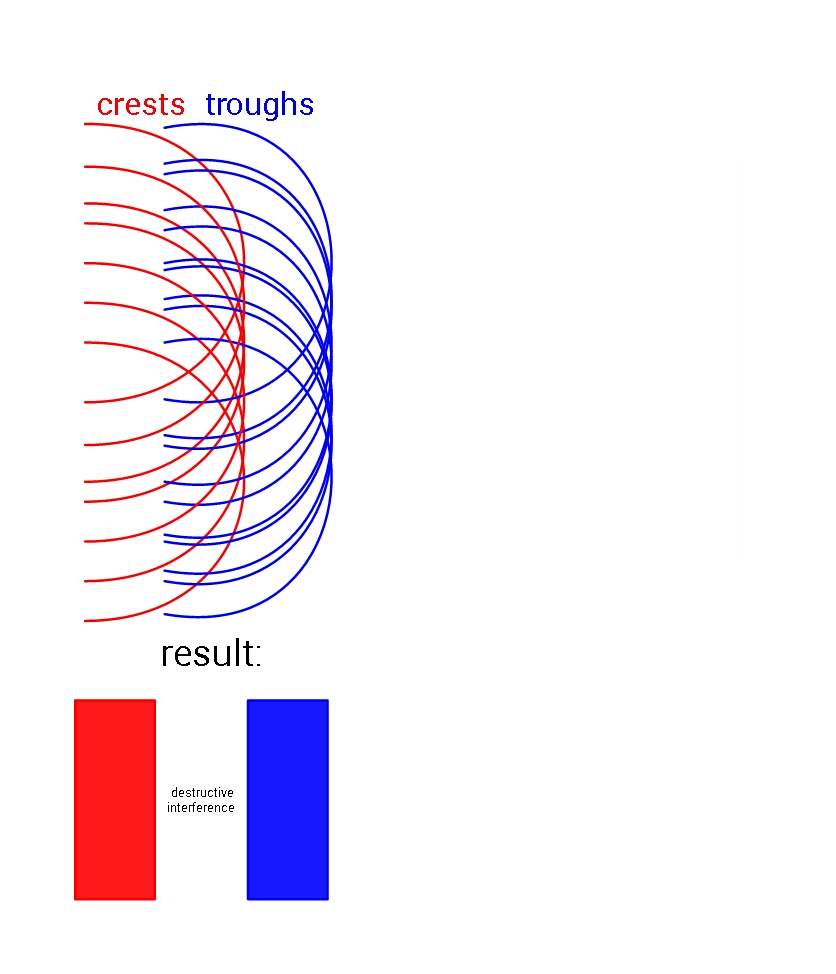 Whatever the answer should be, one needs to be careful that only straightforward waves should survive. And I'm not concerned specifically about backward waves (and I have no idea why people assumed so), but about every possible direction.

I think that the answer may be that although there are troughs and crests all over, they have different densities, which causes that some parts should survive. But I'm waiting for others to confirm it before diving in the details.

When it comes to refraction, the problem with direction gets worse. If every point of a wavefront produces waves in every direction with and without refraction, then how can waves change direction? What exactly is different after refraction than before?

The question gets even more complicated, when we have refraction and diffraction simultaneously. In that case the side waves don't get canceled (this is the cause for diffraction), so how can refraction have an effect?

Some commenters told me that Huygens' principle is not accurate. It's just an approximation. I wonder if they're right, because I didn't see such a statement anywhere. If they're right, then I'm not interested in understanding Huygens' principle, because I'm interested in the real facts of wave propagation.

One of them told me that the exact truth is Maxwell's equations. But I couldn't find an interpretation of Maxwell's equations which predicts wave propagation, at least not a well explained one.

This is really a fundamental question. If you know of an article or inexpensive ebook etc. that explains this in a way that all my questions will be answered, please give me a link. (in addition to, or without, your answer.) (It's not easy to find. I searched a lot before posting this question.)

Answer 1

Imagine a simple plane wave, moving to the right, in a direction perpendicular to the wavefronts (i.e., to the "isophase planes"). Huygens wavelets emitted at all points on a given wavefront interfere constructively with each other in the forward direction, as you know. They never interfere with the wavelets from another wavefront in the same wave train, because they are moving at the same velocity as the wave train.

You want to know why there is destructive interference in the backward direction. To reach that understanding, you need to introduce something that accounts for the fact that the wave is actually moving.

The Huygens principle as it is usually presented can easily lead to confusion. The normal presentation begins with the assumption that every wave is actually a monochromatic wave train that starts out stationary. If that were true, there would be waves moving in both the forward and backward directions.

So, now re-do Huygens principle, taking time into account. In the backward (left) direction, an emitting point on the forward moving wavefront encounters backward-moving wavelets emitted by the forward-moving wavefront to the right of it, because it has moved to the right. The encounter is slightly too soon to be in step, because of the fact that the backward-moving wavelet travels less than a full wavelength before hitting the advancing left wavefront. Add that up over all the wavelets emitted by all points in the wavefronts ahead of it, and the sum washes out to zero: that is destructive interference, so this version of Huygens principle does not produce a backward-moving wavefront.

Edited 10/1/20

Answer 2

The wave equation has two initial conditions: the initial displacement, and the initial speed of the initial displacement. If the initial speed of the initial displacement is given the appropriate value then the backward wave is canceled. As an ongoing wave propagates this happens 'automatically' so there are no backward waves in an ongoing wave.

See my

https://www.researchgate.net/publication/340085346

Huygens' Principle geometric derivation and elimination of the wake and backward wave, rev2, 3/21/20

especially the appendices, particularly appendix D. {sorry for the math--hope its ok}

Now there is a peer reviewed version (Nature Scientific Reports):

https://www.nature.com/articles/s41598-021-99049-7

Answer 3

You can find an explanation by fermilab about the 'true' way light works. In his explanation, he tells that although there is constructive interference that creates correct waves in the forward direction in Huygens-Fresnel principle, it also predicts there are waves that propagates to the sides, which is invalid(unless talking about quantum probability).

Although, it doesn't explain about reflection, just about refraction, but it still gives you the correct idea about why light does these things. In his explanation about refraction, you need to understand that light is also an electromagnetic wave, and everything is a wave according to the quantum field theory, focus on the electric part, when light enters a medium, the electric wave of the light interacts with the electrons of the medium, causing the electrons to vibrate, this creates a new electric wave which combines with the light wave to create a brand new wave that is a brand new sort of quasi particle which has mass, this is why it slows down when entering a medium, since it is basically transformed into another entity. When it escapes the influence of the electrons, the wave will go back to normal and turns back into regular light.

In another video of his, he also explains the bending property of refraction in light, he said that it is also caused by the same property as to why light slows down. You see, in light, there are two waves that must be perpendicular to each other, these two waves combine into light. I have explained before that light has an electric wave which effects electrons, which in turn creates new waves that pushes back, this pushing back action, changes the electric wave's strength in one coordinate and thus, changing its overall direction, and because light must be perpendicular to it, it bends. You can test it out yourself by making the diagrams.

If you need further explanation, search the videos titled, "Why light slows down in water" and, "Why light bends in glass" by Fermilab, that's where I got my information from. I am not competent enough to figure out from this theory how 'reflection' works, maybe someone else in this post will be able to continue this brand new source.