0
$\begingroup$

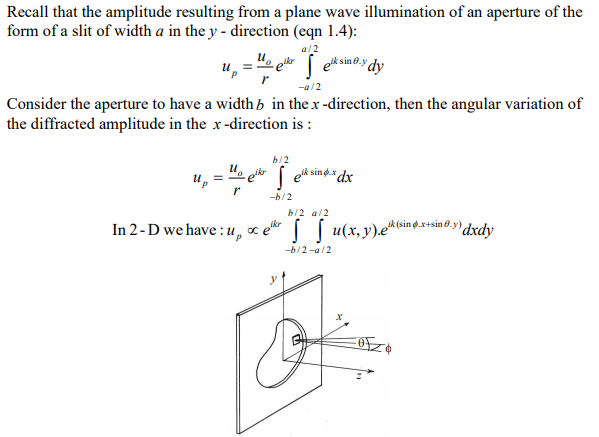
In this derivation, I can understand the formulas stated for 1D diffraction for both x andy (with the phase difference $\delta= ksin\theta y$) in the far-field limit, but how can we just sum the phases from both the x and y components in such a simple manner?

I would expect a formula like pythagoras would be needed instead of just $\delta = k(sin(\phi) x + sin(\theta)y)$.

enter image description here

Improve this question
$\endgroup$

1 Answer 1

Reset to default
0
$\begingroup$

You would need a more complicated formula if you were doing Fresnel diffraction, but here you are making the Fraunhofer approximation that all rays are parallel.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Even with this approximation, I'm not sure how the total path length difference can just be equal to the path length difference in the x direction + the path length difference in the y direction. Its obvious geometrically for the 1D case, but I've not seen a geometrical diagram to show it for 2D. $\endgroup$
    – Alex Gower
    Commented Aug 27, 2020 at 8:50
  • $\begingroup$ @AlexGower Did you ever figure this out? I've run into this exact problem as well, all the derivations I have seen are 1D and I understand perfectly well that it works in 1D, but when considering off-axis points in 2D my attempts show that the path length differences across the aperture start having terms of the form $\sqrt{x^2 +y^2}$ (coefficients omitted) which makes the subsequent integrals intractable. Am I missing something obvious or is this just being handwaved away? $\endgroup$
    – Thomas
    Commented Dec 26, 2022 at 3:50

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.