Deriving formula for effect of slit width and multiplicity for multi-slit diffraction pattern

Question

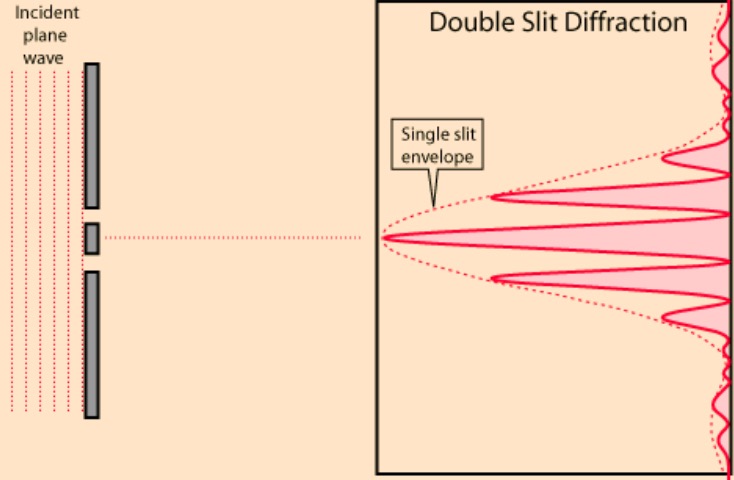
I have to derive formulas for the effect of slit width and slit multiplicity on a multi-slit diffraction diffraction pattern. The formulas I've been given are the typical ones,

$d\sin\theta=m\lambda$,

for the single slit pattern, where d is the slit width,

$D\sin\theta = n\lambda$

for the double slit pattern, where D is the separation of the slits, and

$L\tan\theta \approx L\sin\theta$

where L is the distance from the slit to the surface on which the pattern is being projected on.

I'm absolutely stuck on how to change these to give what I want. Would I solve for L and set the two equal to each other? Or would I draw out the slit and create a formula from the diagram?

Thank you for any help.

Answer 1

It might well be that you are being asked to give an indication of what the interference patterns look like.

Here is what the double slit pattern looks like.

You single slit formula gives the position of the maxima for the diffraction envelope which modulates the double slit pattern.

Note that you can have missing orders where in this example the first minimum of the diffraction pattern occurs at the same position as the third maximum of the double slit pattern.

For three slits with the same spacing between slits and the same slit width the pattern looks like this.

The things to note are that the diffraction envelope is still the same width as for the double slit, the separation of the principal maxima is the same as the double slit but the principal maxima are narrower.

What is not shown is the fact that the intensities for the three slit arrangement are greater than the double slit.

There is much more on the Internet with the HyperPhysics website a good one to start at.