I recently had seen an interesting experiment where a water bottle could be placed in a freezer for a while and would retain its liquid state until someone tapped on it, where it would quickly crystallize into ice. The explanations that I have seen for this experiment use terminology such as nucleation which I can't get an intuitive sense of. From what I learned from thermodynamics, when water is at zero degrees celsius, all energy taken from it goes into transformation before its temperature can decrease any further, as the molecules have to arrange themselves into a crystal lattice. I just need a very intuitive explanation of why transformation wouldn't always occur until a small amount of energy is applied through it with something like a tap on a water bottle.
-
4$\begingroup$ Have you read up on supercooling by the way if you haven't read that. $\endgroup$– TriatticusCommented Nov 16 at 23:59
-
3$\begingroup$ To clarify a deep confusion here: 0 degrees Celsius is NOT the point at which all energy is taken out of the molecules! They are still very far above absolute zero and still have quite a bit of energy. $\endgroup$– Matt HansonCommented Nov 17 at 1:32
-
2$\begingroup$ Oh @MattHanson I meant it as 0 degrees celsius makes energy taken for the fusion transformation before the temperature can decrease further. I might be wrong here, but I think that -273.15 degrees celsius is the limit, or at least for water. $\endgroup$– Alex AbramovCommented Nov 17 at 2:29
-
1$\begingroup$ You’re right in that the minimum temp is -273.15 degrees C, but the transformation still involves energy change! It just involves no temperature change because the state transformation has to take place first due to the principles of equilibrium thermodynamics. Also the minimum temperature is not substance dependent. $\endgroup$– Matt HansonCommented Nov 17 at 3:55
-
4$\begingroup$ @MattHanson I believe "all energy taken from it goes into transformation" means "all of the energy that is removed causes phase change [instead of temperature change]" not "all of the energy has been removed." There is no deep confusion here. $\endgroup$– phoogCommented Nov 17 at 8:54
3 Answers
For an (ice) crystal to form it needs to have a starting point to form around, that is called the Nucleation point. Liquids can be in a non stable state where they are supercooled but they can not make the transition to the lower state.
When you tap a water bottle you induce a small perturbation (disturbance) of the system and say move an air bubble to a more favorable spot so that nucleation can occur around it, or you move some impurity in a place so that ice starts to form around it. That is why the "tap" is needed, only to enable a nucleation site to come up.
Also from that Wiki page:
The most common crystallisation process on Earth is the formation of ice. Liquid water does not freeze at 0 °C unless there is ice already present; cooling significantly below 0 °C is required to nucleate ice and for the water to freeze. For example, small droplets of very pure water can remain liquid down to below -30 °C although ice is the stable state below 0 °C.
-
$\begingroup$ I've always found this explanation somewhat unsatisfying. How does merely tapping the container create nucleation sites? If there are air bubbles or impurities in the water, why aren't they sufficient to act as nucleation sites before the container is disturbed? What does it mean for a nucleation site to "come up" or to be in a "favorable spot"? $\endgroup$– DavidCommented Nov 18 at 0:35
-
$\begingroup$ @David air can be trapped in a microscopic crevice in the container wall where the water "can't get at it", but tapping provides enough energy to dislodge it and let it get surrounded by water, where nucleation can begin. $\endgroup$– hobbsCommented Nov 18 at 5:18
-
$\begingroup$ A sound wave in itself can be enough to start nucleation. $\endgroup$– StianCommented Nov 18 at 6:26
-
$\begingroup$ I wonder: There exist "warm packs" with some lcolored iquid inside and some "metal clicker" (I can't describe it better: You bend it and it makes a clicking sound). When you click, the liquid becomes "crystalline" and rather warm. Putting the thing into hot water reverts the process. So is that the same effect, but at a different scale of temperature? $\endgroup$– U. WindlCommented Nov 18 at 9:40
-
$\begingroup$ @U.Windl Hi, I get what you are trying to explain. It is called a Heating pad. That metal clicker is a "holder" of little crystals of some chemical like Sodium acetate (like salt eg.). When you "click" it, you shoot out one of the crystal molecules and than ice starts to form around it. The reason why it gets warmer when ice starts to form is the latent heat of solidification. That is the heat "produced" when a substance enters the lower configuration state. You can read more on this here: physics.stackexchange.com/questions/816668/… $\endgroup$– User198Commented Nov 18 at 18:09
This is the difference between energetics, and kinetics.
For H2O at (say) at -10 °C, the ice phase has a lower energy than the water phase. However, if you have water, and you don't have a low energy route to get to ice, then the conversion does not happen. Liquid water is in a locally stable configuration. Any small changes in bonding or relative position results in an increase in energy, so there is an activation energy 'hill' over which the system has to climb to reconfigure as ice.
This is exactly the same as having a lump of carbohydrate, like sugar for instance, in an oxygen atmosphere. Conversion to CO2 and H2O would be energetically favourable, but there isn't a low energy route to get there. It needs heating to many hundreds of degrees to provide the activation energy to break the existing bonds to allow new ones to form.
Instead of supplying the necessary activation energy to overcome the energy barrier to reaction, both reactions may be made to go by lowering the barrier.
In the case of water to ice, that's by providing a substrate, a nucleation centre, on which water molecules can start to rearrange themselves into an ice configuration by making bonds with the substrate.
In the case of oxidation of carbohydrate, living cells lower the energy barrier to the reaction with enzymes, catalysts, substrates and reaction chains that allow it to happen at body temperature.
-
1$\begingroup$ Wow this answer is wonderful. I get the intuition behind it, and I finally understand how the nucleation point works, where the water can have enough energy to effectively “activate” and turn into a crystalline, low energy form, which then makes the further water molecules not need additional activation energy to shift into a lattice shape. Thank you again! $\endgroup$ Commented Nov 17 at 18:39
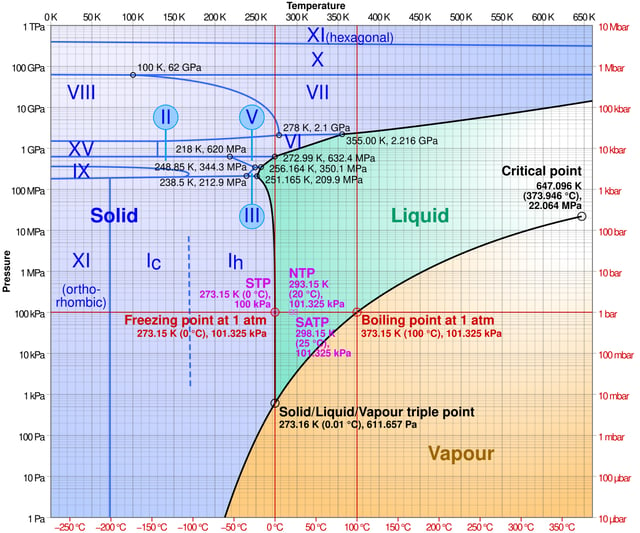
Besides the already given answer, and adding to your overall question, you might want to check out the concept of phase diagrams. These diagrams show you the phase of a particular substance under certain conditions of pressure and temperature. Water only freezes at zero degrees celsius in standard atmospheric pressure. At high pressures it starts to freeze at a slighter lower temperature, then it starts freezing at higher and higher temperatures. Eventually the pressure is so large that the molecules are effectively "squished", meaning you have ice even at really high temperatures. But at very low pressure the molecules essentially evaporate freely and you have a gas even at low temperatures.
Phase diagrams can be determined experimentally, but also through equations such as the Clausius-Clapeyron equation for the liquid and vapor phases (liquidus-vaporus).
-
1$\begingroup$ Thank you for the response! I understand the explanation, but I don't get why the transformation temperature would stay the same for pressures of 10 MPa to 1 kPa. Why does the temperature of freezing barely change for these pressures, but dramatically change for temperatures above 1GPa? I saw the equations, but is there a simple explanation for this effect? $\endgroup$ Commented Nov 17 at 2:00
-
1$\begingroup$ @AlexAbramov it does not stay absolutely the same, but the change is small because the density change is small between liquid and solid. One can zoom the phase diagram at different scales and find different interesting stuff. $\endgroup$– fraxinusCommented Nov 17 at 9:08
-
1$\begingroup$ @AlexAbramov, The regions of the diagram that are labelled with different roman numerals correspond to different crystal structures —different allotropes of solid water. I don't fully understand the shape of the boundaries, but I'm guessing that the different densities of the various allotropes and phases play some role. $\endgroup$ Commented Nov 17 at 13:05