1
$\begingroup$

I'm here to ask a really stupid question just to be sure of its answer. My professor gave us an exercise where we have to determine the Lagrangian of a system that is formed by a circular ring of mass $M$ and radius $R$ which is placed in the $(x,z)$ plane. In this plane, it rotates around one of his point $O$ fixed in the origin of the axes. Also, there is a particle $P$ of mass $m$ that moves on this ring-like object without friction.

The Lagrangian has to be written in terms of the generalized coordinates $\theta$ and $\phi$, where $\theta$ is the angle formed by the segment that connects $O$ with the center $C$ of the ring guide with the $z$ axes and $\phi$ is the angle formed by the segment that connects the position of the particle $P$ with the center of the ring guide $C$ and the $z$ axes.

I have no problem in understanding how to write the Lagrangian itself, but I'm more confused on how the professor wrote the solution for this problem. They stated that the coordinates of the center $C$ of the guide can be written as: $$ \begin{cases} x= R\sin \theta\\ z=-R \cos\theta \end{cases} $$ where I would have written since $z = R \sin\left(\frac{\pi}{2}-\theta\right)=R \cos\theta$.

EDIT: I'll add an image of the problem so that it is easier to visualize the system. This is not official, it is how I understood it. 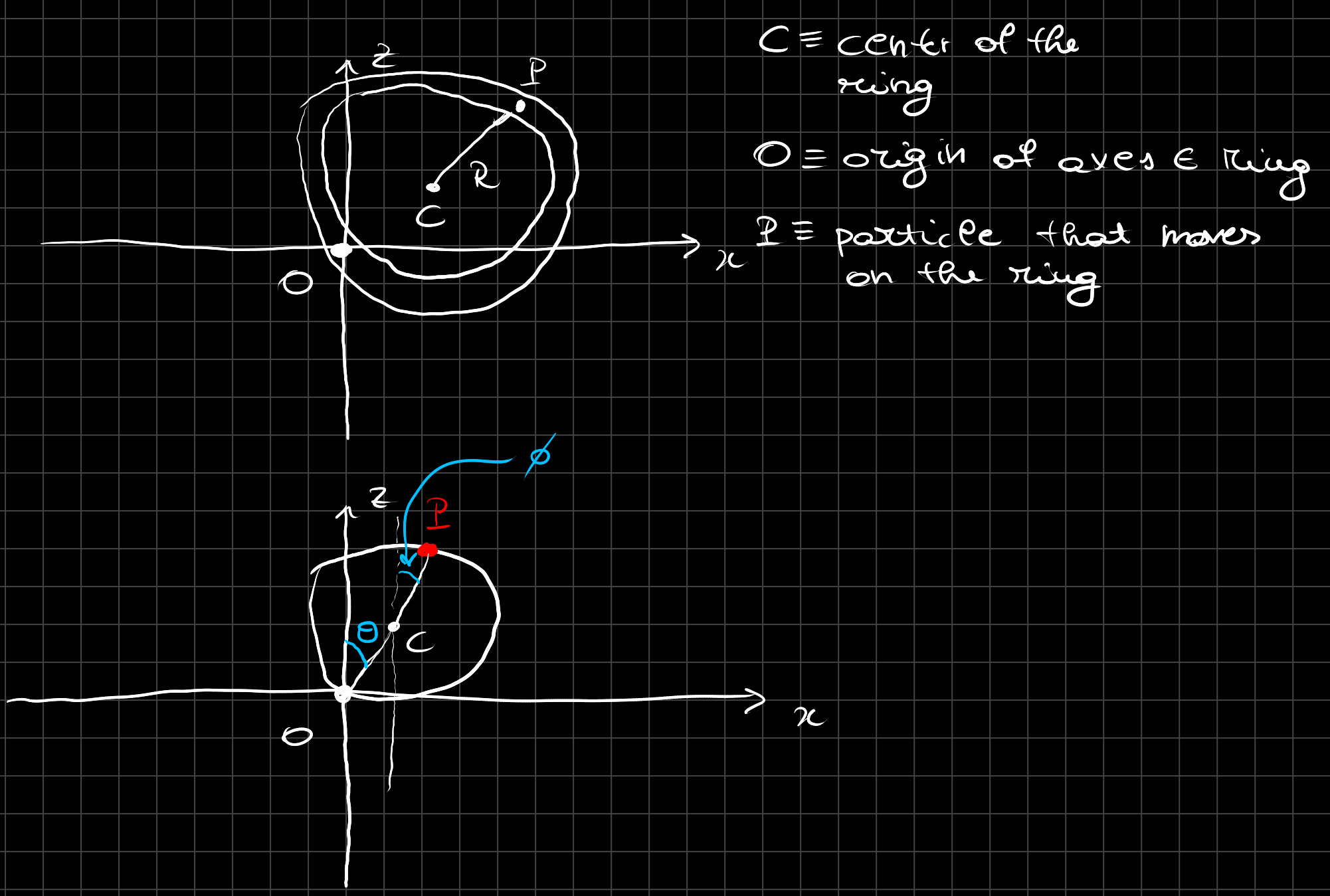enter image description here Keep in mind that the ring guide can rotate around the origin $O$, so $\theta$ is not fixed.

Improve this question
$\endgroup$
6
  • $\begingroup$ Do you have a picture of your problem; for me its hard to understand. $\endgroup$
    – trula
    Commented Jul 29 at 16:44
  • $\begingroup$ @trula I updated the question with the image. It was hard even for me to visualize, they definitely should have added an image of the system. $\endgroup$
    – deomanu01
    Commented Jul 29 at 20:22
  • $\begingroup$ Both possibilities are ok, you start with $\Theta=0$ on z=R with C on the positive z axes , your professor with z=-R but the circle will roll if you change $\Theta$ so it does not matter for the problem . $\endgroup$
    – trula
    Commented Jul 30 at 11:22
  • $\begingroup$ So he basically oriented the z axes downwards with respect to what I did? $\endgroup$
    – deomanu01
    Commented Jul 30 at 11:33
  • $\begingroup$ Yes, thas an other viepoint for the same situation, i prefer mine. $\endgroup$
    – trula
    Commented Jul 30 at 13:34

0

Reset to default