Movement of inclined plane with its slope changing over time

Question

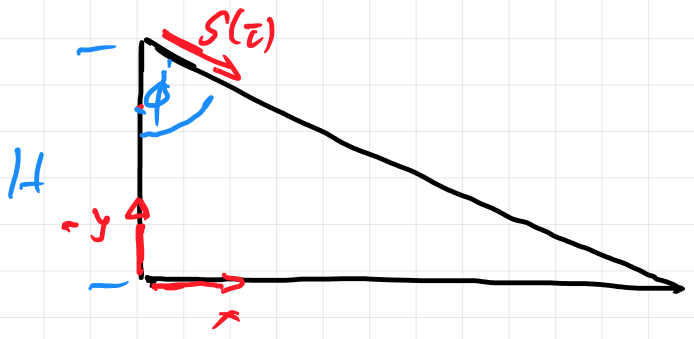
Imagine a situation of a mass over the center of inclined planed which its slope in changing over time. The angle changes acording to the movement of forced damped harmonic oscillator. I want to know the position of the particle respect the center, so i use polar coordinates $x=r\cos\phi$ and $y=r\sin\phi$ when $\phi$ is the solution of forced damped harmonic oscillator $a\ddot\phi+b\dot\phi+c\phi=F\sin\omega t$ that gives me the angle of the slope.

The lagrangian is given by:

$L=\frac{1}{2}m(\dot r^2+r^2\dot\phi^2)-mgr\sin\phi$

On the other hand, I want to consider the dissipative force between the mass and the plane so the Rayleigh dissipation function is $R=-\mu Nv=-\mu mg\cos\phi \sqrt{\dot r^2+r^2\dot\phi^2}$

Then, the equation of motion is given by:

$\frac{d}{dt}\frac{\partial L}{\partial \dot r}-\frac{\partial L}{\partial r}+\frac{\partial R}{\partial \dot r}=0$

The angle $\phi$ is a constraint given by the damped equation and i know its value every time.

Finally the motion is given by:

$\ddot r-\mu g\cos\phi\frac{\dot r}{\sqrt{\dot r^2+r^2\dot\phi^2}}-\dot\phi^2r+g\sin\phi=0$

My question is if the approach I have done of the problem is correct.

Answer 1

start with the position vector to the mass

$$\mathbf R=\left[ \begin {array}{c} s\sin \left( \phi \left( \tau \right) \right) \\ H-s\cos \left( \phi \left( \tau \right) \right) \end {array} \right] $$

from here the velocity is:

$$\mathbf v=\left[ \begin {array}{c} \sin \left( \phi \left( \tau \right) \right) {\dot s}+s\cos \left( \phi \left( \tau \right) \right) { \frac {d}{d\tau}}\phi \left( \tau \right) \\ -\cos \left( \phi \left( \tau \right) \right) {\dot s}+s\sin \left( \phi \left( \tau \right) \right) {\frac {d}{d\tau}}\phi \left( \tau \right) \end {array} \right] $$

hence the kinetic energy

$$T=\frac m2\,\mathbf{v}\cdot\mathbf{v}$$

the potential energy

$$U=m\,g\,\mathbf R_y-F_\mu\,s$$ where $~F_\mu=\mu\,N~$ is the friction force

and with EL you obtain the EOM

$$m\,\frac{d^2\,s}{d\tau^2}-m \left( {\frac {d}{d\tau}}\phi \left( \tau \right) \right) ^{2}\,s(\tau)-m\,g\cos \left( \phi \left( \tau \right) \right) +\mu\,N =0\\ N=m\,g\sin(\phi(\tau))$$

the function $~\phi(\tau)~$ is the solution of damped harmonic oscillator.