Optimal selection of generalized coordinates in Lagrangian system

Question

EDITED: The number of bonds is actually 2, not 1 (look at edit history). Fixed for archiving purposes.

Problem: The edge A of an homogeneous rod (of length $\ell$ and mass $m$) is performing a smooth circular motion around the center $O$ with a constant angular speed $\omega$. The motion takes place on the $Oxy$ plane without friction under the action of an attractive force $\vec{F}=-k \vec{r}_k$ (k>0), where $\vec{r}_k$ is the vector position of the center of mass. Note: In the original image from my textbook, it appears as if $F_k$ acts towards $(x,y)=(0,1)$ and not towards the origin $(0,0)$. There is no gravity.

Goal: To calculate the Lagrangian of the system.

Attempted solution: The rod is a solid body moving on a 2-dimensional plane. Therefore there are $3n-k$ degrees of freedom, where $n$ is the number of the bodies (here $n=1$) and $k$ is the number of bonds (here $k=2$), since the edge A is confined to move along the circle and the angular speed is constant. Subsequently, one needs 1 generalized coordinate to describe the system.

$\phi$ is angle between $(OA)$ and $Ox$ for which $|\dot{\phi}|=|\omega|$, but what would the generalized coordinate be ?

---

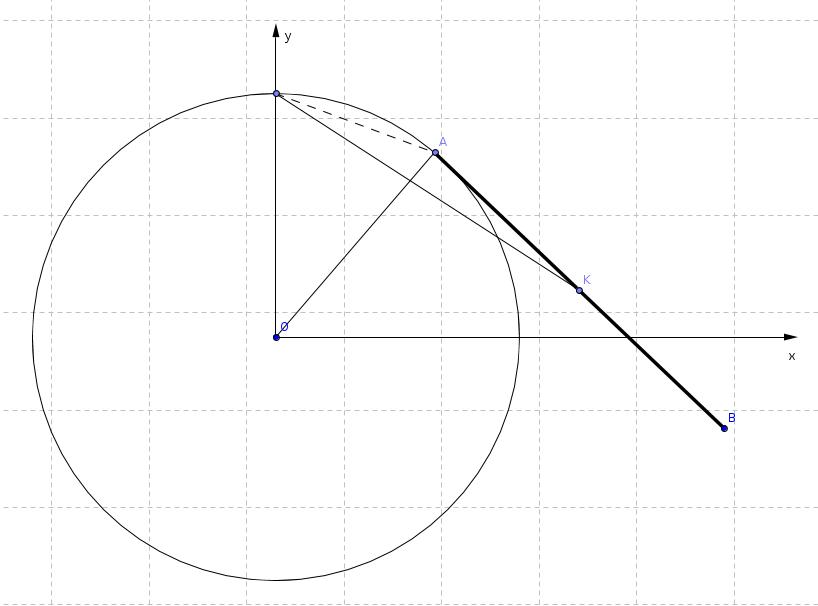
Based on the answer from @pppqqq, here is an updated diagram of the problem:

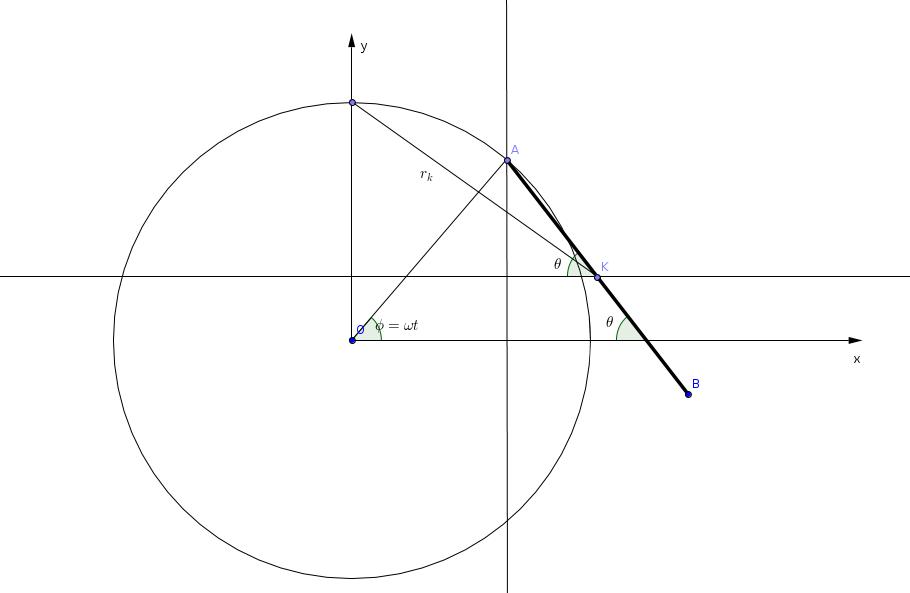
Pending issues: (These have been answered by @pppqqq)

1. Are we assuming that the length of the rod is $\ell'=2\ell$ ? If not, shouldn't it be $x_{CM} = R\cos\phi + (\ell/2) \cos\theta$ ?
2. Shouldn't $y_{CM}$ be $y_{CM} = R sin\phi−\ell\sin\theta$ (assuming that the length of the rod is $2\ell$)?

Answer 1

The numbers of deegres of freedom for a planar body in the plane is $$\text{2 (coordinates of CM)}+1\text {(angles to determine the body orientation)}=3.$$

As you correctly recognize, the constraint is one: $$|\vec {OA}|=R,$$ so you need two numbers $\varphi, \theta$ to localize the body in the plane.

So, you have the first, $\varphi$, and I gave you a big hint by calling also the second with an angle-like name. The second number could be... ?

---

The second number could be the angle $\theta$ that the rod makes with an axis, say the $x$ axis.

With this the coordinates of the center of mass are (if $R$ is the radius of the circle, $2\ell$ the lenght of the rod) $$x_{\text {CM}}=R\cos \varphi +\ell \cos \theta,\qquad y_{\text {CM}}=R\sin \varphi +\ell \sin \theta .$$ The next step is to calculate the kinetic energy $T$ of the body. This comes in two parts (Koenig's theorem): $$T=T_{\text {CM}}+T_{\text {rotational}}.$$ The second one is simply: $$T_{\text {rotation}}=\frac {1}{2}I\dot \theta ^2=\frac{m\ell ^2}{6}\dot \theta ^2,$$ (the moment of inertia of a rigid rod about the CM is the variance of the uniform distribution, multiplied by $m$, $\frac{m L^2}{12}$). The other term is $$T_{\text {CM}}=m \frac {\dot x ^2+\dot y ^2}{2}=\frac{m}{2}(R^2 \dot \varphi ^2+\ell ^2 \dot \theta ^2+2R\ell\cos (\theta -\varphi)\dot \theta \dot \varphi )$$ (check the cosine term). I leave to you the potential energy; just a thing: to simplify the outcome, you can note that here you have

1. A characteristic lenght, that you can choose as $R$ or $\ell$.
2. A characteristic mass

Since the equations of motion are unaltered by a scaling of the lagrangian, you can divide the whole lagrangian by $mR^2$ or $m\ell ^2$, or simply, which is equivalent, set $m=1$, $R=1$. This will simplify your equations and make your life easier.