0
$\begingroup$

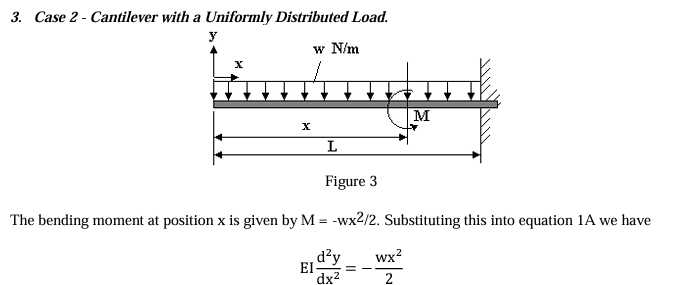
I am trying to understand how to calculate the bending moment for a cantilever with a uniformly distributed load so that I can build an equation of moments, as shown in this example:

enter image description here

I tried calculating the moment but didn't arrive at their expression. I tried the following: $$M = -\int_0^x wl*dl + \int_0^{L-x} (L-x-l)w*dl $$ where L is the length of the beam, x is the position (for which I am calculating the moment) with respect to the end of the beam, and w is the weight per unit length. I have added the right integral to represent the moments from the forces applied to the right of position x, but from the example above it would seem that only the left integral contributes to the moment: $$M = -\int_0^x wl*dl = -wx^2/2$$ why am I supposed to disregard the moments from the right?

Improve this question
$\endgroup$
1
  • $\begingroup$ Voting to reopen. Clearly a conceptual question about calculation of moments, not a "do my homework" question. $\endgroup$
    – gandalf61
    Commented Mar 1 at 10:13

1 Answer 1

Reset to default
0
$\begingroup$

You have a static situation and the definition of bending moment is the torque that tries to bend the cantilever at the point $x$. Now you can use that there must be opposite torque on the parts of the cantilever that are left and right of the point $x$, to keep the situation static.

The part on the right is difficult to compute because it interacts with the wall at the fixation point and also will feel gravity. The part on the left is fortunately more easy, there's only gravity. You calculate it with only the first term in your expression. This does not mean that the right-hand torque is neglected, but you just happen to know it already: it must be opposite to the left-hand result. (It is of course needed to keep the system in equilibrium.)

Improve this answer
$\endgroup$
1
  • $\begingroup$ I will clarify my question. My understanding was that Euler–Bernoulli beam theory gives me the moment from microscopic considerations, and now I must equate that to the moment from macroscopic considerations. Why should that not include moments from the right side of the beam? In other words, my misunderstanding stems from Euler–Bernoulli beam theory: what moment does this theory give us and what moment should we equate to it subsequently? $\endgroup$
    – eheshing
    Commented Mar 3 at 16:33

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.