0
$\begingroup$

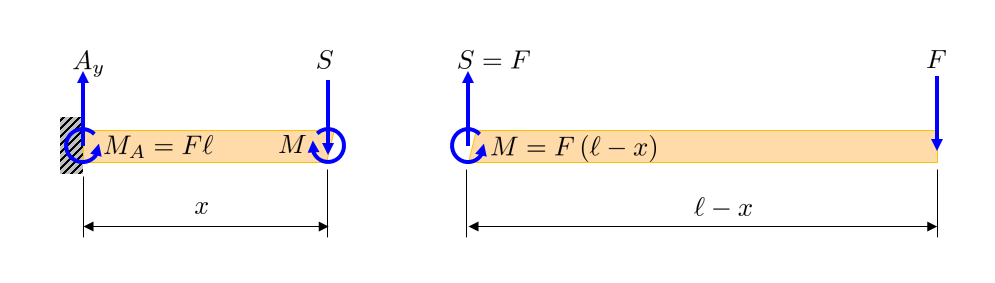
If I have a cantilever beam of length L fixed at the left end to a wall and I hang a weight W from it's right free end then why should the bending moment at a point x units to right of the wall be W(L-x)?

If I understand correctly, the bending moment at a point on the beam should be the total torque of the forces acting on cross surface at that point about an axis passing through the geometric center and perpendicular to the plane of bending, then how is this equal to W(L-x)?

Improve this question
$\endgroup$

3 Answers 3

Reset to default
3
$\begingroup$

If you split the beam in two at the position $x$ and do Free Body Diagrams you will understand why the internal moment is such.

FBD

Each split body needs to be in balance. To balance the part of the beam between the split and the end where the load is applied a moment of $F \left( \ell -x \right)$ is needed.

Improve this answer
$\endgroup$
2
  • $\begingroup$ If the force causes a torque about the point x, then what is the force at the point x that is causing this bending moment and is the torque of this force calculated about the free end at L $\endgroup$
    – Metric
    Commented Apr 18, 2019 at 14:53
  • $\begingroup$ The force $S$ is called shear force and it causes (shear) stress and deflections in beams, but of much less magnitude as the effect of internal bending moment (unless the beam is really short in length). $\endgroup$
    – John Alexiou
    Commented Apr 18, 2019 at 14:56
2
$\begingroup$

If you focus on the section of the beam between x and L, to hold that section in equilibrium, you need an internal upward shear force of W and an internal counterclockwise bending moment of (L-x)W applied to the cross section at x.

Improve this answer
$\endgroup$
6
  • $\begingroup$ Is the internal counter clockwise moment evaluated about the free end at L. I don't see how the bending moment ( which is the torque of the forces on a cross section about the neutral line) is related to this $\endgroup$
    – Metric
    Commented Apr 18, 2019 at 14:55
  • $\begingroup$ the internal counter clockwise moment is not evaluated about the free end at L. The internal counter clockwise bending moment at x balances the moment of the applied force W at x = L. In my judgment, @ja72's diagram on the right shows this very strikingly. $\endgroup$
    – Chet Miller
    Commented Apr 18, 2019 at 15:21
  • $\begingroup$ I know it's supposed to be a simple, but I can't seem to understand it. Can you tell me a bit more about bending moment as in how it is formed and how it can counter the torque due to the external force. Is bending moment a torque? Then about which point is it acting? $\endgroup$
    – Metric
    Commented Apr 18, 2019 at 15:56
  • $\begingroup$ The top half of the beam cross section at x is in axial tension (tensile stress), and the bottom half of the cross section is in compression. This translates into a bending moment about the neutral axis. $\endgroup$
    – Chet Miller
    Commented Apr 18, 2019 at 16:14
  • $\begingroup$ I think I understand it now. The torque due to the external force about the point on the neutral surface at x is balanced by torque of the forces above and below the neutral surface about that point on the neutral surface which basically is just the moment of bend. Am I right? $\endgroup$
    – Metric
    Commented Apr 18, 2019 at 18:55
1
$\begingroup$

To summarize what others have said, to be in static equilibrium the bending moment in the beam will be a maximum at the support and zero at the point of application of the load, linearly decreasing from the support to the load so that $M=W(L-x)$. This is because the moment due to the load that needs to be counteracted by the bending moment of the beam at any cross section is the product of the load and the length of the moment arm to the section. The length of the moment arm to each section of the beam is $L-x$.

Hope this helps.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.