1
$\begingroup$

Is there a rough formula for the fraction of galaxies in the observable universe with masses between M and M + dM? Or perhaps a graph that displays the same information? I've looked online but can't find anything except mass distribution within a given galaxy.

Thanks for any help

Improve this question
$\endgroup$
2
  • $\begingroup$ The concept you're looking for is the "mass function", e.g. the stellar mass function (for the distribution of galaxies by their mass in stars), or the baryonic mass function (for the distribution by mass in baryons), or the halo/subhalo mass function (for dark matter). $\endgroup$
    – Sten
    Commented Sep 23, 2023 at 17:22
  • $\begingroup$ Thank you! I think I was using the wrong terminology in my searches so I didn't get any valid results $\endgroup$
    – Thanos
    Commented Sep 23, 2023 at 17:41

1 Answer 1

Reset to default
0
$\begingroup$

The concept you're looking for is the galaxy mass function. I'll just point out how to interpret one example. This is a "stellar mass function", so it's showing the distribution of galaxies by how much mass they have in stars only. It comes from a simulation paper; the curves show simulation data while the points show observational data.

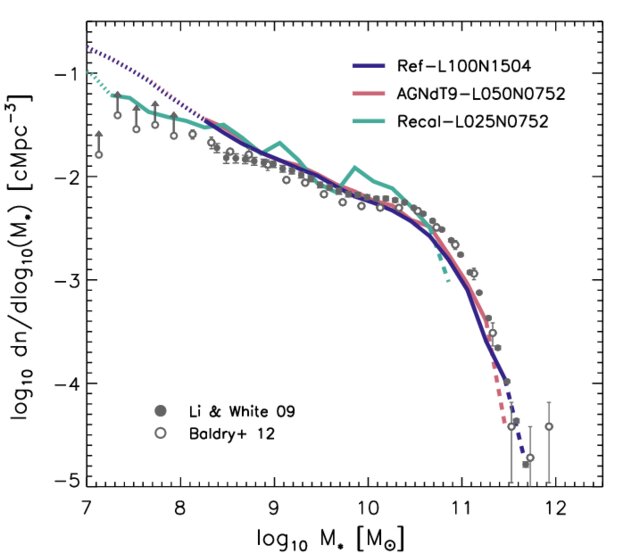
stellar mass function

$M_*$ refers to a galaxy's mass in stars ("stellar mass"), given in units of $\mathrm{M}_\odot$ (mass of the sun). $n$ refers to the number density of galaxies, i.e. the number of galaxies per spatial volume. It is given in inverse cubic comoving megaparsec ($\mathrm{cMpc}^{-3}$). The quantity $$\mathrm{d}n/\mathrm{d}\log_{10}M_*$$ is then the differential number density of galaxies, per logarithmic interval in stellar mass. For example, since $\mathrm{d}n/\mathrm{d}\log_{10}M_*\simeq 10^{-2.2}$ at $M_*\simeq 10^{9.5}~\mathrm{M}_\odot$, that means there are about $10^{-2.2}$ galaxies per $\mathrm{cMpc}^{3}$ that have stellar masses in the range $10^{9}~\mathrm{M}_\odot$ to $10^{10}~\mathrm{M}_\odot$.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Okay thank you that is really helpful. I was slightly confused by the meaning of the unit dex^(-1) which appeared in the majority of graphs, I assume that is equivalent to taking the differential with respect to log base 10 of the independent variable? $\endgroup$
    – Thanos
    Commented Sep 24, 2023 at 18:20
  • $\begingroup$ @Thanos That just means it's $\mathrm{d}n/\mathrm{d}\log_{10}M$, i.e. the differential number density per decade (factor of 10) in mass. It's a dimensionless unit so is not needed as long as the meaning is clear. $\endgroup$
    – Sten
    Commented Sep 24, 2023 at 18:46

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.