Why rainbow colors are as they are? [duplicate]

Question

There are handful of questions related to rainbow formation here already, but after looking into them, I feel that I must ask yet another one.

I did a little research on the subject, and most of the articles or youtube videos tend to focus on refraction-reflection-refraction in the single droplet part. The big picture, for some reason is expected to be self-evident after all that geometry and calculus put into finding maximum reflection angles for different wavelengths. Others will show a picture with two droplets and two rays of red and blue colors, where one ray of each color is missing observer's eye and have that as sufficient evidence. However, it's certainly not obvious moment for me.

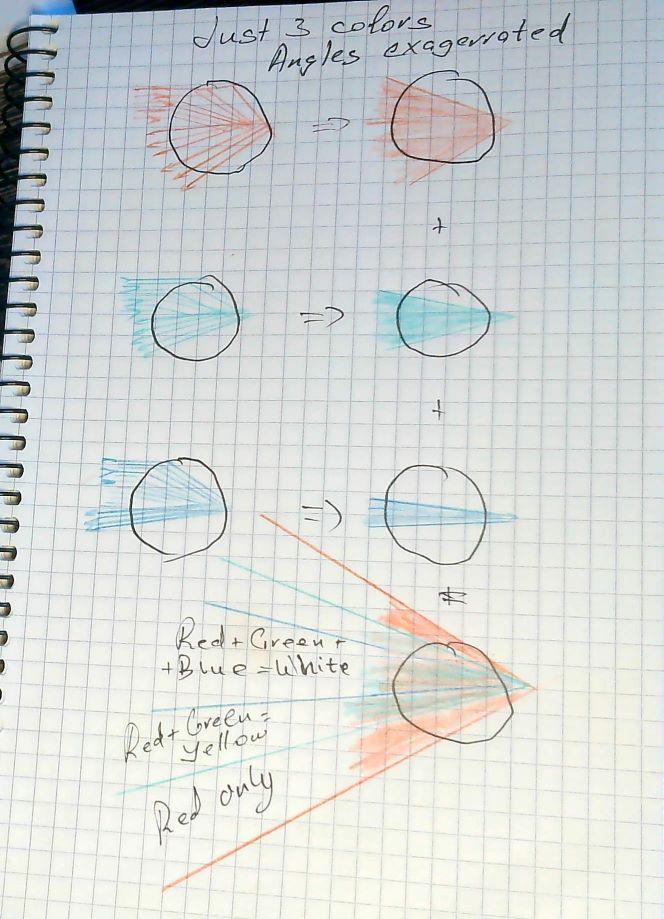
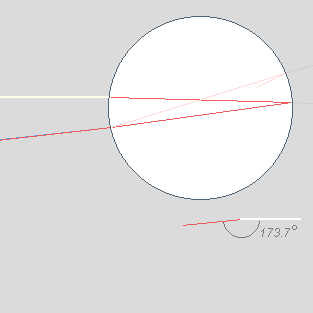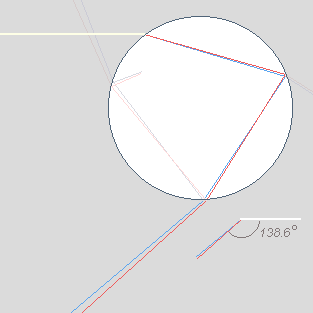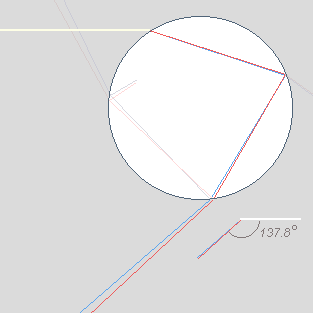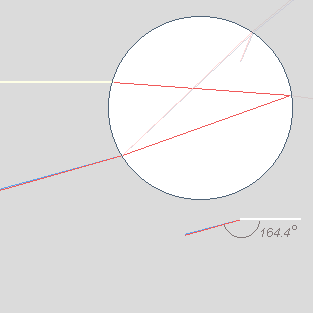
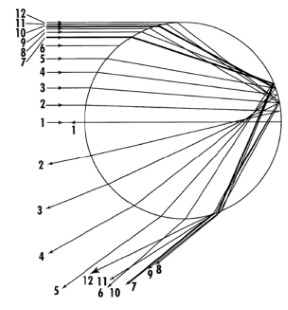
So if I get it right, abstracting away all the calculus-geometry stuff, if we take beam of parallel single wavelength rays hitting a perfect spherical droplet, it is going to be reflected in the shape of cone with the apex at the droplet, where half-angle depends on the wavelength (I like the image used for illustration in this question)

Taking into account that white solar light is actually composition of spectrum, we can replace every droplet of water by a tiny light which will emit white light inside the cone with 40° half-angle, then on the edge of that cone we'll start losing violet, then blue, then green and so on up to 42° where we'll have only red left.

Now from the observer's point of view, those droplets that are closer than 40° from antisolar point are going to reflect white light, and that matches with my observation: area inside rainbow is slightly brighter than outside.

Yet as we get closer to 40°, the magic called "the rainbow" starts, and that's where my understanding gets in conflict with reality:

Droplets at 40° reflect towards the observer all the light except violet. Respectively, I would expect to see inner rainbow ring as white except violet, i.e. bright green. Then with reduction of blue and then green it should have gotten more and more yellow. To my best understanding we should see kind of "cumulative spectrum" (in lack of better words I just coined this term), with no light outside the 42°(check), starting with red (check again), through the yellow to green at 40°, but without blue and violet (wrong), with just white inside the 40° ring (check again):

Red => Red
Orange => Red+Orange = Red
Yellow => Red+Orange+Yellow = Orange
Green => Red+Orange+Yellow+Green = Yellow
Blue => Red+Orange+Yellow+Green+Blue = Green
Violet => Red+Orange+Yellow+Green+Blue+Violet = White

As apparently we are able to see blue and weak, but clear violet stripe in the rainbow, my understanding is wrong. So why don't we get red, yellow, green and blue light in it as well? Why do we see spectrum as if we were using prism, not the "cumulative spectrum"?

Answer 1

With the addition of some good diagrams I think I now understand your question.

This diagram does not show a key feature of the reflections.

The intensity of the reflected rays varies and so you do not observe a uniform cone of reflected light.

Here is a gif animation to show you what I mean.

[Individual images were taken from an Atmospheric Optics webpage and combined to produce a gif file.]

Parallel rays are coming in at the top of a water droplet and refracted, reflected and refracted again to emerge from the bottom half of the drop.

What you should note is that for a given range of impact parameters of the incident rays the highest concentration of emergent rays occurs occurs around an angle of $137.5^\circ$, ie that is where the emergent light is brightest and light from around that angle swamps the light from that emerging at other angles. So you diagram should show a high intensity of red light around a particular direction and and much lower elsewhere.

Here is a ray diagram to illustrate the "bunching" of light rays along a particular direction.