0
$\begingroup$

There are YouTube videos where a person hold a cup of $\rm CO_2$ and pour it on top of burning candles to distinguish them. As far as I know, $\rm CO_2$ and $\rm O_2$ both are almost perfect ideal gas at atmospheric conditions, so the volume they occupy shouldn't affect each other. Their joint probability distribution should just be the two Boltzmann distributions in product, and should be mostly uncorrelated. If so, how can $\rm CO_2$ displace $\rm O_2$ and distinguish fire?

While a cup of CO2 has partial pressure much higher than CO2 partial pressure in the atmosphere, I feel it should still be nearly ideal since N2 is nearly ideal even though the atmosphere is almost all N2 (same order of magnitude)

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ Ideal gas density is directly proportional to molar mass. $\endgroup$
    – David White
    Commented Feb 15, 2023 at 23:49
  • $\begingroup$ @DavidWhite for a single species, I agree. For two species, I don't know what's the conventional definition of molar mass. Also, I don't see how that's relevant, no offense. $\endgroup$
    – Bohan Xu
    Commented Feb 15, 2023 at 23:55
  • 1
    $\begingroup$ You only have one molecular species in the cup. $\endgroup$
    – David White
    Commented Feb 15, 2023 at 23:58
  • $\begingroup$ @DavidWhite oh, I know CO2 is denser. I agree the Boltzmann distributions of CO2 is more affected by gravity, but it still should be uncorrelated with the Boltzmann distribution of O2, since both are ideal and the "repulsive volume", so to speak, they occupy should be negligibly small. $\endgroup$
    – Bohan Xu
    Commented Feb 16, 2023 at 0:03

4 Answers 4

Reset to default
4
$\begingroup$

I think your problem may be in your statement Their joint probability distribution should just be the two Boltzmann distributions in product. That may be true once the O2/N2 mixture that makes up the atmoshere and the CO2 volume have fully mixed, but until that occurs the unmixed gases have significantly different densities (as pointed out by @Thomas Fritsch), so the denser CO2 will displace the air and put out the candle.

Diffusive mixing of the two gas volumes will be a fairy slow process (diffusion coefficient of CO2 in air is 16 mm2/s) so the time for that to occur is long compared to the time for the gas displacement to take place.

The ideal gas laws do not take diffusion into account, so are not a suitable basis to use in analysing the problem.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thanks for the answer. It's interesting that the trajectory of the particles will make a "U-turn", so to speak. The heavier pocket first all goes down together to the very bottom (given the time needed is less than the time scale of diffusion), but then diffuses, and the probability distribution will then shift back up. If there is only one heavy particle released from near the bottom, its distribution will net shift up directly. It's just pretty interesting and counter-intuitive to me. I guess more is indeed different~ $\endgroup$
    – Bohan Xu
    Commented Feb 17, 2023 at 19:01
4
$\begingroup$

As far as I know, CO$_2$ and O$_2$ both are almost perfect ideal gas at atmospheric conditions, so the volume they occupy shouldn't affect each other.

Room temperature CO$_2$ and O$_2$ can often be treated as ideal gases, but this is not one of those times. The issue is collisions. The mean free path of an air molecule (e.g. O$_2$) at STP is about 60 nm - an incredibly small distance. If you divided a container in half using a partition and filled one side with O$_2$ and the other side with CO$_2$, it would take an extremely long time (apparently on the order of 10 minutes) for the gases to mix completely once the partition was removed.

As a result, your CO$_2$ will tend to move as a fairly cohesive mass of fluid on the scale of seconds, which is the time scale you're studying when you dump it out onto a candle. This is also why we talk about air parcels and cold fronts in meteorology - distinct "packets" of air undergoing bulk motion tend to move around each other rather than mix together, because the time required for the latter is far longer than the time required for the former.

So really, the remarkable thing is not that ideal gas approximation fails, but rather that it so often succeeds. The key insight is that collisions cause a gas to relax toward equilibrium. Once that equilibrium is achieved, collisions no longer alter the macroscopic properties of the gas, and so as long as there are no appreciable long-range interactions between gas particles, the ideal gas approximation tends to yield the correct results. As soon as the gas is no longer in equilibrium - say, when the CO$_2$ is poured out of its container - the approximation fails badly until collisions have caused the system to equilibriate again.

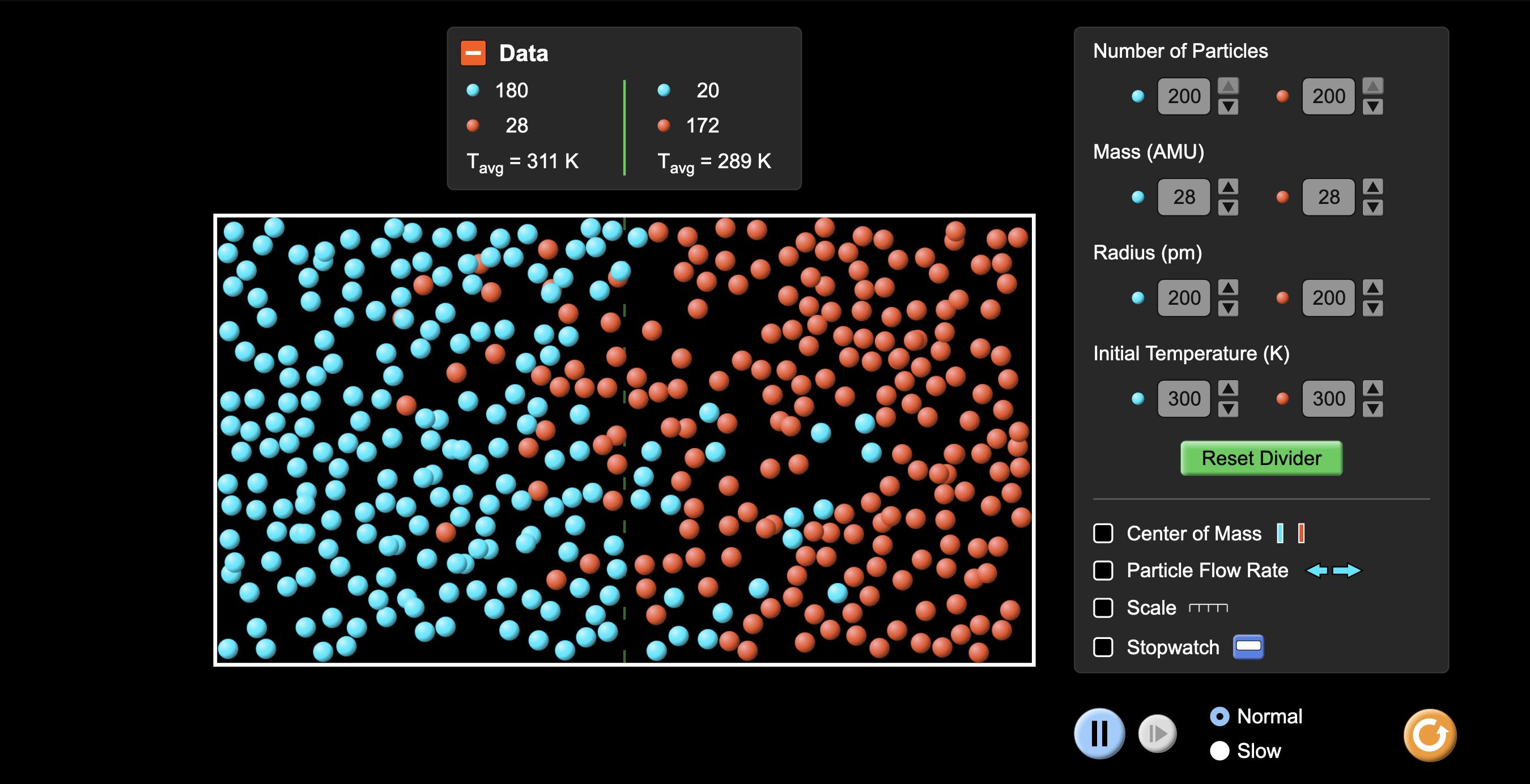
This excellent simulation from PhET illustrates precisely this phenomenon, so it's worth a look.

enter image description here

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thanks for the answer. $\endgroup$
    – Bohan Xu
    Commented Feb 17, 2023 at 19:01
2
$\begingroup$

The important quantity is not pressure, but density. $CO_2$ is heavier than air. More exactly (from Carbon dioxide - Physical properties):

At standard temperature and pressure, the density of carbon dioxide is around $1.98\text{ kg/m}^3$, about $1.53$ times that of air.

That's why carbon dioxide sinks down in air, as long as the gases have not mixed yet.

Improve this answer
$\endgroup$
2
  • $\begingroup$ I agree the Boltzmann distributions of CO2 has a bigger effect from gravity, but it still should be uncorrelated with the Boltzmann distribution of O2, since both are ideal and the "repulsive volume", so to speak, they occupy should be negligibly small. $\endgroup$
    – Bohan Xu
    Commented Feb 15, 2023 at 23:59
  • 1
    $\begingroup$ (+1) And the Lake Nyos disaster provides a tragic corroboration: en.wikipedia.org/wiki/Lake_Nyos_disaster. $\endgroup$
    – Ed V
    Commented Feb 16, 2023 at 0:48
2
$\begingroup$

Analogous phenomenon: If I drop a sugar cube in a vat of water, it sinks to the bottom and sits for a while, even though the solubility of sugar in water is high—certainly enough for complete dissolution.

The key idea is that any cup of carbon dioxide in air has been specially prepared to be in a far-from-equilibrium condition, one that immediately starts evolving toward equilibrium.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.