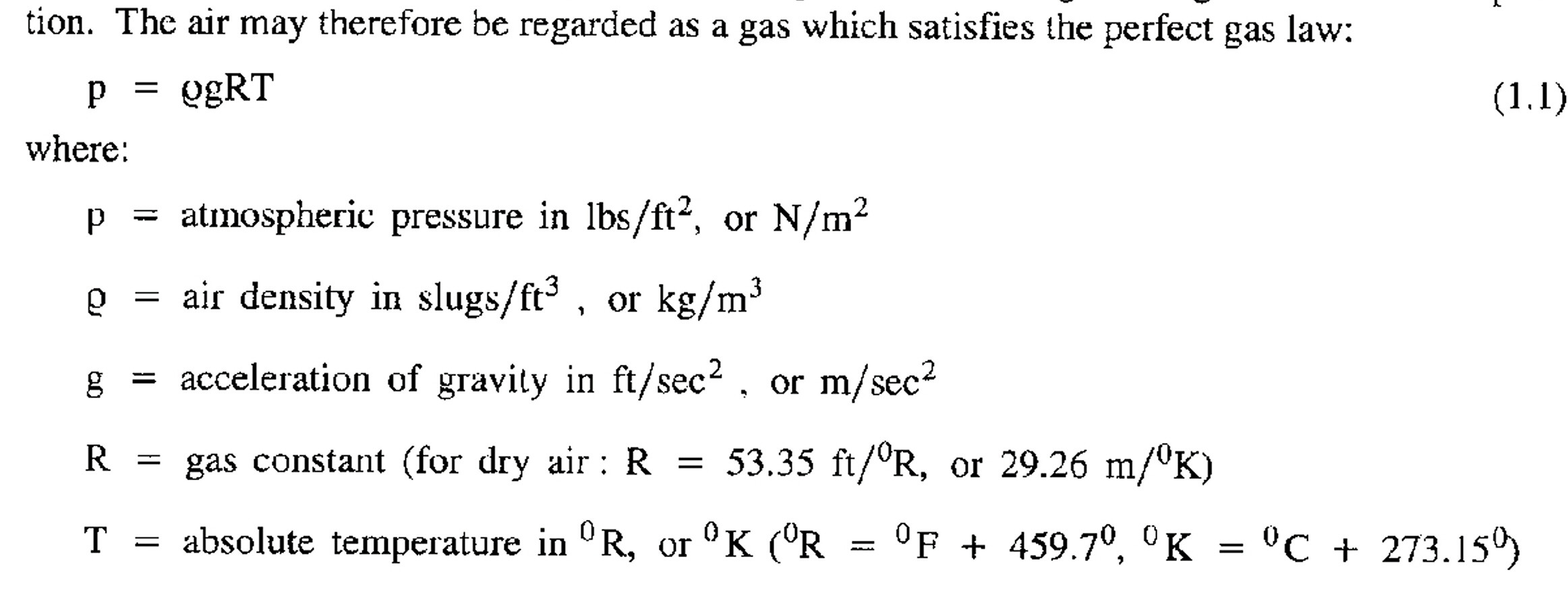For ideal gases we studied p=ρRT . But why sometimes we can find p=ρgRT? (for an instance Ref: Dr. Jan Roskam "Airplane Aerodynamics and Performance" Pargraph "1.1 ATMOSPHERIC FUNDAMENTALS" equation (1.1) )
1 Answer
When we write the ideal gas equation of state we normally write is as:
$$ PV = nRT $$
or with a quick rearrangement:
$$ P = \frac{n}{V}RT $$
where $n$ is the number of moles and $R$ is the gas constant in joules per kelvin - $R=8.314$ J/K. The quantity $n/V$ is the molar density i.e. the number of moles per cubic metre. Roskam has written the equation using different units. If I expand his density to be mass divided by volume then his equation reads:
$$ P = \frac{mg}{V}RT $$
where $m$ is the mass in kilograms not the number of moles, and he also writes the gas constant in units of metres per kelvin - $R=29.26$ m/K. The quantity $mg$ used in Roskam's version of the equation differs from the number of moles, $n$, used in the physicists equation by a factor of the weight of one mole of air. Roskam has absorbed this difference into his value for the gas constant.
It's not unusual for engineers to use units that differ from those of physicists. An engineer probably neither knows nor cares what a mole of air is ($0.0288$ kg or $0.283$ N at ground level for anyone who's interested) and is far more interested in what they can measure directly i.e. the weight of the air in kilograms.