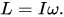A stone is attached to a string and is revolved in a circular path with constant angular velocity $\Omega$. In this state its angular momentum is $L$. If the length of the string is reduced to half and again it is revolved with the same angular velocity $\Omega$ then find angular momentum?
Why we cannot use Conservation of angular momentum here Bcoz no external torque acts on it.
Conservation of angular momentum is a very fundamental conservative law. It says that If no torque acts on the body the angular momentum is conserved. In your case Angular momentum will not be conserved because:
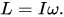
When you reduce the length by half I become one-fourth of it's initial value and so the new angular momentum will be one-forth of the initial angular momentum. If Angular momentum was conserved the the rotational velocity would have increased by 4 times of it's initial value.You are correct that Angular momentum should be conserved and in reality it will happen but, in this question is comparing angular momentum of 2 different systems are compared and so here the conservation is not important because it's not one system but two independent systems.
According to your question, the angular velocity is same in both cases even though the length of string has decreased. So obviously the question is talking about two entirely different cases where the angular momentum are different and hence not conserved.
