3
$\begingroup$

Conservation of angular momentum can be applied when there is no external torque on the system which is isolated. However, my physics teacher gives me a sum that:

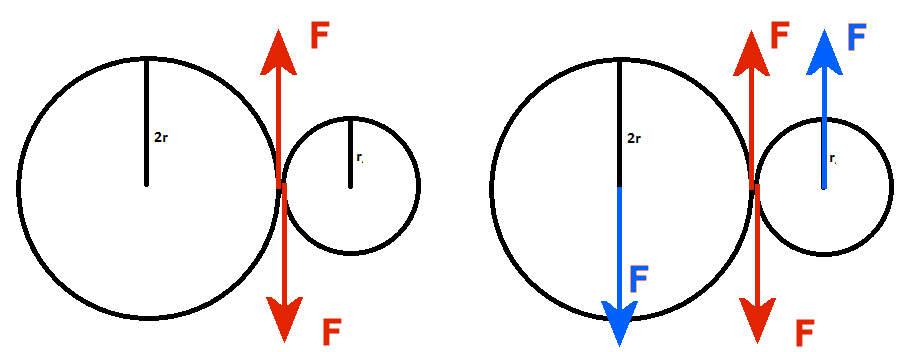
Two discs are pressed against each other until they stop slipping on each other. Find angular speed of the bigger disc when they stop slipping. Disc 1 (mass $m$, radius $r$) and disc 2 (mass $m/2$, radius $2r$). Disc 1 had angular speed $\omega$ initially, whereas disc 2 was at rest initially. enter image description here

I tried to apply the law of conservation of angular momentum in this case since there is no external torque, but my teacher says that angular momentum is not conserved since there is an external torque acting on it. I fail to understand what is the external torque in this case. I don't need the answer to this question, but the reason why the law of conservation of angular momentum cannot be applied, and what is the external torque that acts on this body?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
6
$\begingroup$

Look at the left hand diagram which has the two frictional, equal magnitude and opposite direction. forces (red) acting on the discs.

enter image description here

There are no external forces but each disc has a net force on it and so the centre of mass of each disc will undergo a translation acceleration.

To stop that translational acceleration of the centres of mass of the two discs forces (blue) must be applied to the axles of the discs and these are external forces for a system which is defined to be just the two discs.

Note that there are similar problems where the two discs share a common axis of rotation.
In that case conservation of angular momentum can be used.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Excellent answer, sir! Just like the pulley system in which the support attached to the pulley gives some force to balance it in the air, the hinges over here provide force for stopping the translation acceleration. $\endgroup$
    – Siddharth Venu
    Commented Mar 7, 2017 at 9:01
  • $\begingroup$ @Farcher But do these forces exert a net torque along the axis of rotation? I mean shouldn't their torque equal zero as the pass through the axis so the angular momentum is conserved along the axis of rotation? $\endgroup$
    – Antonios Sarikas
    Commented Dec 29, 2020 at 20:51
  • $\begingroup$ There is an external torque (due to the blue couple) acting on the system of magnitude $F(r+2r) = 3Fr$ and it is in an anticlockwise direction. $\endgroup$
    – Farcher
    Commented Dec 30, 2020 at 0:06

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.