3
$\begingroup$

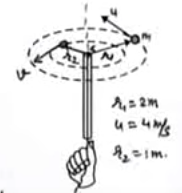
enter image description here

A point mass is tied to one end of a cord, other end of which passes through a hollow tube caught in one hand. The point mass is being revolved in a horizontal circle of radius $r_1$ with a velocity $u$. The cord is then pulled down so that the radius of the circle reduces to $r_2$.

Now, my teacher said that to find the final velocity (say $v$) at radius $r_2$ we can use conservation of angular momentum about center of circle.

But, I do not think that is correct as torque due to gravity ($mgr$) is acting on the point mass. So we should not be able to use conservation of angular momentum. Please tell me if I am going wrong somewhere. Can we conserve angular momentum or not? And why so ?

Improve this question
$\endgroup$

2 Answers 2

Reset to default
1
$\begingroup$

Angular momentum is always conserved. It may or may not be constant within a defined system, depending on whether the external torque about some point is zero or not.

In reality, the plane of motion of the mass is slightly below the support point at the top of the tube. The shape swept out by the string is not planar, but almost conical sloping downward from the tube support to the mass. As a result of this slope, there is an upward force which keeps the mass at a constant height. Otherwise, gravity would pull the mass downward.

(Edited paragraph) Also, the net torque about an instantaneous horizontal axis at the same height as the mass must be zero for a constant vertical height. That means that although the $mgr$ torque which you mention is present, there is also a torque in the opposite vertical rotational sense produced by the tension in the string. If the tension is changed, there is a slight torque which allows the mass to rotate slightly upward or downward as necessary to come to a new equilibrium at a new $r$ and new tilt angle and new tension. This component torque doesn't affect the rotational speed in the horizontal plane. If the horizontal angular momentum component remains constant (no torques about a vertical axis), that makes the calculation straightforward.

Improve this answer
$\endgroup$
5
  • $\begingroup$ +1, but maybe the first sentence could confuse the OP. Angular momentum is generally not conserved in an external gravitational field, take a pendulum. $\endgroup$
    – pppqqq
    Commented Jan 7, 2017 at 17:43
  • $\begingroup$ The application of a torque to a pendulum causes the angular momentum of the pendulum to change. That means the angular momentum of the pendulum is not constant, but the angular momentum is neither created nor destroyed. It simply moves to another part of the universe, outside the defined system of the pendulum. Angular momentum is conserved. Usually we ignore exactly where it went or came from. The torque tells us how fast it's changing in the local system. $\endgroup$
    – Bill N
    Commented Jan 7, 2017 at 17:54
  • $\begingroup$ ↑ for sure. But the OP is clearly concerned with the angular momentum of the bob, not the angular momentum of the world. $\endgroup$
    – pppqqq
    Commented Jan 7, 2017 at 18:10
  • $\begingroup$ @pppqqq True, so the angular momentum of the system related to the horizontal rotation is constant. The angular momentum of the system related to the vertical motion changes slightly and comes to a new, stable value. $\endgroup$
    – Bill N
    Commented Jan 7, 2017 at 18:13
  • $\begingroup$ @BillN Can you show with the help of a diagram as to how tension balances the torque due to gravity ? $\endgroup$
    – user102705
    Commented Jan 7, 2017 at 19:18
1
$\begingroup$

Judging by the sketch, the orbit is in the same plane as the tension in the string/cord, so gravity is being ignored. The only force on the particle is the tension in the string, which is directed towards the centre and therefore exerts no torque about the centre. So angular momentum is conserved.

Are you asking what effect the torque due to gravity might have if it is not ignored as the string is pulled? I am not sure about that myself.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.