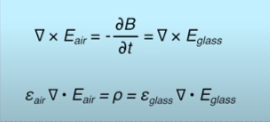Those equations are written for the $E$- and $B$-fields at the boundary. The $E$-field has a discontinuity at the boundary, but the $B$-field is continuous at the boundary, and this can be deduced by Ampere's law.
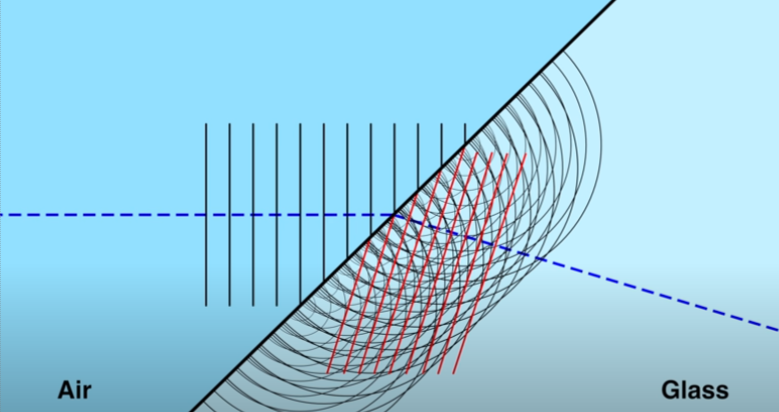
We need to make some notes first. In the video, Don Lincoln is considering the case of $p$-polarized light coming to the air-glass interface. In $p$-polarized light, the $B$-field is parallel to the surface of the interface.
One can use Maxwell's equations to show that the components of the $E$- and $B$-fields that are parallel to the surface must be the same in the limit $z\rightarrow 0+$ from above and in the limit $z\rightarrow 0-$ from below where $z=0$ is the level of the surface.
Since in the case of $p$-polarized light we have that $\vec{B}(x, y, z, t)$ is parallel to the surface at all $(x, y, z, t)$, our proof will demonstrate that $\vec{B}_{\text{air}}(z\rightarrow 0+) = \vec{B}_{\text{glass}}(z\rightarrow 0-)$.
To show our claim, we will use Ampere's law. At the air-glass interface, consider a rectangular loop $C$ of height $h$ and length $\ell$ half-way between the the two media as depicted below.
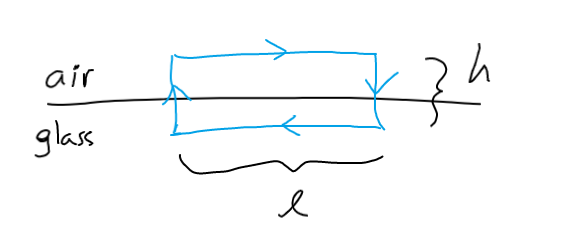
By Ampere's law, we have
$$ \oint_{C} \vec{B}\cdot \text d\vec{l} = \iint_{S} \mu \left[ \vec{J} + \epsilon\frac{\partial\vec{E}}{\partial t} \right] \cdot \text d\vec{A} $$
where the right-hand side is an integral over the rectangle whose boundary is loop $C$. The absolute value of the area integral on the right-hand side is bounded above by $\text{|maximum of integrand|}\times \text{Area}$ where $\text{Area} = h\ell$. As we send $h\rightarrow 0$, we see that $\text{Area} = h\ell\rightarrow 0$, so the area integral in the above equation goes to zero. Hence in the limit of $h\rightarrow 0$, we have
$$ \oint_{C} \vec{B}\cdot \text d\vec{l}\rightarrow 0. $$
Thus, $\vec{B}(z\rightarrow 0+)\cdot \text d\vec{l}_{1} + \vec{B}(z\rightarrow 0-)\cdot \text d\vec{l}_{2} = 0$ for any length element $\text d\vec{l}_{1}$ parallel to the surface. Since the loop goes in opposite directions above and below the surface, $\text d\vec{l}_{1} = -\text d\vec{l}_{2}$ and thus $\vec{B}(z\rightarrow 0+)\cdot \text d\vec{l}_{1} + \vec{B}(z\rightarrow 0-)\cdot (-\text d\vec{l}_{1}) = 0$. Since we can orient the length element $\text d\vec{l}_{1}$ any way we want as long as it is parallel to the interface surface and since $\vec{B}$ is parallel to the interface surface in $p$-polarized light, it follows that $\vec{B}(z\rightarrow 0+) - \vec{B}(z\rightarrow 0-) = 0$, and so $\vec{B}(z\rightarrow 0+) = \vec{B}(z\rightarrow 0-)$, as desired.
Thus, $\partial\vec{B}/\partial t$ is the same as $z\rightarrow 0+$ and $z\rightarrow 0-$. The behavior of $\vec{B}$ does change as you pass from air to glass, but we've shown that it is same at the boundary, meaning it is continuous as you pass through the boundary. This is exactly the same way the parallel component $\vec{E}_{\text{parallel}}$ of $\vec{E}$ is same at the boundary and continuous across the boundary, as the video claims. In fact, a similar proof of this can be devised using Faraday's law.
(Lastly, I should repeat that the $B$-field is parallel to the interface surface only in the case of $p$-polarized light. In the case of $s$-polarized light, things are a bit different. The same reasoning above applies, but it only applies to the component of $\vec{B}$ that is parallel to the interface surface.)