I read about the dispersion of light by a prism and a block (slab), but I don't understand why light bends at all.
I know that red light has the longest wavelength and that energy is inversely proportional to wavelength, hence red light contains the least energy. I also know that it bends the least. But why? Why does red light not bend as much as violet light?
Please don't use Snell's law in your answer.
If you don't want (ray-based) Snell's law, then we can do it using the wave aspect. BTW the analogy totally stands with water waves, with the depth playing the role of refraction index.
-> when the light waves enter the glass, or when water waves enter shallower water, they slow down and wavelength get shorter. This has the effect of tilting the wavefront, and this is the true cause of change of direction in refraction.
And this tilting effect does not have the same amplitude depending of the wavelength (the distance between wave fronts).
Why does a light-beam bend through a prism, and usually more so for violet than red? (And how does it relate to photon energy?)
Firstly, the key you need to understand is that light moves slower in a medium (such as a prism) than it does in nearly free space (such as air). Light is a wave (a propagating oscillation) of the electromagnetic field. Light always travels at the same speed ("c") in free space, including the free space between atoms of a medium. However, it scatters from atoms and molecules. Specifically, light's oscillating electric field perturbs the electron clouds around atoms to undergo simple harmonic motion, and this periodic acceleration of electric charges causes a secondary electromagnetic wave to be radiated outward from each atom. The phase of this secondary wave is delayed with respect to the original wave (because displacement lags acceleration in simple harmonic motion due to inertia of here the electrons). When you add up all of the interference effects between the original wave and the contributions radiated from all the points in the continuous medium, the result is equivalent to if the light simply propagated a bit slower than "c" (while within the medium), but with the same frequency (and hence with a shorter wavelength).
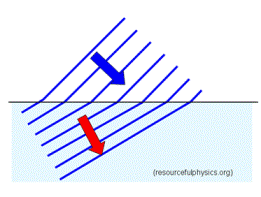
Now, when a plane wave (such as light, or any other wave) impinges at an angle on a zone where its wavelength (the spacing between successive wave-fronts) becomes shorter, the angle of the wave-fronts bends. This is Christiaan Huygens' principle.
What you're really interested in is not the direction of (the normal perpendicular to) the wavefront, but rather, the direction of the light beam as a whole. This is again dictated by interference. Conveniently, the math turns out that the beam bends the same as the wavefront does (and by learning the math you encounter interesting additional affects such as diffraction, where the beam spreads out and changes direction at the edges, and occasionally results in interesting patterns). You can roughly estimate this outcome by drawing Huygens-Fresnel diagrams (where for each point along a wavefront you pencil a circle of radius one wavelength of that zone, and maybe rub out a bit at half-wavelength radii, then most of the thicker concentrated marks will correspond with where most of the beam's energy is propagating).
The reason red usually bends less than violet is simply because violet usually propagates slower through a medium than red does. This is a property of how strongly the particular material interacts with electromagnetic waves of different frequencies (hence, how strongly it re-radiates, which interferes and results in the slowing effect above). This is called dispersion: the dependence between refractive index and frequency.
Note that the details of dispersion are specific to the material. A few materials may bend red more than they bend violet, which is called "anomalous dispersion" (rather than "normal dispersion").
Now it turns out that the dispersion relation can be determined from the absorption spectra of the material. (The math connecting these is the Kramers-Kronig relation.) As you mentioned, the energy of a red light beam is divided amongst many photons, whereas the energy of a violet light beam is more concentrated among fewer photons. Where this starts to come into the explanation is that anomalous dispersion usually occurs close to a resonance peak (where the photons have almost exactly the right amount of energy to excite the atoms/molecules to a different quantum state).
It's comprehensive and detailed explanation was given by Fermat. Known as Fermat's Principle or The Principle of Least Time. Which in turn gives the explanation for Snell's law.
A very detailed & beautiful explanation here by Feynman. http://www.feynmanlectures.caltech.edu/I_26.html
About Fermat. www-history.mcs.st-and.ac.uk/Biographies/Fermat.html
If you're wondering about the mathematics then check out 'Calculus of Variations'.
Below I've tried to put what I know:
The first way of thinking that made the law about the behavior of light evident was discovered by Fermat in about 1650, and it is called the principle of least time, or Fermat’s principle. His idea is this: that out of all possible paths that it might take to get from one point to another, light takes the path which requires the shortest time.(This isn't always true, it just requires the first derivative to be zero, to learn more use this link -> https://math.berkeley.edu/~strain/170.S13/cov.pdf)
Before we continue any further we must, however, make an assumption about the speed of light in water. We shall assume that the speed of light in water is lower than the speed of light in air by a certain factor, $n$.
$T=\int dt = \int \frac{dl}{v} = \frac{1}{c}\int ndl $
The total travel time is the integral of the distance $d$ over the speed (itself a function of position). The index of refraction is $n = \frac{c}{v}$, where $c$ is the speed of light in vacuum, so I can rewrite the travel time in the above form using $n$. The integral $\int ndl$ is called the optical path.
When we solve for this changing '$n$', we get to Snell's Law which was not the point of this question. So without previous knowledge about the wave nature of light Fermat's Principle can explain most phenomena in geometric optics.
I came to know that red light has the longest wavelength and then I read a formula, Energy is inversely proportional to wavelength.
This is a quantum mechanics formula, $E=h\nu,$ where $\nu$ is the frequency.
That means that red light contains the least energy . And it bends the least. WHY? Why does it not bend as much as violet ( I know they have more energy but what makes them bend ? )
A crystal is a many body organized quantum mechanical entity. Even though composed by zillions of atoms, it can be treated quantum mechanically as one entity when scattering happens, a photon hitting a crystal. The quantum mechanical solution will give a probability distribution for the scattering of a single photon to get through the crystal. This probability distribution has a sharp maximum at the dispersion angle of the crystal. This is BECAUSE the classical framework emerges from the underlying quantum mechanical, has to be consistent and it can be shown to be. The difference in the energy of the photon makes a difference in the maximum of the scattering angle because the energy enters scattering equations.
To answer this question first you need to understand what prisms are made of, usually glass, that is silica (SiO2).
Now the atomic size of for example an atom inside the prism is 60 pm, that is 0.06 nm.
Now this size is very small compared to visible light photons' wavelength which is about 400-700 nm.
When the photon's wavelength is much bigger then the atom's size they interact with, the interaction can be described (and in the case of glass is best described) by elastic scattering (Rayleigh), by the way this is the reason why the sky is blue.
is the predominantly elastic scattering of light or other electromagnetic radiation by particles much smaller than the wavelength of the radiation.
https://en.wikipedia.org/wiki/Rayleigh_scattering
Now this causes the photons with shorter wavelength to interact with the atoms more (higher probability), causing the angle to change more in the case of shorter wavelength.
Just like the sky is blue, that is, the shorter wavelength photons get scattered more (higher probability), and change angle more into our eyes to make the sky look blue, analogously the shorter wavelength photons will interact with the atoms in the prism more and scatter more and change angle more.
As you say, the red light photons contains the least energy, have the longest wavelength (in the visible range), and interact with the atoms the least, thus, they follow an almost straight path through the prism.
