1
$\begingroup$

How does light going through a medium make light refract? Here’s a good picture : enter image description here

The problem with this analogy is it depends on the students or light being connected to each other and as far as I know, parallel photons are not connected to each other. For example if there was a single student, they could slow down and continue in their original direction. The rotation of the line of students depends on the slowing down of the first student to affect the motion of the second student and cause their direction to deviate and so on. I can not think how this works for photons. Is this analogy flawed?

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ "I understand why the light will turn to an angle" and "continue in the direction" cannot simultaneously happen; it means you didn't actually understand. $\endgroup$
    – naturallyInconsistent
    Commented Mar 30 at 22:50
  • $\begingroup$ @naturallyInconsistent so I understand why there will be an angle, but I don’t know why that’d make the light turn. $\endgroup$
    – Wyatt
    Commented Mar 30 at 22:54
  • 1
    $\begingroup$ The angle is the turn. There is no angle without the turn. $\endgroup$
    – naturallyInconsistent
    Commented Mar 30 at 23:11
  • $\begingroup$ Thanks for that edit @KDP, it captures what I’m confused about. $\endgroup$
    – Wyatt
    Commented Mar 31 at 0:00

4 Answers 4

Reset to default
0
$\begingroup$

The photon picture of light (and the marching soldiers analogy) may not be perfect to understand the refraction of light. For understanding this the wave picture is more intuitive. Consider light passing from medium 1 (with higher wave speed) to medium 2 (with lower wave speed).

refraction of waves
(screenshot of animated image at ISVR - Refraction, Snells Law)

Because of this the wavelength is shorter in medium 2 than in medium 1. This makes the wave fronts (and hence also the light rays) bend when passing the medium border.

Improve this answer
$\endgroup$
0
$\begingroup$

The analogy is poorly described and this has led you to make several mistakes in your interpretation.

The problem with this analogy is it depends on the students or light being connected to each other ...

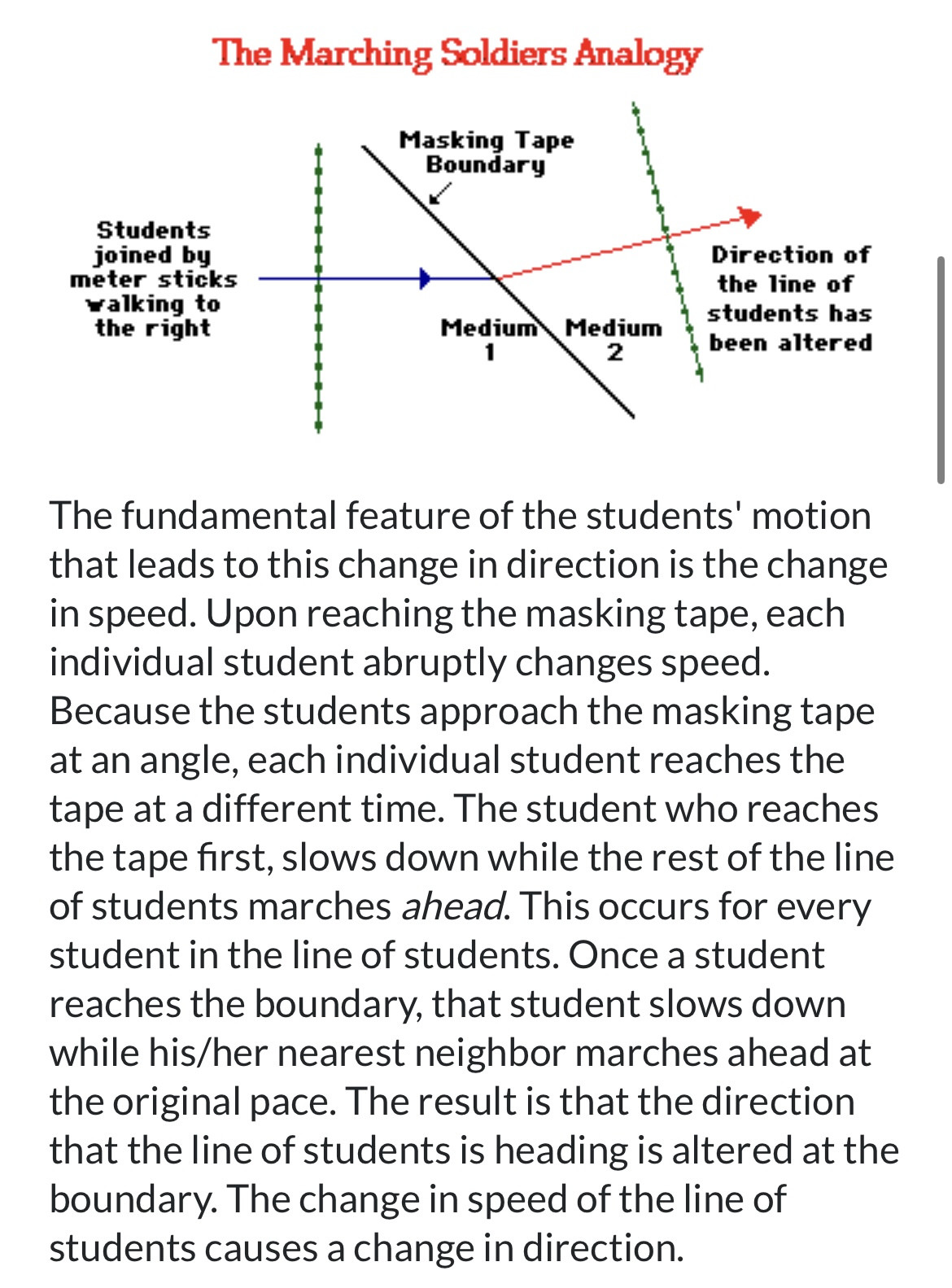
No, it doesn't. The "meter sticks" in the analogy are unnecessary - in fact, the distance between students changes as the line moves from Medium 1 into Medium 2. The analogy only depends on the students in the line moving in the same direction and at the same speed as each other in Medium 1 and at a slower speed in Medium 2. No connection is necessary, and they don't even have to be evenly spaced along their line.

For example if there was a single student, they could slow down and continue in their original direction.

All of the students do continue to walk in the same direction as they pass the tape. It is the direction of the student line (which is the analogue of the wavefront of the light) that changes, not the direction of motion of any individual student.

The diagram is misleading here because each student in the right-hand line should be on the same horizontal line as their counterpart in the left-hand line.

The rotation of the line of students depends on the slowing down of the first student to affect the motion of the second student and cause their direction to deviate and so on.

Once again, no it doesn't because the direction of motion of each individual student is unchanged. No connection or communication between students is required.

Improve this answer
$\endgroup$
0
$\begingroup$

You have to know the Fermat's principle if you want to know the light behavior exactly. In this law, light choose its trajactory by minimizing its travel time.

By this, light could be bend its trajactory since the difference of speed of light .

Improve this answer
$\endgroup$
0
$\begingroup$

The analogy of connected individual particles behaving like a wave is wrong and you are right to be confused. The difference between particle travel and wave travel is that a particle's linear path always covers the shortest distance between two points. This is Heron's principle and is accurate when explaining the reflection of light.

Heron's principle when applied to light transmission fails for the same reason you are confused by the marching student analogy. The marchers will cover the same linear path in different times and there is no reason for the particles to change direction.

A wave's path covers the shortest time between two points and is explained by Fermat's principle. This means a wave can change direction to meet this requirement. I've included links to Herons principle and Fermats principle to help.

https://proofwiki.org/wiki/Heron%27s_Principle_of_Reflection

http://electron9.phys.utk.edu/optics421/modules/m1/Fermat's%20principle.htm

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.