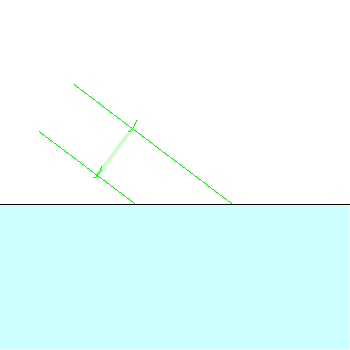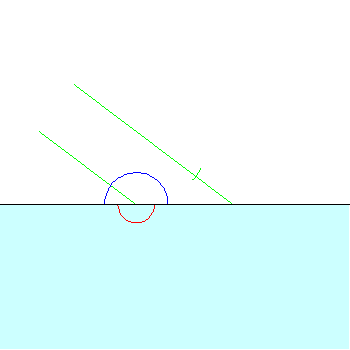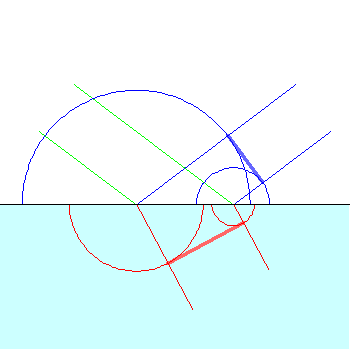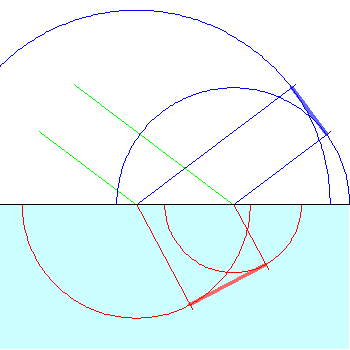
I am not a physicist, but rather a middle school science teacher. Please be gentle. The marching soldiers has been a really good analogy for explaining why a change of direction is caused by hitting the boundary of a new medium at a non-normal angle. In a pre-Covid time, you could actually have students do this in the classroom. Very fun. Except... what does the pole locking the marchers together represent?
Yes, it's a wavefront, but what makes that wavefront stay together in shape? I've never seen that explained. I think I understand that being in the same phase is what makes that surface of points a wavefront, but is this a descriptive definition or a prescriptive one? What keeps a wavefront together in the same shape, in perpetuity?
Suppose we are in a universe where change in direction does not happen passing through a new medium. We start the marching soldier analogy: Alice is connected to Bob by a pole, and Alice hits the slower medium first because they are approaching the medium-boundary at a non-normal angle. We would then say that holding the same pole as Alice makes Bob slow down too even though he's still in the faster medium because that is the only way to preserve direction— the thing we're observing in this supposed universe. We would just say that of the analogy: the pole does it, they're connected (sharing information about velocity?), so Bob slows down too. But why? Maybe the pole has some property that keeps it from turning, so Bob has to slow down too. Maybe in this universe, the definition of a wavefront— or whatever this universe calls the pole— is the set of all points where the wave stays in the same direction. That does not happen in our universe, so we don't have to answer that.
In our universe, it is still the analogical pole that does it. (Is it possible that saying the "pole does it" is doing too much work in my understanding? Where are the cause and effect happening in the real world as opposed to the analogy?) In our universe, a wavefront is the set of all points in a wave that is at the same phase. Okay. Alice slows down in the new medium, but Bob at the other end of the pole in the faster medium does not, so the pole turns. But why is there a pole? Why is the wavefront staying in phase through its shape when that's just the definition of what the wavefront is? It feels a bit like circular reasoning to me: the wavefront is changing direction because the wavefront... is a set of points in a wave that we observe to be changing direction?
Does this question make any sense?? It is obviously something I don't understand about what a wavefront is... can anybody explain it? Why does being the same phase lock you in shape? It seems like information has to be shared between photons for this to work, so that's my hint that the answer is getting quantum. But please explain it? I am comfortable with pop-sci explanations of quantum electrodynamics, what's the leap I need? Or maybe it's not quantum and it's actually much simpler than I make it out to be?
Essentially, what makes the wavefront a prescriptive thing? The wavefront is a set of points in the same phase, what makes them stay that shape (we also just accept the pole doesn't shrink, bend, or deform in any way) even when a part of it starts changing speed?