(Georgi chapter 14) Why does the $n=2$ states transform under the 5+1 of the angular momentum?

Question

In Georgi's Lie algebra in particle physics, chapter 14, the 3D harmonic oscillator is studied. The systems exhibits $SU(3)$ symmetry in the energy levels, we can construct the 8 generators of the $SU(3)$ using the creation/annihilation operators. We also notice that a subgroup of the 8 generators generate angular momentum (so the 3 generators of $SO(3)$ symmetry).

But then Georgi take an example: if we consider the 6 states that have $n=2$ (that lie in the (2,0)=6 rep of $SU(3)$), they transform like 5+1 under angular momentum. I'm not sure that I understand what this means. Does it mean that we can find some linear combinations of the 6 states that is invariant under the the action of the 3 generators of $SO(3)$ (giving the singlet state), and that we can find 5 states that the just transform in each other under the action of the $SO(3)$ generators? This may be a dumb question, but I'm a bit stuck on this.

Answer 1

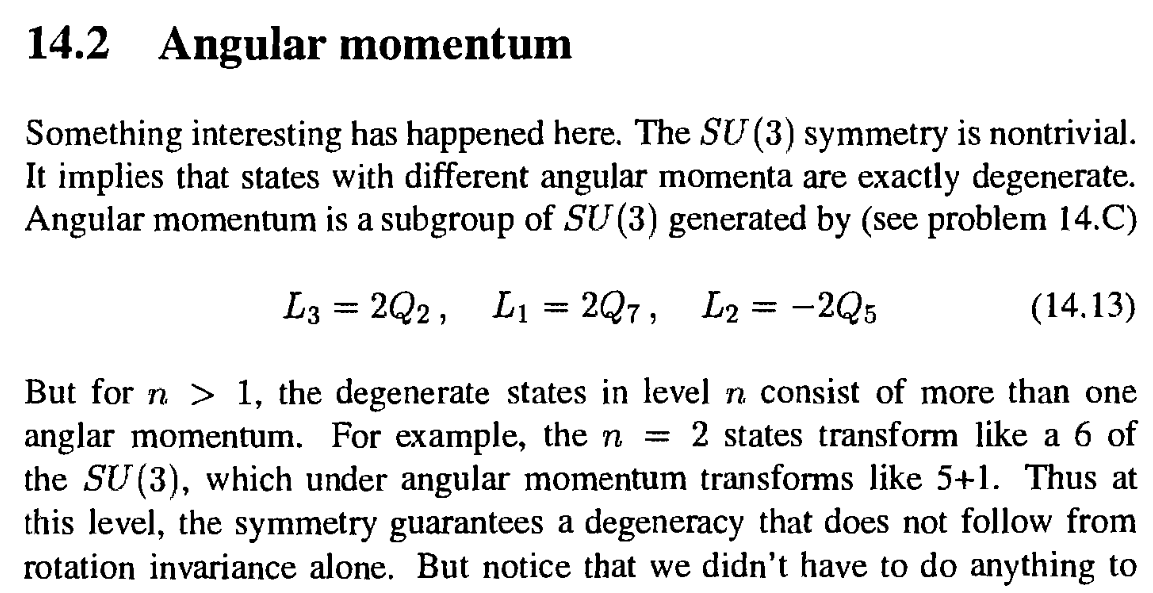
If you go back to the undergraduate study of the 3d harmonic oscillator, you can check that $n=2$ states contain $\ell=2$ and $\ell=0$ states. And yes, it means precisely what you think: you can organize the $n=2$ states to one is fully invariant and the remaining 5 transform amongst themselves under the action of $\mathfrak{so}(3)$

The example is important because it illustrates the difference between $\mathfrak{so}(3)$ and $\mathfrak{su}(2)$. Whereas the algebras are isomorphic, they "sit" differently inside $\mathfrak{su}(3)$. Irreps of $\mathfrak{so}(3)$ must have integer angular momentum, whereas irreps of $\mathfrak{su}(2)$ can have half-integer value of $j$.

In particular, the defining representation, of dimension $3$ and with Dynkin label (1,0), contains a single irreducible representation $\ell=1$ of $\mathfrak{so}(3)$ but a direct sum $\frac{1}{2}\oplus 0$ of $\mathfrak{su}(2)$ irreps. Inside $(1,0)$, the $\mathfrak{so}(3)$ generators are such that $iL_{k}$ is a real antisymmetric $3\times 3$ matrix, whereas of course the $\mathfrak{su}(2)\oplus \mathfrak{u}(1)$ generators form $2\times 2$ blocks.

The adjoint representation, of dimension $8$ and with Dynkin label $(1,1)$, contains $L=1$ and $L=2$ $\mathfrak{so}(3)$ generators: they are linear combinations of the root vectors. On the other hand, the $\mathfrak{su}(2)\oplus \mathfrak{u}(1)$ contents is found by identifying roots with generators.

An alternative way of stating this difference is that the $\mathfrak{su}(3)\downarrow \mathfrak{so}(3)$ branching rules are not the same as (and much more complicated than) the $\mathfrak{su}(3)\downarrow \mathfrak{su}(2)\oplus \mathfrak{u}(1)$ branching rules. In particular, for multi-particle states, there are "missing labels" when using the $\mathfrak{so}(3)$ labelling schemes, whereas $\mathfrak{su}(2)\oplus \mathfrak{u}(1)$ provides enough labels to completely distinguish states.

A lot of applications of $\mathfrak{su}(3)\downarrow \mathfrak{so}(3)$ were pioneered by

Elliott JP. Collective motion in the nuclear shell model. I. Classification schemes for states of mixed configurations. Proceedings of the Royal Society of London. Series A. Mathematical and Physical Sciences. 1958 May 6;245(1240):128-45.

Within this model, the $L=2$ generators are identified with the quadrupole moments, while the remaining $L=1$ generators are angular momenta. Thus, the model can predict quadrupole transitions between angular momentum states of nuclei when $\mathfrak{su}(3)$ is a valid global symmetry.

An expression for the quadrupole and angular momentum generators can be found in

Rowe DJ, Le Blanc R, Repka J. A rotor expansion of the su (3) Lie algebra. Journal of Physics A: Mathematical and General. 1989 Apr 21;22(8):L309.