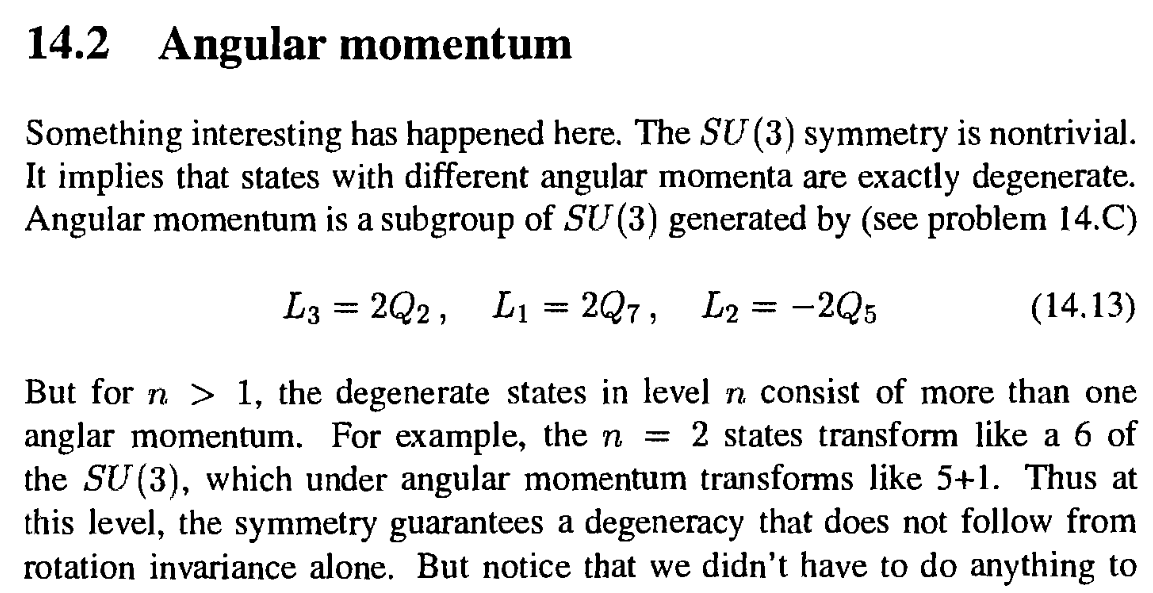
In Georgi's Lie algebra in particle physics, chapter 14, the 3D harmonic oscillator is studied. The systems exhibits SU(3)$SU(3)$ symmetry in the energy levels, we can construct the 8 generators of the SU(3)$SU(3)$ using the creation/annihilation operators. We also notice that a subgroup of the 8 generators generate angular momentum (so the 3 generators of SO(3)$SO(3)$ symmetry).
But then Georgi take an example: if we consider the 6 states that have n=2 $n=2$ (that lie in the (2,0)=6 rep of SU(3)$SU(3)$), they transform like 5+1 under angular momentum. I'm not sure that I understand what this means. Does it mean that we can find some linear combinations of the 6 states that is invariant under the the action of the 3 generators of SO(3) $SO(3)$ (giving the singlet state), and that we can find 5 states that the just transform in each other under the action of the SO(3) generators $SO(3)$ generators? This may be a dumb question, but I'm a bit stuck on this.
Thank you for your help !