If the following graph is given then, why is the displacement equal to the area of the shaded triangle above the axis minus the are of the shaded triangle below the $t$ axis.
Why can it not be area of the shaded triangle above the axis minus the area of the trapezium
I get that it’s because we take the area with respect to $t$ axis. But I don’t understand why.
Velocity is defined as $\vec v = {d \vec s \over dt}$ where $\vec s$ is position which varies with time. For one dimensional motion in say the $x$ direction $v = {dx \over dt}$. Therefore $x(t_1) - x_0 = \int_{0}^{t_1} v(t) dt$ where $x(t_1)$ is the final displacement in the $x$ direction at time $t_1$, $x_0$ is the initial position, and $v(t)$ is the time dependent velocity in the $x$ direction. $\int_{0}^{t_1} v(t) dt$ is the area under the $v(t)$ versus $t$ curve between times $0$ and $t_1$, and this area is the total $x$ displacement $x(t_1) - x_0$. For your example $v(t)$ is linear in time, initially in the positive $x$ direction which increases $x$, but later at 1 sec $v(t)$ is in the negative $x$ direction which decreases $x$; the shaded area you show is correct for the area representing the total displacement.
The displacement is similar to distance (but with a sign), $d = v \times t$ where the $v$ should include the sign and $t$ is a positive increase in time.
For times below $1$ second, it's the area of the first shaded triangle.
For times above $1$ second $v$ is negative, so the displacement is the area of the second shaded triangle, but that area counts as negative - it means the moving object is heading back towards where it started.
Displacement is the distance from the starting point, not the total distance traveled.
So the total displacement is the first area minus the second area.
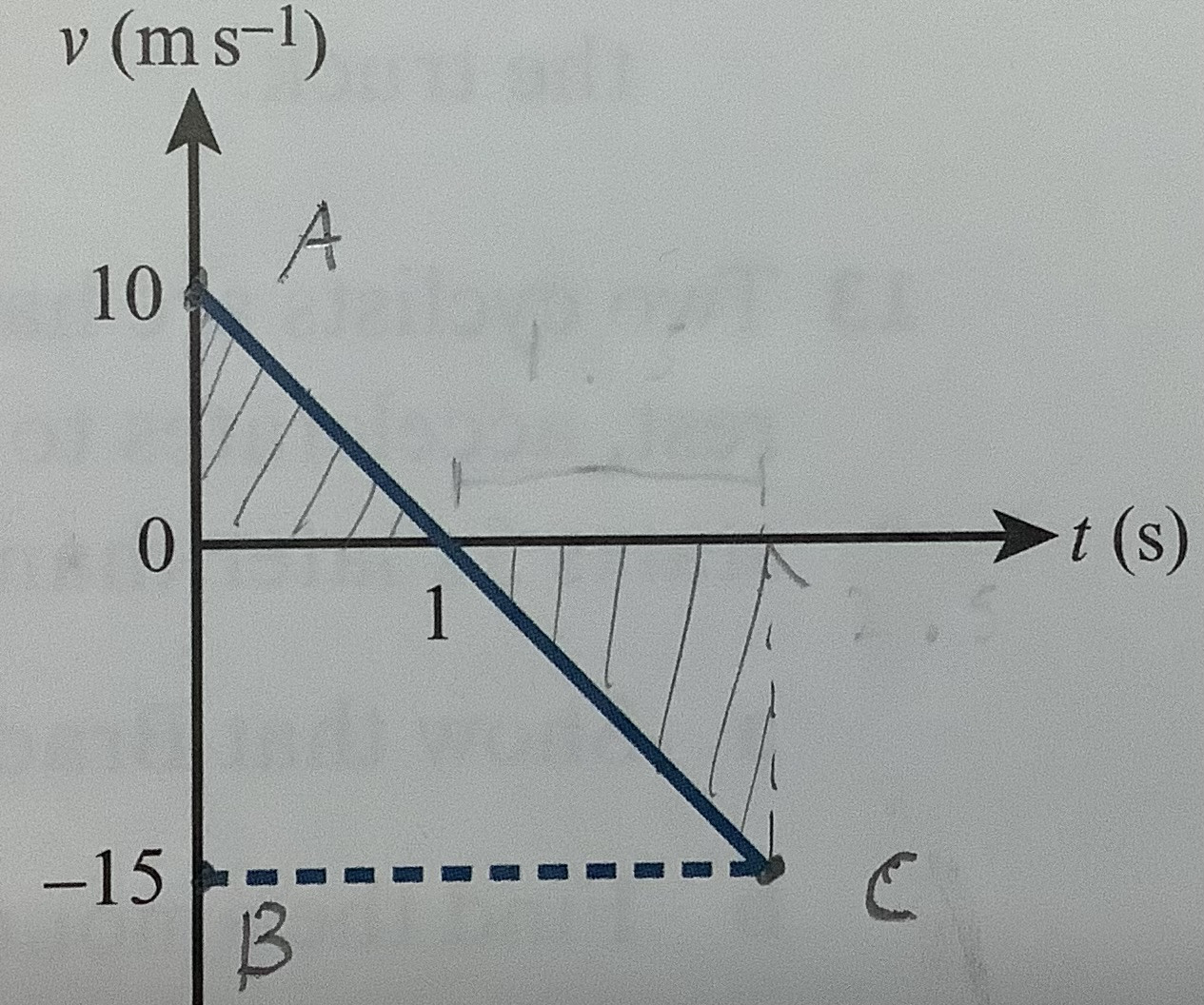
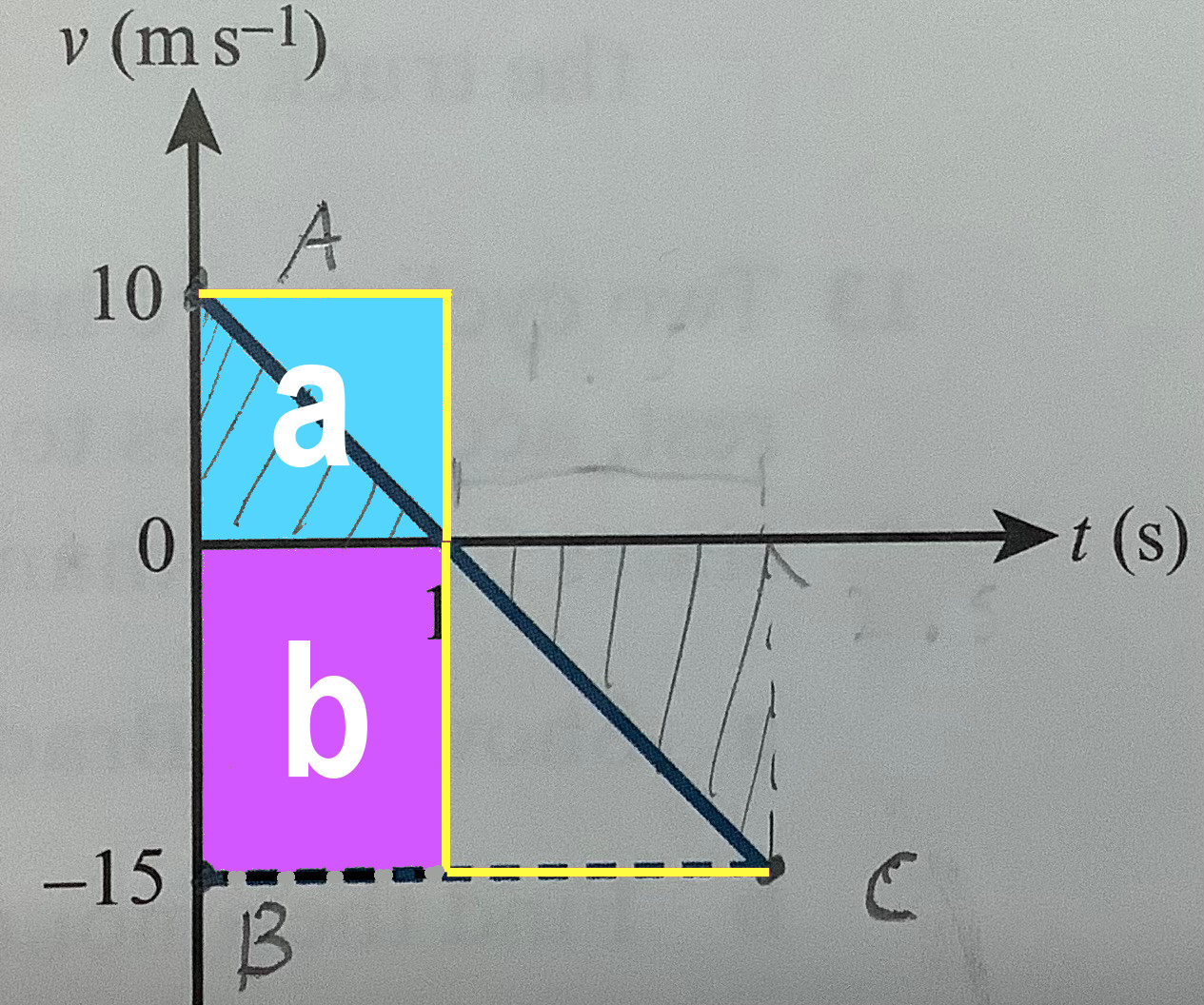
The "$v$-axis", as you call it, is really just the line where $v=0$.
For parts of a $v$-vs-$t$ curve where $v=0$, the object isn't moving - so nothing to add to the net displacement.
When $v(t)$ starts to go slightly over $v=0$, the object starts moving slowly upwards (I'm assuming the 'positive' direction is plotted going upwards, which is usually the case for $x$-vs-$t$ graphs). So the teeny bit of area between the curve and the $v$-axis is what contributes to the net displacement.
As $v(t)$ gets further away from $v=0$, the object is moving faster, and there's a much larger area to add.
Same goes for $v(t)$ below the $v$-axis, except the object is moving in the opposite direction, so you have to subtract it instead of adding it.
To get the total displacement, you need to add all the upward movement (area above $v=0$) and subtract all the downward movement (area below $v=0$).
The "reason" for something being obviously not true can sometimes be gained by considering at similar example.
In the diagram below consider the motion defined by the graph drawn in yellow.
Your "addition" of the area of a triangle and the area of a trapezium now becomes the "addition" of the area of rectangle $a$ and the area of rectangle $b$ which does not take account of how long the movement is after time $=1$.
