Can a black hole form a focal point of a nearby star at a certain distant point by gravitational lensing? Also, can the BH make a virtual picture of that star to a distant observer so the star looks distorted due to the same effect of gravitational lensing? But as I understand grav. lensing there should also be an aberration of the light beams at the focal point...
-
$\begingroup$ Gravitational lenses do not have a focal point. Parallel rays converge at different places along the optic axis depending on their distance from the axis. $\endgroup$– John RennieCommented Sep 21, 2021 at 6:58
-
1$\begingroup$ Possible duplicate: Do gravitational lenses have a focus point? $\endgroup$– Chiral AnomalyCommented Sep 21, 2021 at 13:03
1 Answer
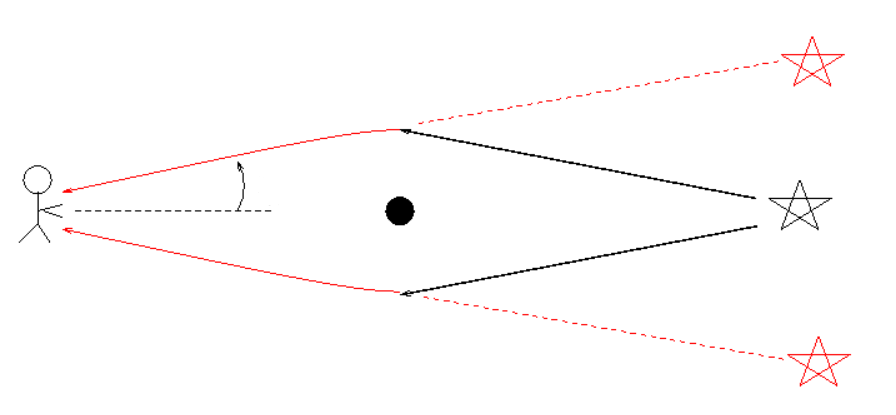
Yes it can, though the image of the object (due to the gravitational lensing of a black hole or whatever the massive lensing object may be), appears as a ring$^1$, and not a traditional single focal point e.g., convex lens refracting light.
To get an idea why the image formed is a ring, consider the following diagram:
As one can see here, the star behind the lens will appear in "two" separate locations (two dimensions). But in actual three-dimensional space, the star will appear at all points forming a circle: half of it going into, and the other half coming out of, the page (perpendicular to the page). An example can be seen in this image taken by the Hubble telescope:
This shows the lensing object and ring image as concentric. Here, the gravitational lens itself is a galaxy pictured in the center with the image (of another distant galaxy behind the first) surrounding it (also note the blue-shifting). These images are termed "Einstein rings". The image does not form at a single focal point as can be seen, but rather around the lens forming a ring. These are many smaller images of the distant galaxy located at all points on the ring. Courtesy: Wikipedia.
From the link above:
Pictured above, the gravity of a luminous red galaxy (LRG) has gravitationally distorted the light from a much more distant blue galaxy. More typically, such light bending results in two discernible images of the distant galaxy, but here the lens alignment is so precise that the background galaxy is distorted into a horseshoe -- a nearly complete ring. Since such a lensing effect was generally predicted in some detail by Albert Einstein over 70 years ago, rings like this are now known as Einstein Rings.
$^1$ This effect is predicted by general relativity. The equation $$\tag 1\Delta\theta = \frac{4GM}{r c^2}$$ tells us how much massive objects deflect light in terms of the distance of the rays from the optical axis, which leads to the kinds of images we see above.
For a standard convex lens, the further we move from the center $r$, rays parallel to the axis will deflect by a greater angle, and so $$\Delta\theta\propto r$$ or $$\Delta\theta=kr$$ where in the thin lens approximation, the constant $k=\frac{1}{f}$.
But note in equation (1) we now have $r$ in the denominator. For a gravitational lens (like a blackhole), rays of light coming in parallel will not converge at the same points as we move further from the axis (the equation suggests the magnitude of the deflection is inversely proportional to $r$, or the angle decreases for parallel rays further from the center of the axis).