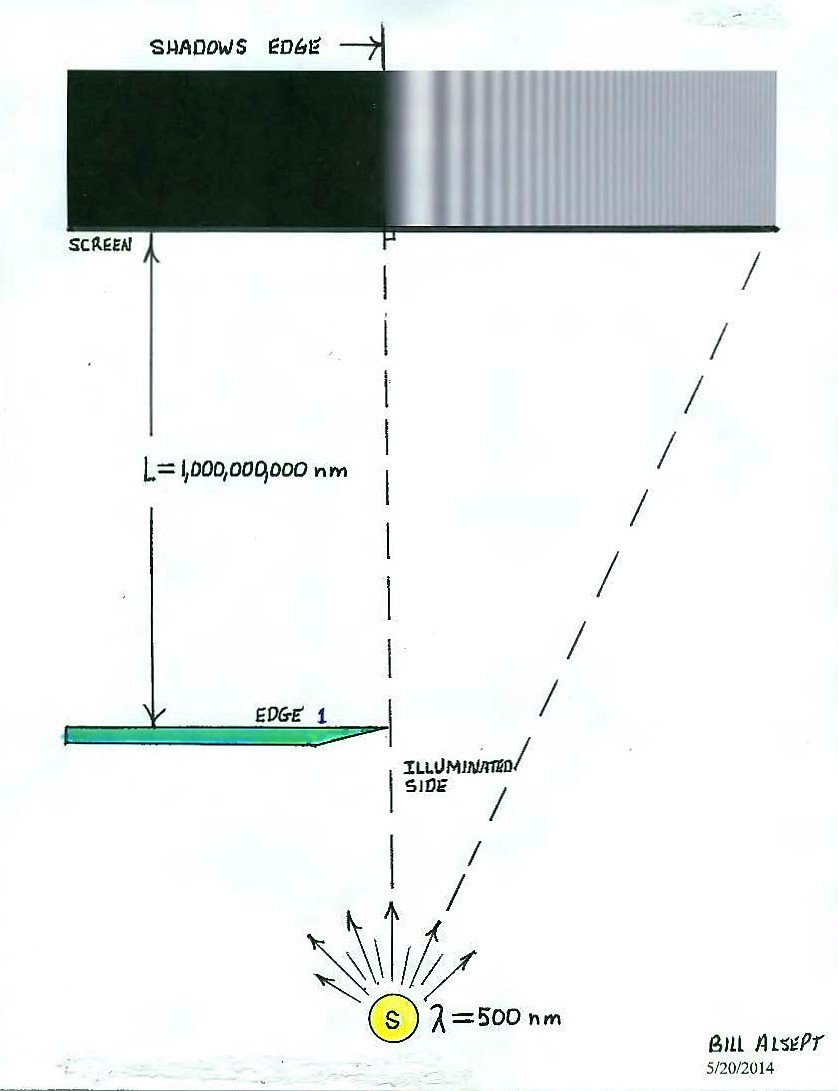
Diffraction, at least as its commonly explained, happens when light crosses an obstacle "of size in the same order as its wavelength". For example, a small slit. However, in one of my labs, we used ~0,2 mm slits; while using light in the order of 650 nm. That's a difference of 3 orders in magnitude; yet diffraction still happened as expected.
Is the simplistic definition of diffraction wrong? Or is the margin larger than the same order of magnitude?
Obstacles of the same order of magnitude as the wavelength give the biggest diffraction effects, but they shouldn't, in my opinion, enter into the definition of diffraction.
Anything that cuts off part of a wavefront causes diffraction. For example, the ability of an optical telescope to resolve apart two stars of small angular separation is ultimately decided by the diameter of the objective mirror or lens, and the spread of the central maximum of its diffraction pattern. And here we may have 5 or even 6 orders of magnitude difference between the wavelength and the linear dimensions of the aperture!
You don't need a slit for diffraction. Photons will diffract at a single edge. 
