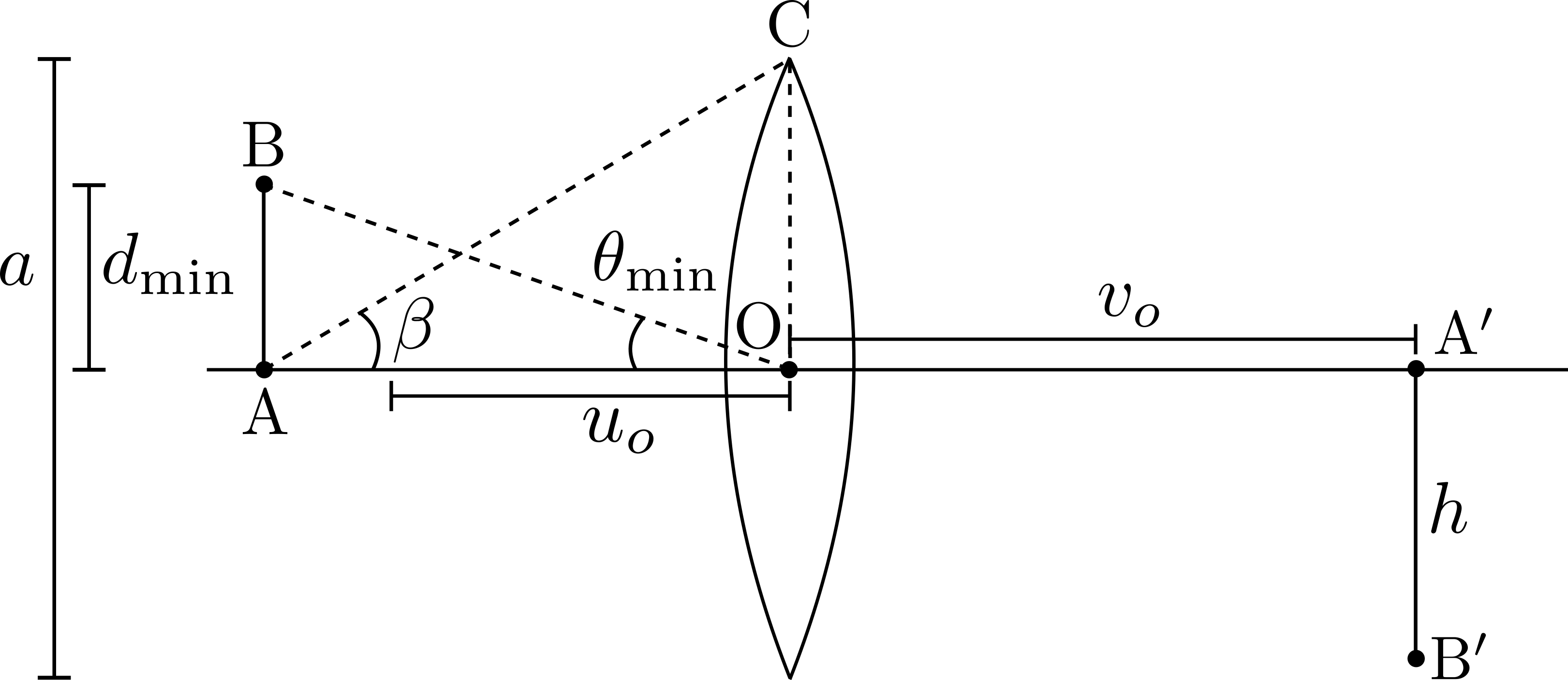
Even under laboratory conditions, the light intensity collected by a microscope can be low, so a larger aperture is in general desirable. However, as you correctly point out, what matters is not the physical aperture (i.e. the radius of the objective lens) but the angle $\beta$. The quantity
$$ NA = n\sin \beta$$
is called numerical aperture and $n$ is the refractive index of the medium in front of the objective lens and
$$ \tan\beta = \frac{R}{f_{OL}} $$
where $R$ and $f_{OL}$ are, respectively, the radius and focal length of the objective lens.
The numerical aperture is related to the resolution of the imaging system via Rayleigh's law
$$ d_{min} = 0.61 \frac{\lambda}{NA}$$
Therefore, the resolution and the amount of collected light can be improved by using larger numerical apertures. This can be obtained by enlarging the lens or by reducing the focal length. Microscope objectives are typically designed to work with a tube lens, therefore the magnification will be the ratio of the focal lengths
$$M = \frac{f_{TL}}{f_{OL}}$$
Since the detector is typically a pixel-based camera it is pointless to increase the optical resolution more than the size of the pixel. Therefore, $Md_{min}> d_{pixel}$ or the optical resolution will be lost by the lack of digital resolution. From this last equation, it should be clear that it is more convenient to reduce the focal length instead of enlarging the size of the objective lens. Moreover, compactness is not a bad thing!