0
$\begingroup$

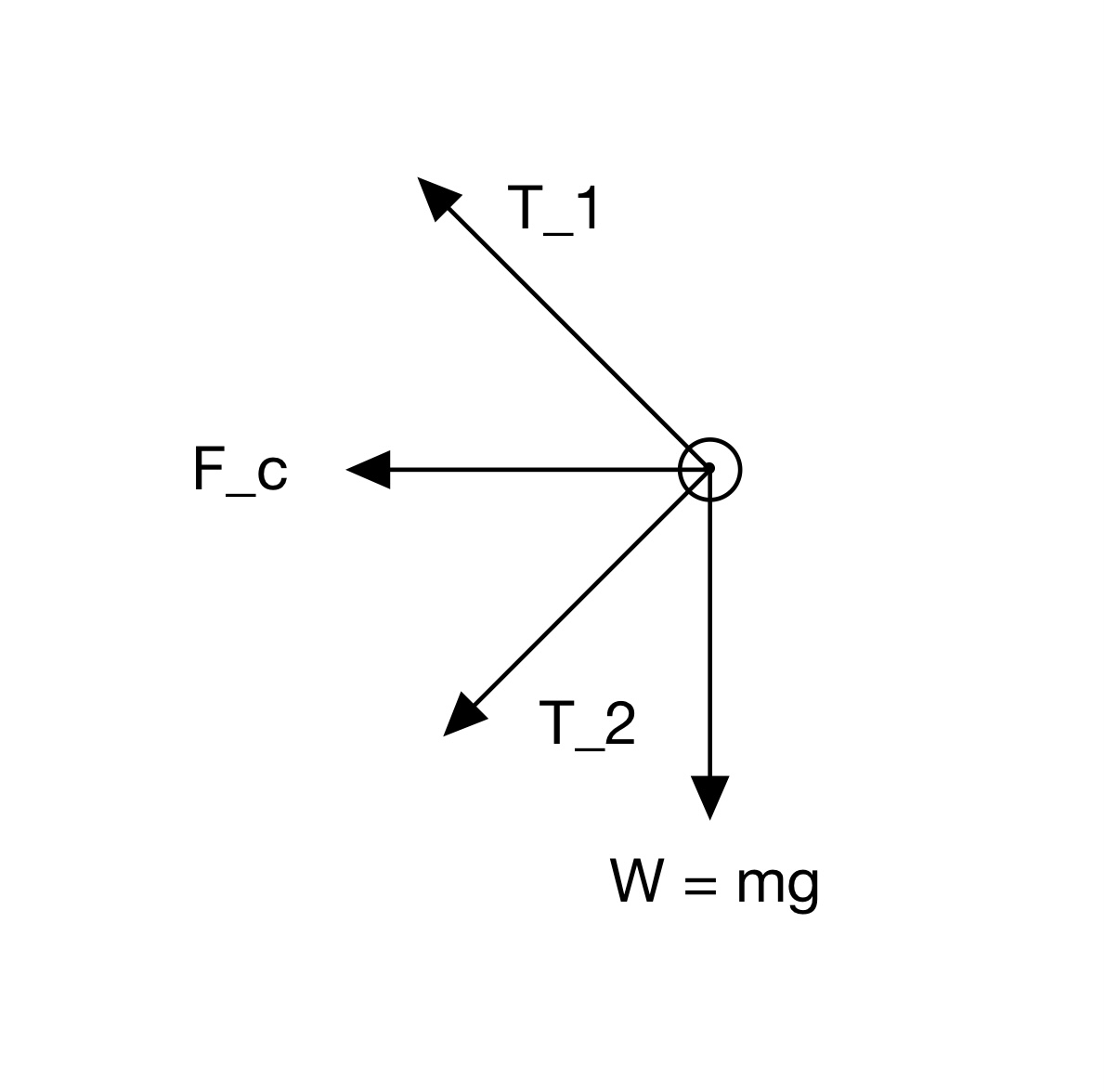
The problem is the following: enter image description here

I notice that this is an equilateral triangle meaning that the angle below and above the dotted horizontal line is $\frac{\pi}{6}$ rad. I then draw a force body diagram of the ball:

enter image description here

And the sum of forces must be $0$: $$ T_1 + T_2 + \frac{mv^2}{r} + mg = 0$$ I do not know how to proceed from here as I feel like I am missing information.

Improve this question
$\endgroup$
1
  • $\begingroup$ You need to use vectors. $\endgroup$
    – Bill N
    Commented Dec 29, 2020 at 4:30

2 Answers 2

Reset to default
0
$\begingroup$

In this case, it will be much easier to use $T \cos\theta$ and $T\sin\theta$ instead of $T_x$ and $T_y$. Let $\theta$ be the angle angle between the $T$ and $F_c$ force.

In this type of question, you'll want to decompose your net force into components. Notice that:

(a) The ball is not moving in the $y$ direction -- therefore, the $y$ component of net force is zero. $\Sigma F_y = 0 \quad\implies\quad mg+T_2 \sin\theta = T_1\sin\theta$.

(b) The ball is undergoing circular motion. Therefore, the component of the net force directed towards the center equals $mv^2/r$. As such, $\dfrac{mv^2}{r} = T_1 \cos\theta + T_2 \cos\theta$.

The reason I used expressed the tension in terms of its length and angle is because: had I expressed it as $T_x$ and $T_y$ instead, then, I'd need two more equations to solve the resulting system. In this case, using the $T_x=T\cos\theta$ and $T_y = T\sin\theta$ is faster and easier.

Improve this answer
$\endgroup$
2
  • $\begingroup$ -1 Although your analysis is correct, this is too close to a complete solution to a homework problem, in my opinion. We shouldn't provide homework solutions on this site. Michael Siefert gives a more appropriate answer. $\endgroup$
    – Bill N
    Commented Dec 29, 2020 at 4:33
  • $\begingroup$ I agree that the equations were a bit too specific, but I wanted to make a point out of expressing the tension components in their polar form. In Michael's answer comment section, OP expressed the tension as $T_x$ and $T_y$ which would have caused some problems when solving the equations, since OP thought that he's 'missing information.' Thus, I wrote down the equations. I assume a quick google search would reveal dozens of (solved) similar questions with a nearly identical approaches, so I figured I would explain the equation setup, hopefully clearing everything up. Thank you for the feedback $\endgroup$
    – user256872
    Commented Dec 29, 2020 at 6:01
0
$\begingroup$

There are two conceptual problems in your approach.

  1. The equation you write down for the forces must be a vector equation. In particular, this means that the vertical component of the equation must be satisfied, and the horizontal component of the equation must be satisfied. This allows you to get two separate equations involving $T_1$ and $T_2$, which will be sufficient to solve the problem. (Right now you have one equation for two unknowns.)

  2. The force $mv^2/r$ is not a separate force on the object. Rather, the object experiences three forces (the two tensions and gravity); and if we assume that the object is executing uniform circular motion, then the net force on the object must be $mv^2/r$ inwards (not zero, as you seem to be implying in your equation above.)

Improve this answer
$\endgroup$
3
  • $\begingroup$ @MichealSeifert But then don't I just get $$T_{1_y} + T_{2_y} = mg$$ and $$T_{1_x} + T_{2_x} = F_c$$ still resulting in too much variables? $\endgroup$
    – Stanislas CASTELLANA
    Commented Dec 28, 2020 at 20:26
  • $\begingroup$ @StanislasCASTELLANA: $F_c$ is known, since $m$, $v$, and $r$ are known. Take a look at my point #2 above again. $\endgroup$
    – Michael Seifert
    Commented Dec 28, 2020 at 20:30
  • $\begingroup$ @MichealSeifert Thank you Sir $\endgroup$
    – Stanislas CASTELLANA
    Commented Dec 28, 2020 at 20:53

Not the answer you're looking for? Browse other questions tagged or ask your own question.