Why does atmospheric drag speed up satellites?

Question

I've read that old satellites slowly lose altitude when encountering the very thin atmosphere, and strangely enough they speed up. My theory is that the work done by the drag force is insignificant in comparison to the whole energy of the orbiter, $$ E = -\frac{GmM}{2r} $$ so it essentially pushes it to a lower orbit while mostly conserving the total energy. This would explain why it speeds up, but I can't quite visualize how a force that acts tangentially to the path can produce radial deviations .

Answer 1

I can't quite visualize how a force that acts tangentially to the path can produce radial deviations.

Consider a satellite orbiting the earth on a circular orbit. If there were no air drag, the attractive gravitational force ($F_g=-GMm/r$) and the repulsive centrifugal force ($F_c=mr\dot{\phi}^2$) perfectly balance. Hence, the radius $r$ stays constant, giving a circular orbit.

Now, add some air drag. The drag force is acting horizontally. That means that the angular speed ($\dot{\phi}$), and hence the centrifugal force ($F_c=mr\dot{\phi}^2$) will decrease. This destroys the balance between centrifugal force and gravitational force, so that there is now a small net force pointing down towards the earth.

Answer 2

My theory is that the work done by the drag force is insignificant in comparison to the whole energy of the orbiter...it essentially pushes it to a lower orbit while mostly conserving the total energy.

The work done by drag force is significant, because it decreases net energy of the orbiting body, as it spirals down to lower and lower orbits.

At first, when the drag force is perpendicular to gravity, it slows down the satellite, so that the satellite has lower speed than necessary for staying on the circular orbit. Thus it starts approaching the center of attraction; its velocity then has non-zero component towards the center of the Earth.

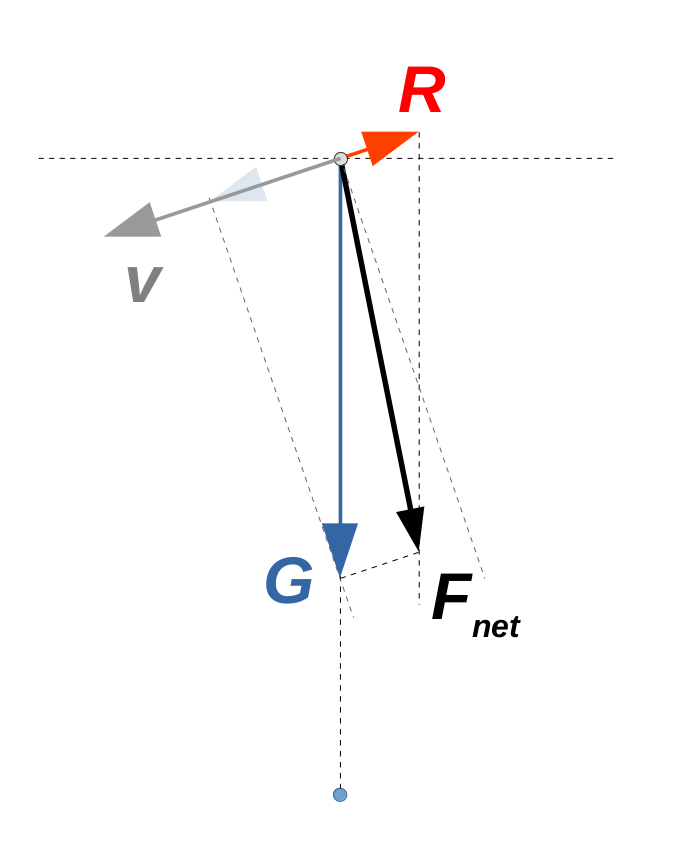
Then, further fall and increase of speed is due to work of gravity force - see the figure. $\mathbf v$ is velocity, $\mathbf R$ is the drag force (opposing velocity), and $\mathbf G$ is force of gravity. When velocity is tilted towards the center of attraction, and if the drag is low enough, the component of gravity force in direction of velocity is actually greater than the drag, so the satellite accelerates.

Without a drag force, this acceleration could be happening only on part of an elliptical orbit, and then the satellite would get enough tangential speed to start moving away from the center. With drag, this reversal of radial motion can happen, but the satellite won't reach the original height, but only a lower height. So after each orbit, the satellite gets on lower and lower (almost circular) orbit.

Answer 3

Here's a rough mathematical description:

Imagine a small body of mass $m$ orbiting at a speed $v$ around a large body of mass $M$. The radius of the circular (frictionless) orbit is given by equating the gravitational force to the centripetal force: $GMm/r^2 = mv^2/r$. This gives $r = v^2/GM$. The kinetic energy of the body is $\frac{1}{2}GMm/r$.

Now say friction acts on the object such that the tangential velocity reduces by an amount $\Delta v$, which is much smaller than $v$. This causes two effects: a decrease in the the radius and an increase in the (inwards) radial velocity. The new radius can be found from the old one:

\begin{equation} r = v^2/GM \implies \Delta r = 2 v \Delta v /GM. \end{equation}

Note the radius decreases because $\Delta v$ is negative. What is the acquired radial velocity? If we assume work done by friction is negligible (as you correctly suspected in the question, this is true), then by energy conservation we must have

$$\mathrm{PE}_i + \mathrm{KE}_i = \mathrm{PE}_f + \mathrm{KE}_f$$ $$ -\frac{GMm}{r} + \frac{1}{2}\frac{GMm}{r} = -\frac{GMm}{r-\Delta r} + \frac{1}{2}m (v_t^2 + v_r^2)$$

On substituting $\Delta r$ from Eq 1, and $v_t = v - \Delta v$, you will find an expression for $v_r$ in terms of $v$ and $\Delta v$. You should see that the magnitude of the velocity $\sqrt{v_t^2 + v_r^2}$ is larger than the initial velocity $v$ (I haven't checked this explicitly but this should be the case).

Physically, friction lowers tangential velocity, which results in the orbiting body falling inwards (it needs a certain velocity to maintain a circular orbit). This inward fall results in the radial velocity increase. This increase is more than the tangential decrease, causing a net increase in speed.