When I fill the higher end of a hose till its overflowing. I can keep the lower end of the hose bout half a foot below it without water flowing out of it. The middle of the hose is all beneath the higher hose end. The crinks in the hose seem minor, especially since raising one end of the hose up and down shows changes in the water level of the other end. Why is the water level in one end of the hose able to be higher than the other end? The hose has a diameter of bout 1 inch. Doing some napkin calculations shows that surface tension is bout 2 orders of magnitude lower than the pressure difference arising from the height difference in water levels. Which seems to rule that out. The hose is a normal garden hose.
Add a comment
|
3 Answers
$\begingroup$
$\endgroup$
1
In addition to the explanation of @niels nielsen, the presence of vertical loops or humps in the hose can present a problem.
If any of these vertical loops have the top of the loop filled with air, then the levels at the two ends need not be the same. You describe filling the hose by pouring water in one end of the hose. This method almost guarantees bubbles in the hose.
The whole basis of a water level is that the hydrostatic pressure changes with depth in the liquid. Introducing places where you go up through water, and then down through air trapped in the loop, throws the whole process off.
A better way to fill the water level hose is to put one end in a bucket of water, then draw some water by suction to the lower end. Allow the syphon to run for a while, and move the hose around to eliminate humps, and get any bubbles moving.
When I use such a device to measure the slope in my driveway, I use clear plastic tubing, just so I can see any bubbles. (And I can see the level dropping and gradually slowing down to its final position.)
If you have a length of tubing, filled with bubble-free water at rest, the water levels at both ends will be the same.
-
$\begingroup$ Why would air pockets affect anything? If you go down through air won't gravity just push the water down, thereby pushing the air out of the way? And if you go up through air, that's the same as if you had two open containers connected by a tube. $\endgroup$– roobeeCommented Jul 20, 2020 at 14:11
$\begingroup$
$\endgroup$
for a long hose, the inertia of all the water in it slows down the hose's response to changes in the level of its open ends. It takes ~seconds for the water to start moving, and once it starts moving, it will persist for ~seconds more even if you immediately readjust the end heights of the hose in such a manner as to stop it.
So the question is, for how long can the ends of the hose be offset before flow is established? 1, 2, 5, 10, 20, or 50 seconds?
answered Jul 20, 2020 at 4:52
$\begingroup$
$\endgroup$
While the question didn't mention trapped air, that is the probable reason according to my experience. So, I agree with DJohnM. But why does air inside the hose result in a height difference between the hose ends?
Air is compressible, and its volume changes inversely with the pressure.
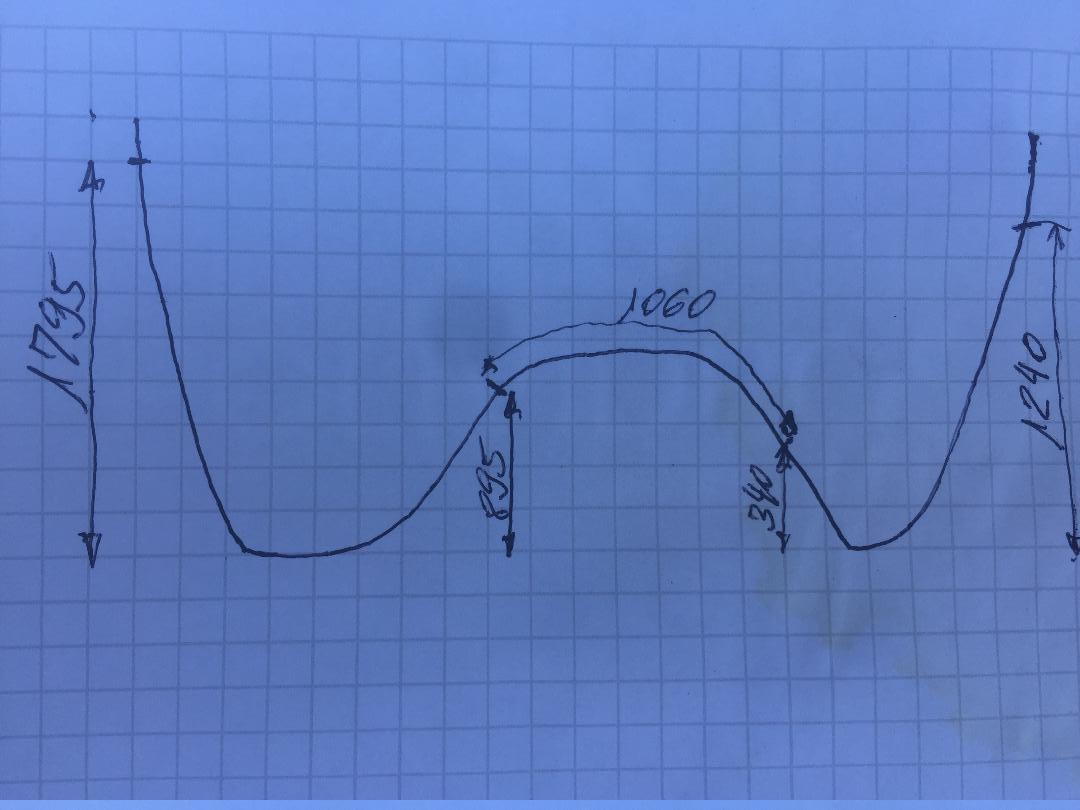
In the picture below, a transparent hose was filled with water from the left with the help of a funnel. It is suspended by a holder in the middle part and touches the ground before goes up at both sides. After pouring the water, an equilibrium was reached with a length of $1060mm$ of air trapped in the middle region, and a lower height of water at the right end, compared to the left one.
The difference of $900mm$ of the levels at left and right corresponds to the pressure of the air inside. Knowing that $10330mm$ of height corresponds to 1 atm, there is a pressure of $1+\frac{900}{10330} = 1,087$atm there. The volume is contracted accordingly, what means that the length of the air trapped region would be $L = 1060*1,087 = 1152$m at the atmospheric pressure.
Note that because water is incompressible, that equilibrium solution with different heights at the ends is not possible without trapped air inside. But with air in the middle that is the equilibrium configuration. If the air region was more at right, the right water column would be bigger than the left one, pushing that region to left.
answered Mar 18, 2023 at 1:20