Lets define some variables first. Lets say that the length of the column of air that you trap in the tube between the water level and your thumb is $h_0$ and it is initially at the same pressure as the surrounding air which we will call $P_0$. Lets also define the cross-sectional area of the tube to be $A$. As you draw the tube out of the water, the water level in the tube will rise above the surrounding water level, and the pressure at the bottom of this column of water will be given by
$$
P_0=P_t+\rho g h_w,
$$
where $P_t$ is the pressure of the volume of air trapped by your thumb, $\rho$ is the mass density of the water, $g$ is the gravitational acceleration at the surface of the Earth, and $h_w$ is the height difference between the water in the tube and the surrounding water. The second term on the right hand side is a standard equation from fluid statics. You can see from this already that the pressure can not be the same at the top of the tube anymore, as you suspected.
We can use the ideal gas law to rewrite the first term on the right hand side
$$
P_tV_t=P_0V_0\qquad\Rightarrow\qquad P_t=P_0\frac{V_0}{V_t}
$$
Now the volume of air at the top of the tube before you start to draw it up is given by $V_0=Ah_0$, but as you draw it up the pressure and volume will change to $V_t=Ah_t$. So, putting all of this information into the first equation we get
$$
P_0=P_0\frac{h_0}{h_t}+\rho g h_w
$$
The final thing we need to take care of is replacing $h_t$ with something we actually know. Namely, the height we draw the tube up to, which we will call $h_s$ and is given by $h_s=h_t+h_w$. Sticking this into the above equation yields
$$
P_0=P_0\frac{h_0}{(h_s-h_w)}+\rho g h_w
$$
Solving this equation for $h_w$ gives
$$
h_w=\frac{1}{2\rho g}\left(P_0+\rho g h_s+\sqrt{P_0^2+(\rho gh_s)^2+P_0\rho g(4h_0-h_s)}\right).
$$
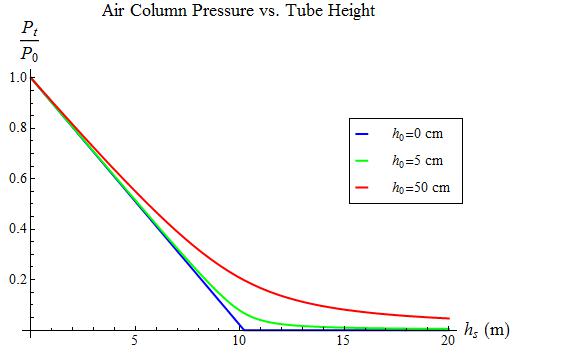
I've plotted this equation below for differing values of $h_0$. Notice that there is a maximum height to which you can draw water with this method, this is the height at which the pressure in the tube reaches zero.

Finally, since your question was actually about the pressure in the tube, we can rearrange the above equations to solve for the pressure in the tube under your thumb.
$$
P_t=P_0-\rho gh_w=\frac{1}{2}\left(P_0-\rho g h_s-\sqrt{P_0^2+(\rho gh_s)^2+P_0\rho g(4h_0-h_s)}\right).
$$
This equation is plotted below for a number of different values of $h_0$.