If spacetime itself is expanding, how could we ever tell?

Question

If space is the measured distance between 2 objects, then saying the space expanded is nonsensical unless we have a measuring stick outside of the space fabric to measure the expansion. 2 objects moving away from themselves isn't the same as space expanding.

What measuring stick is outside space? Doppler redshift shows the relative speed of a star in relationship to us - but would have no effect on a space expansion.

Edit: There seems to have been some confusion (very possibly on my part) on the nature of my question. I'm not talking about an explosion-type situation where matter in the universe is moving apart (with nuclear forces holding stars/planets and gravitation forces holding solar systems, and star clusters together). This is intuitive. I'm talking about statements made by physicists that "space itself" is expanding. An excerpt from a scientific American article: "the spacetime containing matter cannot remain stationary and must either expand or contract" https://www.scientificamerican.com/article/where-is-the-universe-exp/

Edit after related question presentation: So according to the answers on that related question, we should be able to measure exactly that extra energy spent holding matter together against the accelerating space-time expansion. Would radioactive decay would be speeding up too? Would it get easier and easier to split an atom? So thats how we'd measure spacetime expansion?

Answer 1

If spacetime itself is expanding, how could we ever tell?

It is a common question, if everything is expanding what gives the scale? The answer: by measurements of the movement of cosmic masses.

The simplest answer is seen in the analogy of the raisin bread, the raisin being matter, the dough being space expanding.

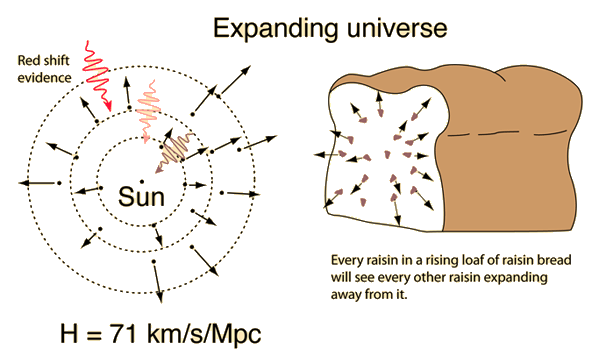
Why are the raisins not expanding as the loaf of bread expands, but the space between them grows? Because the raisins are held by chemical bonds, electric and magnetic forces much stronger than the expansion, and remain whole because they do not participate in the chemistry of the dough.

This is a simple analogy. A raisin (if conscious) deduces that the bread loaf is expanding because all other raisins are moving away from each other. It is not an explosion, because there is no center.that can be fitted with three dimensional kinematics.

What gives a measuring stick for the raisins to measure expansion is the geometric stability of the raisins themselves :they are made of solid matter held together by electromagnetic and strong forces and keep the size, because the effective force involved in the expansion of the loaf is by orders of magnitude smaller than strong and electromagnetic forces composing the raisin. The raisins keep their size so can deduce the expansion of the loaf because they have different chemical properties than the dough. The measuring stick is their size.

Doppler redshift shows the relative speed of a star in relationship to us - but would have no effect on a space expansion.

Doppler redshift from all directions about us, see the left image above, showed that every thing is receding from everything else. As this happens in explosions, the Big Bang cosmological model came into effect. That the atoms and molecules of matter do not expand with the expansion of the "bang" is due to the strong, electromagnetic forces holding matter together against this explosion allowing for measuring sticks in the size of stars; even the gravitational forces holding the galaxies together. The effective expansion force is very much weaker and thus the measuring sticks show a "big Bang".

It is interesting to read Hubbles biography. His observations coincided with the time General Relativity became dominant in the field of cosmology:

Starting with Albert Einstein’s 1917 paper “Kosmologische Betrachtungen zur Allgemeinen Relativitätstheorien” (“Cosmological Considerations on the General Theory of Relativity”), a number of physicists, mathematicians, and astronomers had applied general relativity to the large-scale properties of the universe. The redshift-distance relation established by Hubble and Humason was quickly meshed by various theoreticians with the general relativity-based theory of an expanding universe. The result was that by the mid-1930s the redshift-distance relationship was generally interpreted as a velocity-distance relationship such that the spectral shifts of the galaxies were a consequence of their motions. But Hubble throughout his career resisted the definite identification of the redshifts as velocity shifts.

The concept of four dimensional space time being directly connected with the masses and energy in the universe and all the variations of modelling the universe comes with General Relativity and its mathematics. The Big Bang model is a GR model and is the mainstream cosmological model at present. It models the redshifts as expansion of four dimensional space and is not falsified at present, as it is extended to fit any new observations.

The answer to:

I'm talking about statements made by physicists that "space itself" is expanding.

is that we have a General Relativity mathematical model that fits all the data and observations at present, including the redshifts, by the hypothesis that four dimensional space is expanding from an original four dimensional "region". The expansion is measurable because matter is held together much more strongly than the expansion rate.

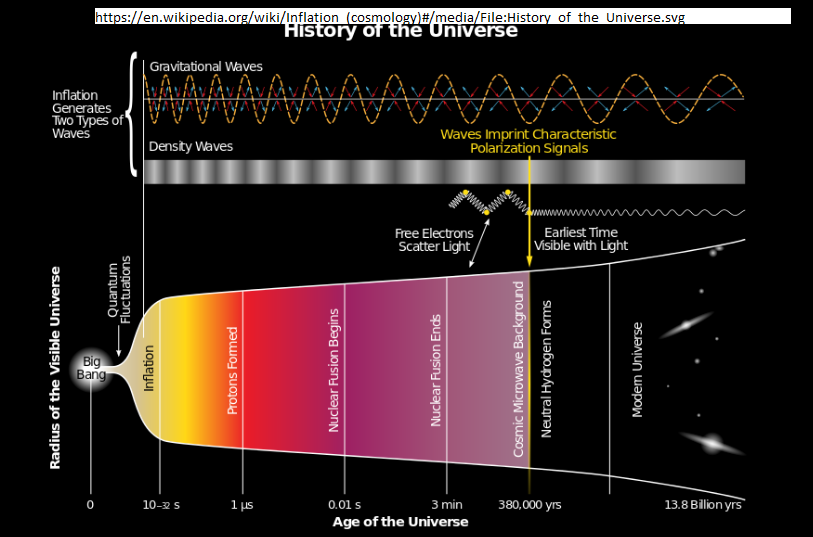
This shows a two dimensional cut of the four dimensions, time being the x axis and space the y. It is a fit to the observations, including the Hubble velocity. The other two dimensions not shown have the curvature of space too. It is the mathematical equations that give these plots, and they depend on general relativity.

We can tell, because the G.R. model fits all the data we have up to now.

See this extensive answer here.

Answer 2

NB: The nature of the question has changed since I placed this answer. This answer does not address the current version of the question. This answer addresses how we distinguish expansion of space from a model where galaxies move away from us through space (which is not straightforward).

At the moment it is Hubble's law, some indirect measurements and a bit of philosophy.

We observe that galaxies appear to move away from us, in an isotropic fashion, at a rate that is proportional to their distance from us.

Whilst one could argue that we are at (or near) the centre of this very uniform expansion it begs the question as to why Hubble's law should exist and why the universe appears isotropic to us, but wouldn't from a different position in the universe. The simplest explanation is that General Relativity applies (as we observe in a number of other cases) and we live in an expanding universe - this then means we do not need to occupy some privileged position in the universe (an erroneous assumption that has proved wrong every other time it has been made).

In such a universe, the redshift of distant galaxies is not caused by relative motion, but by the expansion of space. At high redshifts, these phenomena become distinct in that the relationship between "velocity" and redshift is different, for instance allowing "faster than light" (apparent) speeds.

So basically at present, expansion fits the facts (far) better and more simply than any of the alternatives.

A further piece of indirect evidence comes from a careful analysis of the physical conditions of gas at high redshifts, illuminated by background quasars and subtle alterations to the cosmic microwave background (CMB) spectrum, caused by the Sunyaev-Zel'dovich effect, towards galaxy clusters at low redshifts. Both of these methods give the temperature of the CMB at those locations.

In the expanding universe model, the temperature should increase as $1+z$, where $z$ is the redshift. If one instead has a non-expanding universe, and explain the CMB as due to some expanding shell of material, then the average temperature wouldn't change for distant galaxies unless the shell gas has been uniformly cooling by an amount that just happens to agree with redshift of that galaxy.

Avgoustidis et al. (2015) review the evidence for the temperature evolution of the CMB and conclude that it agrees with an adiabatic expansion to better than 1%.

Direct evidence for the expansion is on the horizon though. In an expanding universe, the speed at which galaxies move away from us can change slowly with time (and with distance) by of order 10 cm/s per year, despite their being no force on them. This is known as the redshift drift. There are plans to measure this tiny effect with the European Extremely Large Telescope over the course of a decade.

Answer 3

Take the model that the space has a net positive curvature so that it is like a 3-sphere. Then take a cross-section through that 3-sphere, and you get a 2-sphere, which we can picture as the surface (N.B. the surface only, not the interior) of a spherical balloon located in an abstract Euclidean space.

The statement that space is expanding corresponds to the statement that this sphere is getting larger. What it means is that if you take an ordinary steel ruler, and lay it down on the surface of the sphere, and another one next to it etc., until you go all the way around, so as to measure the circumference, then if you did the same experiment again after some time has passed, then you will need more of these steel rulers. The reason is that the space expanded but the steel rulers did not.

To do such an experiment in our universe, the rulers would have to be millions of lightyears long, and you would have to wait a billion years to get an appreciable effect, but these are mere technical details....

Finally, I used the circumference of a closed universe to make the point. One could instead measure other properties, such as the distances between a large enough collection of galaxies. Then the observations can be made for a spatially flat or open universe too. But even such universes can also have a finite circumference if they have an unusual topology (though in that case they would not be isotropic).

Answer 4

The answer by anna v is already great, but it might be worth to elaborate on the following point. In the latest edit (v3), OP has added the following sentence:

If "space itself" is expanding, then the sizes of the atoms, planets, stars would also be expanding along with the space between them, which would make us oblivious to the "space itself" expanding.

This is wrong. Why would atoms, planets or stars expand along with the space between them? The most likely distance between the proton and the electron in a Hydrogen atom is given by the Bohr radius $a_0 = \frac{\hbar}{m_e c \alpha} \approx 5 \cdot 10^{-11} m$, it is fixed by the electromagnetic force and none of these elementary constants are changing over time.

The expanding space "inside the atom" (assuming general relativity remains valid here) does introduce a slight "drift", pulling proton and electron apart. The speed of this drift can be approximated by calculating the product of the Bohr radius and the Hubble constant, which gives a speed of approximately $10^{-28} m/s$ - way too slow to have any effect. (This is where the raisin analogy of the other answers comes into play.)

Similar considerations apply to planets and stars.

Answer 5

You could say that the closest galaxies are accelerating away from us, and the next ones are accelerating even faster, and the next ones faster still, and so on that the galaxies very far away are moving away even faster than the speed of light (well, we can only assume, because and the universe is not old enough for us to see those galaxies accelerating to FTL speed) so close to the speed of light they would be invisible.

The problem with this model is the that it would put us squarely on the center of the universe, which would be quite unlikely. It seems much more likely that, symmetrically, every galaxy sees every other accelerating away just like we see it (on average). Add to it that we expect that nothing can accelerate to FTL speed, and you get that the most likely explanation is that space itself expanding is. The simplest model that fits that scenario -- where there is no preferred center to the outwards accelerating galaxies -- is to consider that the distances is increasing everywhere and in every direction, what we summarize as "the universe is expanding".

EDIT: as Rob Jeffries comment implied, the FTL argument doesn't really makes sense, so I struck it out.

Answer 6

Yes spacetime itself is expanding, and even on the smallest scales. It is the effect of this expansion, that is almost negligible on the smallest scales (and experimentally very hard to measure), at the size of atoms for example.

Why does space expansion not expand matter?

You are basically asking how we know that it is not just the objects that are getting farther from each other (like in the famous raisinbread example), but it is the very fabric of spacetime itself that is expanding.

The answer to your question is cosmological redshift. The very quanta of light, photons, as they travel through the expanding spacetime fabric, are getting stretched, because they exist in a fabric that is being stretched, that is expanding itself.

This is how beautifully the quantum object, the photon and its wavelength shows its wave nature when traveling inside a expanding fabric, gets stretched itself, giving us the very proof.

So if you could tie two objects together with a string many light years long, to keep them at rest relative to each other, there really would be a tension in that string. That tension arises because you are forcing the objects to accelerate away from the geodesics they would otherwise follow, and it arises in the same way as the tension in the string if you suspend an object in Earth's gravity.

On the expansion of space on small distances