What does it mean for a resistor to cause a "potential drop"? This means that the potential of an electron after going through a resistor is lower than the potential of the electron before it went through the resistor. Why is this the case?
First, let us go over what electric potential means. The electric potential of an electron is defined as the energy that would be needed in order to bring this electron to its present location from a distance of infinity. This means an electron has a higher electric potential when it is close to a large amount of other electrons (it would take more energy to overcome the repulsive forces of all these electrons in order to bring our electron to them).
Now, remember, we're trying to explain why electrons that have not yet entered the resistor have a higher potential than electrons that have exited it. It turns out that the reason is that electrons end up being more concentrated on the side of the resistor that they are entering, and less concentrated on the side of the resister that they are exiting. Let's look at why this happens.
Let's take a simple circuit consisting of a battery, a wire, and a resistor. Now, we know the resistor is made of material that is less conductive than the wire, so electrons aren't able to move as quickly in the resistor as they are in the wire.
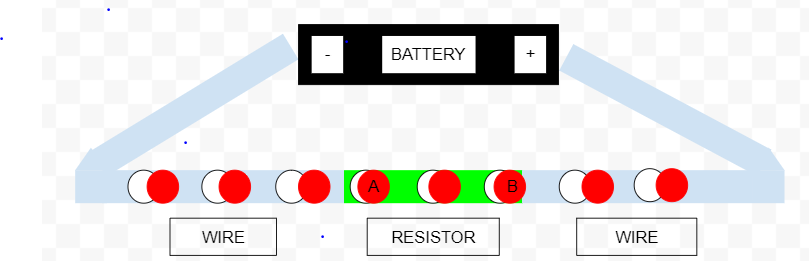
In the diagram above, the white circles represent the electrons in the wire at their initial state (before the battery is connected). The red circles represent the electrons a moment after the battery is connected to the wire.
When the electron closest to the negative end of the battery moves, it causes the next electron to move as well (because of the repulsive forces between electrons). This creates a cascading effect.
However, electron A in the above diagram cannot move as much (in the same timeframe) as all of the electrons behind it. As a result, electron A will be closer to all the electrons behind it than it was before. Hence, it repels these electrons more strongly. This slightly cancels the repulsive force on these electrons due the negative end of the battery, causing them to slow down.
Now, since the repulsive force an electron exerts on another electron decreases with distance, it is clear that those electrons that are closest to electron A will be slowed down the most and those that are furthest will be slowed down the least. Since the electrons that are in the front (meaning electron A and those that are slightly behind it) are the ones that are moving the most slowly, the electrons will start bunching up a bit near the entrance of the resistor.
Let's look at what happens on the other side of the resistor.
On the other side of the resistor, electron B cannot move as much as the electrons in front of it. Hence, electron B will be farther from these electrons than it was before, so its repulsive force on these electrons decreases. Therefore, these electrons also slow down, since the force with which B is pushing them away is now smaller.
However, the increase in distance from B to the electrons ahead of it will cause a greater decrease in repulsive force for electrons that are further from B. (This can be shown by basic math.) Hence, the electrons that are closest to electron B will slow down the LEAST. Hence, electrons will not bunch up nearly as much on the exiting side of the resistor as they will on the entering side.
Since electrons are more concentrated on the "entering side" of a resistor, an electron will have higher potential when entering the resistor and being among all these close-together electrons than upon exiting the resistor.
I hope that made sense!

