Tension in a ring rotating about its own axis

Question

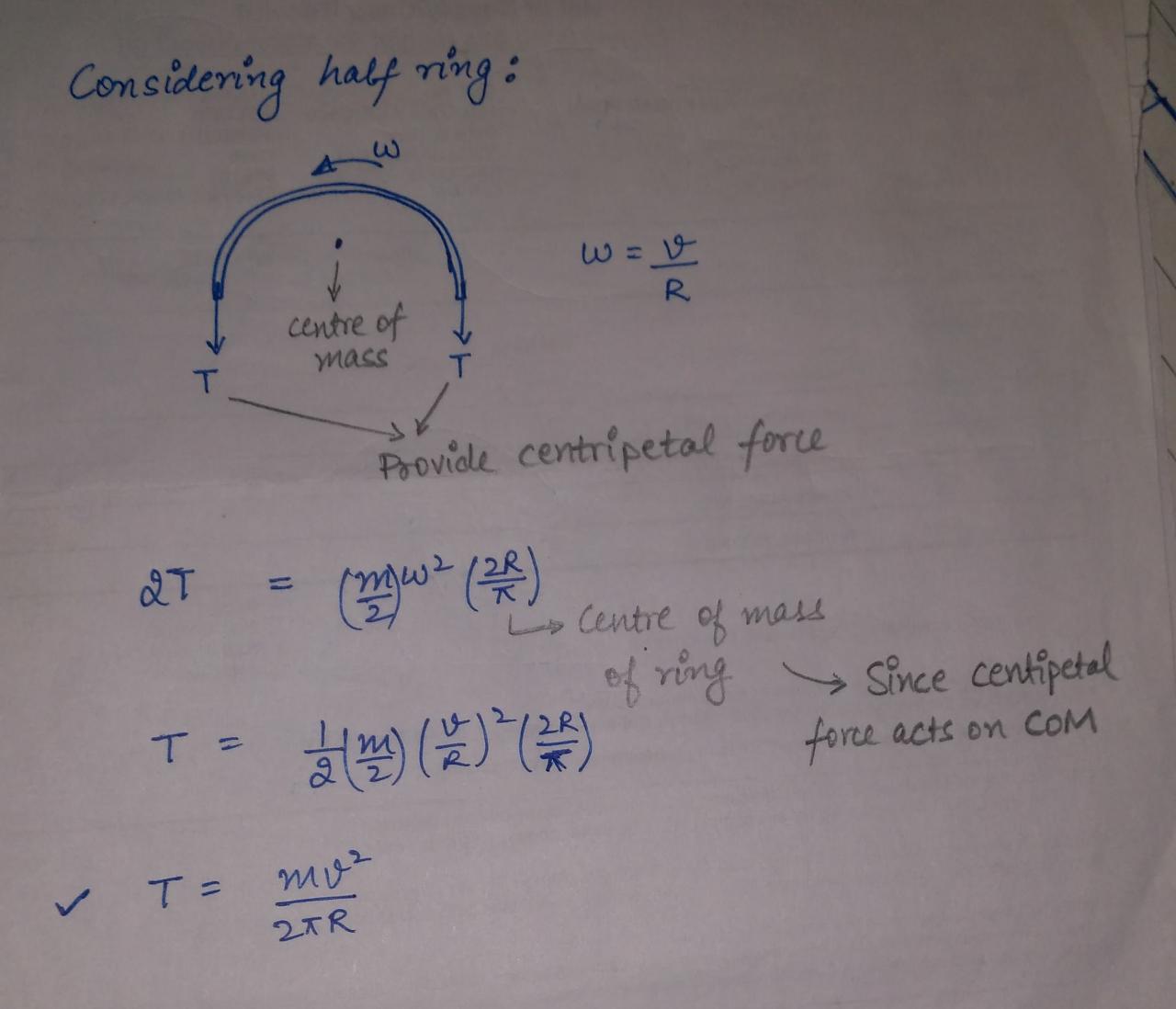
A ring of mass m and radius R is placed on a smooth horizontal table and is set rotating about its own axis in such a way that each part of the ring moves with a speed v. What is the tension in the ring?

Here is how I solved it:

My physics teacher said that this was correct. He discussed another solution in class, which was something like this:

I understood this, but here's my doubt: Why did he have to introduce ω (angular velocity)? Why does putting centre of mass with ω work but not with v (velocity)? I get a wrong answer this way:

What's the flaw here?

Answer 1

For your teacher's solution, you need to use the velocity of the center of mass. The easiest way to do that is to introduce the angular frequency:
We know that the ring spins at velocity $v$, therefore it has to go around one turn in time $T=2\pi R/v$. But the center of mass of the half ring must also go one rotation in the same time $T$, so therefore its velocity must be $v_{cm}=2\pi R_{cm}/T=v R_{cm}/R = 2v/\pi$. Introducing $\omega$ is just a shorter way of doing the same thing.