How are branching ratios for the Higgs boson calculated?

Question

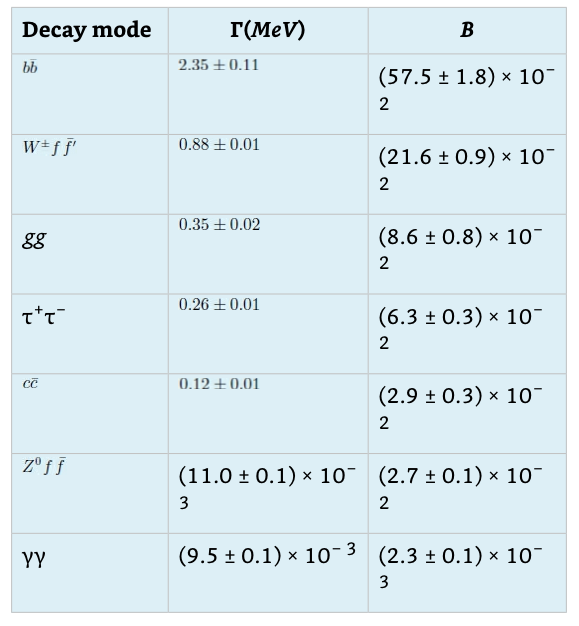
I'm somewhat familiar with how to calculate branching ratios. I know that they are calculated as the ratio between the decay width of a specific decay process and the total decay width of the particle (as said in this stack exchange post). However, I'm not sure how people are getting the values for the various branching ratio of the Higgs boson. In my textbook I have this table which lists the decay widths and branching ratios for the Higgs boson processes:

I was trying to calculate the branching ratio of the four-lepton decays: $$BR(H^0 \to l^+ + l^- + l^{'+} + l^{'-})$$

I used the values on this table and for the decay width of the four-lepton decay I used the decay mode width for $H^0 \to Z^0 f \bar f$. The value I got was $0.00276$. From what I've read the branching ratio for this specific decay is about $0.02\%$, so my answer is not correct. What are the actual values used for the calculation of the four-lepton decay? Are there more than on this table?

Answer 1

So we have that (see the CERNYellowReport) $$\text{BR}(h\to\ell\ell\ell\ell)\approx 2.7\times 10^{-4} \quad\text{for} \quad\ell=e,\mu,\tau$$ This is summed over all combinations of lepton flavors.

You found from your book, $$\text{BR}(h\to Z f \bar f)\approx 2.7\times 10^{-2}$$ $$\text{BR}(Z\to\ell\ell)\approx 0.1 \quad\text{for}\quad\ell=e,\mu,\tau$$ You then tried to find $$\text{BR}(h\to\ell\ell\ell\ell) = \text{BR}(h\to Z f \bar f) \times \text{BR}(Z\to\ell\ell) = 2.7\times 10^{-3}$$ This was the wrong answer, unfortunately.

I find the notation in your book peculiar. I would rather write $$\text{BR}(h\to Z Z)\approx 2.7\times 10^{-2}$$ which appears in the CERNYellowReport. We can indeed then do $$\text{BR}(h\to\ell\ell\ell\ell) \approx \text{BR}(h\to Z Z) \times \text{BR}(Z\to\ell\ell)^2 = 2.7\times 10^{-4}$$ You missed a second factor of $\text{BR}(Z\to\ell\ell)$, required to enforce the fact that the $Z$ decayed to leptons and not quarks or neutrinos.

Note that multiplying the branching ratios like this is an approximation - in particular, a narrow-width approximation in which we assume that the intermediate $Z$ bosons are on-shell. It seems to have worked reasonably well here, but in general narrow width approximations like this could produce unreliable results and destroy information about spin correlations.

See this thesis for a detailed calculation of this branching ratio beyond leading order.

Answer 2

Each calculation in this table involves evaluating the corresponding feynman diagram integral, not values from tables. Feynman diagrams are the recipe for setting up the integrals necessary to be calculated for finding widths and crossections in particle physics.

Here is a paper with Feynman diagrams for Higgs decays.

To get an idea of the complexity of the calculations just for the Higgs to three particles, not four, :

And this is only the first order to the perturbative expansion for the process. The more orders calculated the more accurate the result to be compared with experiment.

All the branching ratios in the table were calculated using the Feynman diagrams implied in the standard model of particle physics.