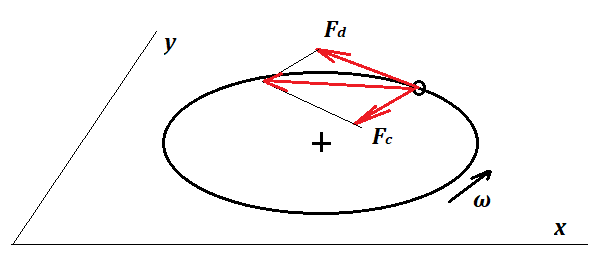
Let's assume a bead of mass $m$ is moving on a circular trajectory in a fluid, and that the typical velocity, the radius of the bead $a$ and the viscoisity of the fluid $\eta$ are such that we are in a regime with $Re\ll1$. What are the driving forces necessary to sustain uniform circular motion with radius $R$ and angular velocity $\omega$?
The derivative of the velocity vector can be written as
$$\frac{d\vec{v}}{dt}=\frac{d\left(\omega R\hat{u}_{\theta}\right)}{dt}=\frac{d\omega}{dt}\hat{u}_{\theta}+\frac{d\hat{u}_{\theta}}{dt}\omega=F_{\theta}\hat{u}_{\theta}(t)+F_{R}\hat{u}_{R}(t)$$
where $\hat{u}_{\theta}$ is the tangent vector at an angle $\theta$.
In order to make the first term of the RHS vanish, I write the force balance tangentially to the circle, in which I have an unknown driving force and the hydrodynamic drag:
$$ F_{\theta}=F_{\theta}^{(driving)}+F_{\theta}^{(drag)}=0$$
In this regime I can write
$$F_{\theta}^{(drag)}=-6\pi\eta av=-6\pi\eta a\ \omega R$$
$$\Longrightarrow F_{\theta}^{(driving)}=6\pi\eta aR\ \omega$$
and this gives me the tangential component of the driving force needed.
What about the normal component? In this regime, does $F_{R}=-\omega^{2}R$ make any sense? Does the hydrodynamic drag have a component along $\hat{u}_{R}(t)$?
EDIT
One could expect a relationship of the type $F_{R}=-m\omega^{2}R$ to be valid in this context, without any dependence on the hydrodynamic drag. However, this suggests that the inertia of the bead (through its mass) plays a role despite the fact that the inertia of the fluid (via the low $Re$ approximation) doesn't. Is this correct? Also, at low $Re$, torques are linear in angular velocities (is this valid in this case as well? Or only for the intrinsic rotation of an object around an internal axis?)