0
$\begingroup$

I have a question on finding the horizontal velocity component of a rotating bar in a (periodic) circular motion. Consider a bar rotating with angular motion: $$ \theta(t) = A \sin (kt) $$

where $A$ is amplitude, $k$ is a constant, and $t$ is time.

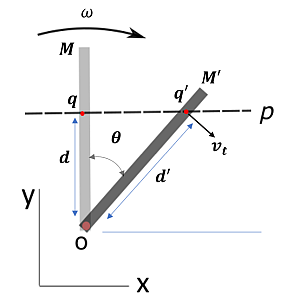
rotating bar M

The bar rotates from initial position $M$ to $M'$ with angular velocity $\omega = \dot{\theta}$. Let's consider a horizontal axis $p$ intersecting the rotating bar at a fixed distance $d$. At $M'$, the transverse velocity is given as: $$ v_t = \omega d' $$

where $d' = d / \cos \theta$.

Here, to find the horizontal velocity component $v_x$ of the bar at M', I have expressed it as (including time derivative of $\theta$):

$$ v_x = v_t \cos \theta $$ $$ v_x = k A \cos (kt) (d/\cos\theta) \cos\theta $$ $$ v_x = k A d \cos (kt) $$

To verify this, I tried a second method by defining the displacement of $q$ along $p$ axis as following:

$$ s = d \tan \theta $$

and performs time derivative of s to get the horizontal velocity $v_x'$ as followings:

$$ v_x' = \frac{ds}{dt} = k A d \cos(kt) [\tan^2(\theta) + 1] $$

The results are different by a factor of $(1+\tan^2(\theta))$. Is there a missing component in the derivation of $v_x$ ?

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ In your second method remember that $d$ and $\theta$ are both functions of time, so $\frac{ds}{dt} = \frac{dd}{dt}\tan \theta + d(1+\tan^2 \theta)\frac{d\theta}{dt}$. I think you have omitted the first term. $\endgroup$
    – gandalf61
    Commented Apr 10, 2019 at 10:32
  • $\begingroup$ @gandalf61 I think I forgot to mention that $d$ is a fixed distance while $d'$ is not. $\endgroup$
    – Karsun
    Commented Apr 10, 2019 at 10:50
  • 1
    $\begingroup$ If $d$ is fixed then $q'$ has a radial veloctiy $v_r$ as well as a tangential velocity $v_t$. So it is no longer true that $v_x=v_t\cos\theta$. Instead $v_x=v_t\cos\theta + v_r\sin\theta$ and your second method gives the correct answer. $\endgroup$
    – gandalf61
    Commented Apr 10, 2019 at 11:11
  • $\begingroup$ @gandalf61 thanks, your intuition is right. $\endgroup$
    – Karsun
    Commented Apr 10, 2019 at 12:56

1 Answer 1

Reset to default
0
$\begingroup$

In your first version you assume $d'$ is constant when writing $v_t=\omega d'$. The motion of $q'$ is a the compound motion of the point rotating with the rod, and also the point sliding further up on the rod. By taking the horizontal component of $v_t$ you ignore the contribution of sliding up on the rod. So your second method is correct.

Alternatively, you could aso say $$ v_x = v_{t,x} + v_{r,x} = kAd \cos kt + \frac{d (d')}{dt} \sin\theta = kAd \cos kt + \frac{\sin^2\theta}{\cos^2\theta} kAd \cos kt = kAd \cos(kt)(1 + \tan^2 \theta)$$ where $v_t$ and $v_r$ are the tangential and radial velocities, and the $x$ subscript means their $x$-component.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.