Force and center of mass acceleration

Question

https://i.sstatic.net/3w7Mt.png

I have a rod with mass $m$. Above are two ways I can apply a perpendicular force to the rod. Since the mass of the rod is uniform, its center of mass is in the center of the rod.

Case 1: It is obvious that the acceleration of the center of mass is equal to $F/m$.

Case 2:

1. If the acceleration at the top of the rod still $F/m$? If not, I guess $m$ is different here. Why is $m$ different here? What is it?

2. I am told that the acceleration in the center of mass is $F/m$, even if the force is applied at the top of the rod. I cannot see why. Can anyone explain to me in an intuitive way why that is?

I found some questions like Force applied off center on an object, What happens when a force is not applied on the centre of the mass? and What does it mean for a force to "go through the center of mass"? but I could not find an intuitive explanation about the reason for this.

Answer 1

When the force is pressing the rod at the end, it causes rotation of the rod.

The rod locally acts like if it were a point-like object with the mass smaller then the mass of the rod: $m_{eff}=F/a_{end}$.

The same force $F$ causes a faster motion of the rod end, compared to the central force case, so the power $P=F.dx/dt$ is higher.

The center of mass accelerates slower then the rod end, by $a=F/m$, the same way as in the central case.

The extra work done by force $F$ due faster motion is invested in rotational kinetic energy $E_r=1/2.I.\omega^2$.

The key point is to honour all 3 fundamental conservation laws for energy, momentum and angular momentum.

Important thing to remember is, even a linearly moving object has nonzero angular momentum, if it is along a line not passing the coordinate system origin.

Answer 2

Can anyone explain to me in an intuitive way why that is?

It's not easy to say what you would consider intuitive and what not. I've made my guess and give an answer with no equations and with some statements I believe you could find intuitive. You'll have to pay a price, however. I'll make reference to several figures I have no time to draw and leave for you to reconstruct.

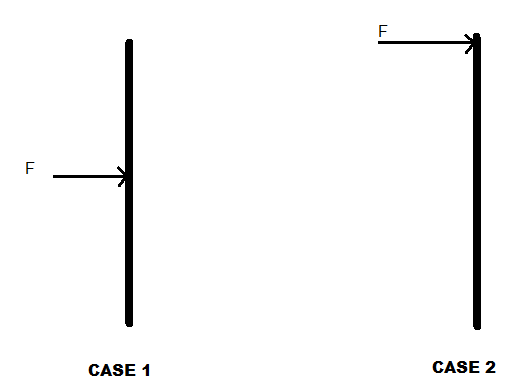
A slight change of notation. The force in case 1 I'll name $F_1$, the one in case 2 $F_2$. Other forces i'll define presently.

You agree that in case 1 the rod will simply accelerate with no rotation, and its com will have acceleration $F_1/m$. Your question is about what will happen in case 2.

1) Add to case 2 two further forces: $F_1$ and its opposite (applied at the same point) which I'll call $F_3$. I ask you to agree that

addition of two opposite forces applied in the same place has no effect.

2) Consider the system of three forces as composed of force $F_1$ alone plus the couple formed by $F_2$ and $F_3$. I make a further assumption:

the effect on rod of several forces is the geometrical (kinematical) composition of the separate effects of each one.

Since we know the effect of $F_1$ we are left to investigate the effect of the couple $(F_2,F_3)$.

3) Consider another force $F_4$ equal to $F_3$ but applied to the lower extreme of rod. I'm going to prove the following:

actions of couples $(F_2,F_3)$ and $(F_1,F_4)$ are identical.

To prove this consider another couple: $(F_3,F_5)$ where $F_5$ is directly opposite to $F_4$. So $(F_3,F_5)$ is globally opposite to $(F_1,F_4)$.

Look at the system formed by 4 forces: $(F_2,F_3,F_3,F_5)$ (force $F_3$ is doubled). It can be seen as formed by two subsystems: $(F_2,F_5)$ and $(F_3,F_3)$.

The effect of $(F_3,F_3)$ is known: an acceleration leftwards of magnitude $2F_3/m$. As to $(F_2,F_5)$ I ask you to accept another assumption

two forces euqal in magnitude and direction, applied in different points, have the same effect as a force of double magnitude applied in the midpoint.

So $(F_2,F_5)$ is equivalent to $2F_1$, which is opposite to $2F_3$. Then the total system $(F_2,F_3,F_3,F_5)$ has a null effect. But remember that it was formed of two couples: $(F_2,F_3)$ and $(F_3,F_5)$ and you see that both couples cancel each other. Since $(F_3,F_5)$ is opposite to $(F_1,F_4)$ we have shown that $(F_2,F_3)$ and $(F_1,F_4)$ are completely equivalent.

This is a non trivial result: we have shown that

translating a couple to a different position (leaving unaltered all parameters: magnitude and direction of forces and relative position of application points) leaves its effect unaltered.

4) Summarizig: $(F_2,F_3)$ and $(F_1,F_4)$ are equivalent. Then applying both at the same time will have twice the effect of each couple alone. But applying both is the same as applying one couple of double arm: $(F_2,F_4)$. What will its effect be? I can appeal to symmetry to state

a couple of forces symmetrically placed wrt to com causes no motion of it, but only an angular rotation around it.

Then we may conclude that the same happens if only one of couples $(F_2,F_3)$ and $(F_1,F_4)$ exists. Both keep com undisturbed causing an angular acceleration of rod around it. A not intuitive result!

5) And now we are at the end. We have seen that $F_2$ is equivalent to $F_1$ plus couple $(F_2,F_3)$. The former causes an acceleration of com, equal to $F_1/m$. The latter (the couple) has no effect on com but causes angular acceleration around it. Then this is the effect of $F_2$ alone, we were looking for.