Energy due to force acting on a rod

Question

Let us consider a rod of length $L$. We apply a force $F$ on this rod in a direction perpendicular to its length. There are two possible cases, we apply the force on the center of mass of the rod, or at some other point along its length. In the case where the point of contact is at the center of mass, there is no torque generated and the rod accelerates linearly. But when the force is applied at any other point on the rod, say on one of its ends, the center of mass will still accelerate with the same acceleration, but now there is rotational acceleration as well. In the first scenario the rod has translational energy only, and in the second case the rod has rotational as well as translational energy. So where did this energy come from?

Answer 1

When the force is applied at one of the ends, it results in linear acceleration of the center of mass plus angular acceleration about the center of mass. So in a given duration, the end to which it is applied travels a larger distance than the center of mass. Since the force is applied over a larger distance, more work is done.

Answer 2

But when the force is applied at any other point on the rod, say on one of its ends, the center of mass will still accelerate with the same acceleration

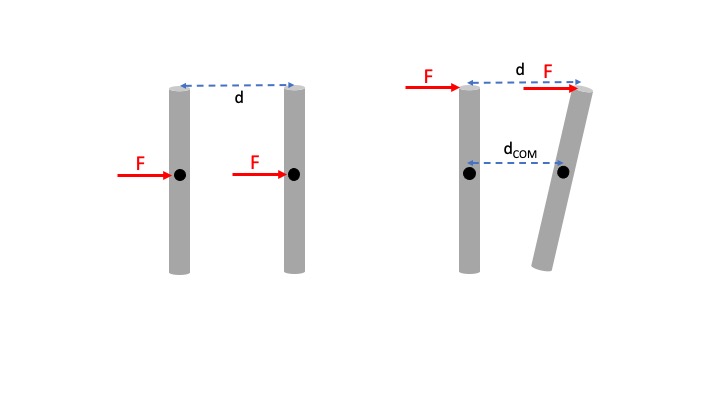
It will not have the same translational kinetic energy when the force is applied to the end as when the force is applied to the COM, for a given displacement of the point of application of the force. That’s because the displacement of the COM will be less when the force is applied to the end than when applied to the COM. See the figures below.

For both figures the work done by the force $F$ is the product of the the force and the displacement of its point of application. For both figures that work is $Fd$. Note however in the figure to the right the displacement $d_{COM}$ of the COM due to the force is less than the displacement $d$ of the point of application of the force. Therefore the increase in translational kinetic energy of the COM is less when the force is applied to the end than when it is applied to the COM for the same displacement of the point of application of the force.

Since the net work done on an object equals its total increase in kinetic energy, the decrease in translational kinetic energy when the force is moved from the COM to the end equals the rotational kinetic energy resulting from moving the force.

Hope this helps.

Answer 3

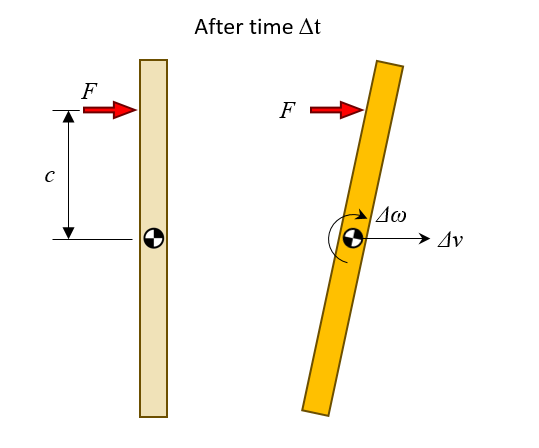
@Vincent has the correct answer, as the force travels a larger distance when the rod is rotating after the same time period $\Delta t$.

In the general case, the force is applied some distance $c$ from the center of mass.

The momentum after some time equals the impulse due to the force, both in the translational and rotational sense

$$\begin{aligned} p &= F \Delta t \\ L & = c F \Delta t \end{aligned}$$

and the resulting motion from the above momenta is

$$ \begin{aligned} \Delta v &= \tfrac{1}{m} p = \tfrac{F}{m} \Delta t \\ \Delta \omega & = \tfrac{1}{I} L = \tfrac{c F}{I} \Delta t \\ \end{aligned} $$

where $m$ is the rod's mass, and $I$ is the mass moment of inertia of the rod.

Now we can look at the kinetic energy of the rod after this time period.

$$ KE = \tfrac{1}{2} m \Delta v^2 + \tfrac{1}{2} I \Delta \omega^2 $$

$$ \boxed{ KE = \tfrac{1}{2} \left( \tfrac{1}{m} + \tfrac{c^2}{I} \right) F^2 \Delta t^2 }$$

As you can see, the larger $c$ is, the higher the $KE$ is the object is. Also note that the part in the parenthesis above is the inverse of the effective mass that the force feels.

$$ m_{\rm eff} = \frac{1} { \tfrac{1}{m} + \tfrac{c^2}{I} } $$

which reduces the further away the force is applied. The above is often called the reduced mass of the rod.