If a rod is on a frictionless plane, and a force is applied on one of it's end, will there be both, translation + rotation motion? Also, if only a single force is applied on a body that does not pass through Centre of Mass, will it always produce rotation + translation?
-
$\begingroup$ To answer your second question clearly, saying that the line of force "does not pass through the centre of mass" is not enough information. You need to break the force down into 2 components and then ask "does the force have a component that passes through the centre of mass. If so, there will be translation, if not, there will only be rotation. See my answer for a full explanation. $\endgroup$– JeneralJamesCommented Nov 30, 2016 at 9:52
-
$\begingroup$ Possible duplication of Division of energy in force on one end of stick or Force acting on a simple rigid body in space $\endgroup$– sammy gerbilCommented Nov 30, 2016 at 13:41
-
$\begingroup$ @JeneralJames Even if the force does not have a component passing through the center of mass, there will be translation. An off-center force perpendicular to the rod will still cause translation. $\endgroup$– CrimsonCommented Nov 30, 2016 at 15:22
-
2$\begingroup$ Possible duplicate of Do objects rotate around the torque vector or its center? $\endgroup$– John AlexiouCommented Sep 17, 2017 at 6:07
-
3$\begingroup$ Possible duplicate of Force acting on a simple rigid body in space $\endgroup$– stafusaCommented Sep 17, 2017 at 22:37
2 Answers
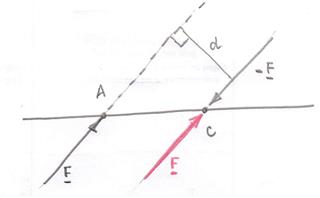
Suppose that you have a force $\vec F$ acting at a point $A$ on the rod as shown in the diagram below.
Add two forces of equal magnitude but opposite in direction whose line of action is parallel to the original force but with those forces acting at the centre of mas.
This results in a force $F$ (red) acting through the centre of mass which provides the translation acceleration of the centre of mass and two forces $\vec F$ and $-\vec F$ (grey) which constitute a clockwise couple of magnitude $Fd$ which will produce the angular acceleration of the rod.
So if the line of action of the applied force does not go through the centre of mass of the rod you will always get a translational acceleration and a rotational acceleration.
-
$\begingroup$ Thank you. I can see the explanation for rotational acceleration in your answer, but where the translation acceleration could be seen from here? $\endgroup$ Commented Dec 15, 2022 at 17:55
-
$\begingroup$ @DamirTenishev The grey forces produce the torque and hence the rotation and the red force which acts through the centre of mass produces the linear acceleration. $\endgroup$– FarcherCommented Dec 15, 2022 at 18:25
-
$\begingroup$ The exact question is, what makes you think that these grey forces won't balance this red force in linear acceleration term? Hint: if A is infinitely far from C we have exactly this: only rotation will occur. The question is how to prove that with a finite distance between A and C this won't happen. Another concern, with your explanation it sounds like there is no difference where to apply the force; the resulting linear force/acceleration will be the same. This sounds a little bit odd, life experience tells me that kick to center much more effective. $\endgroup$ Commented Dec 16, 2022 at 1:53
-
1$\begingroup$ life experience tells me that kick to center much more effective this is due to frictional forces being present. Look at Appendix 20A Chasles’s Theorem and note that many things in rotational dynamics are counterintuitive. $\endgroup$– FarcherCommented Dec 16, 2022 at 8:43
-
$\begingroup$ Thank you so much for the link, this is just what I needed. It seems that you are experienced in the subject, could you please consider my question Rotation of a system of bodies on an axis and the root cause for it Rotation of the systems of two bodies connected by a motor? Maybe you can help me to figure out what is a missing part in my understanding of rotation. $\endgroup$ Commented Dec 16, 2022 at 14:27
Simple answer is that in a frictionless environment force will act on the center of mass to produce a linear acceleration. If the force is acting away from the center of mass ie off set and therefor forming a moment ( m x distance from the center of mass) then the acceleration will be angular and centered on the center of mass ie the rod will rotate in the direction of the applied force, around its center of mass