Horizontal component of Coriolis force in an aircraft: compensating force with its wings angle

Question

I have the following problem:

"An aircraft is flying at 800 km/h in latitude 55◦ N. Find the angle through which it must tilt its wings to compensate for the horizontal component of the Coriolis force."

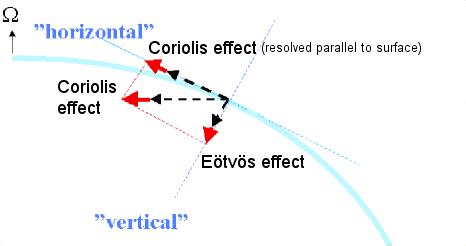
What I understand is that the aircraft follows the trajectory of the Earth's circle in latitude 55. So, since the Coriolis force (Fc) is the vectorial product -2w^r' (w is the angular momentum and r' the velocity in the rotating frame), it points inside or outside the Earth.

In the image, we have the first case:

So, if the aircraft is moving westward, we have the situation in the image, there is a component of the Coriolis Force trying to move the direction of the aircraft northward.

The problem is that I simply can't go on with these informations and find the angle (the answer is 0,155◦).

My specific question may be simpler than the concepts of the problem itself, but there it is: how can I compensate a force northwards only changing the angle of the flight? I can understand if there is a wind pushing the aircraft, I can find a direction to the velocity of the airplane that have one component that exactly vanishes the wind contribution. But how can I vanish a force? Is there a force that appears by changing the angle of the flight?

And more than that, if I change the angle, that wouldn't change also the direction and size of the Coriolis force and we should need another angle to compensate this new component of the Coriolis force?

(I'm not a native speaker, sorry for any language mistake)

Answer 1

On a level flight, you know that all the vertical forces must sum to zero (or you'd be accelerating upward or downward).

By banking the airplane, you can change the direction of the force from the wings. When flying straight, all force is vertical. When banking, a component of the force is vertical and a component is horizontal. The relative sizes of the components depend on the bank angle.

When considering the Coriolis force, you need the wing to continue to supply a vertical force equal to the vertical force when not banked and a horizontal force of some amount. That can only happen at some particular angle.

Answer 2

OK, I just worked this out. I assume the airplane is heading due north at 800 km/h, and it is at latitude 55N. I get that it has to bank left at an angle of 0.0776 degrees. Let me outline the calculation:

Let $a$ be latitude angle north of equator, in radians.
Eastward velocity $V$ of the equator is $464.6 m/s$ (assuming sidereal day and 40,000 km equator).
Eastward velocity $v$ of the aircraft is $V cos(a)$
Change in eastward velocity $v$ at 55N per change in $a$ is $dv/da = -V sin(a) = -380.6 m/s/radian$ (where + is eastward)
Multiply that by aircraft speed in radians per second (0.00003488),
and get $dv/dt = -0.01328 m/s/s$.
That's the coriolis acceleration.
So how much of a gravity is that? Divide by $9.8 m/s/s$ and you get -0.00135.
So the plane needs to bank left by that angle in radians.
Times 57.296 gives 0.0776 degrees of bank.

There's probably an easier way, but why do things the easy way?

Note what this means. The coriolis acceleration, and the bank angle, are proportional to the aircraft's speed in the northward direction. The bank angle going southward is the same. In the southern hemisphere the bank angle is reversed. At the equator, it is zero, and maximum at the poles.