0
$\begingroup$

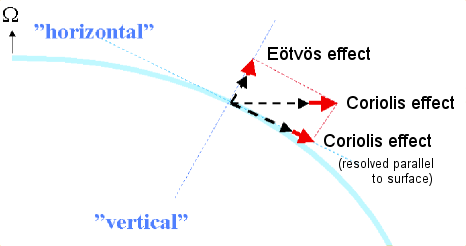
This is NOT a homework question, there are few informations that I found on the Internet about this specific case of the Coriolis Force

We currently are dealing with the Coriolis Force, and I struggle to understand how to calculate the force when moving east or west ALONG the Equator. I know that the force is zero when moving north or south at the Equator, because the Latitude Angle used to calculate the force is zero.

I know that generally $$F_c = -2m (\Omega \times \overrightarrow{v})$$ where Omega is the Angular Velocity, in the case of Earth it is $\frac{2\pi}{24\cdot 60 \cdot60}$ because we divide the period by the total time in seconds it takes for Earth to go around, so one day. And $\overrightarrow{v}$ is the velocity vector. The resulting force $F_c$ points perpendicular to both velocity and angular velocity

I also know that if moving tangetially along the Surface of earth, the formula is $$F_c = 2\cdot m\cdot v \cdot \omega \cdot \sin{\phi}$$ where $\phi$ is the latitude at which the object lies. So at the Equator, $\phi = 0$ so obvisouly $\sin\phi$ is also zero.

But there is a Coriolis Force when moving East or West along the Equator, I know it is directed inside the Earth when moving West, and directed toward outer space when moving East. But how I am supposed to calculate it in this case ? The latitude angle is zero, so I can't use the formula above even tough we are also moving tangetially along the surfcae of Earth

I would really appreciate any support, because either I didn't find it, or there are only few informations about this specific case of the Coriolis Force on the Internet

Thanks a loot for your help, feel free to comment if anything is unclear

Improve this question
$\endgroup$
1
  • $\begingroup$ Feel free to ask if anything is unclear $\endgroup$
    – wengen
    Commented Oct 4, 2022 at 9:05

1 Answer 1

Reset to default
3
$\begingroup$

You are supposed to calculate the Coriolis force using the vector equation you provided, not the latitude dependent resolution tangential to the Earth's surface.

See https://en.wikipedia.org/wiki/Eötvös_effect e.g.,:

enter image description here

At the equator the vector Coriolis effect is entirely vertical, so the portion resolved parallel to the surface is zero. This is all in the Earth-fixed frame.

In the frame of the moving object, there is no Coriolis effect (as $v=0$ in that frame), and the pseudo-force is called the Eötvös effect, which is a change in the apparent local gravity strength caused by a centrifugal force.

Improve this answer
$\endgroup$
2
  • $\begingroup$ So I can just take the Eotvos Formula with velocity in latitude direction = 0 and velocity in longitudinal direction equal to the velocity of the object because it moves east-west along the equator ? The phi angle in this case is also zero because it's the latitude angle, so $\cos\phi = 1$ ? The angular velocity is the same as above, that is 2pi/(24*60*60), and the radius of the earth is 6.378 * 10^6 meters ? This formula gives the acceleration, to get the force can I just mutiply by the mass of the object, so F = m*a ? This seems a bit too easy, am I missing something? Thanks for your support $\endgroup$
    – wengen
    Commented Oct 4, 2022 at 10:35
  • $\begingroup$ By the way, I would like to upvote your answer but I need at least 15 reputation to do so xD. As soon as I get them, I will upvote your answer $\endgroup$
    – wengen
    Commented Oct 4, 2022 at 10:42

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.