Tension at extreme of a rotating rope of mass $m$

Question

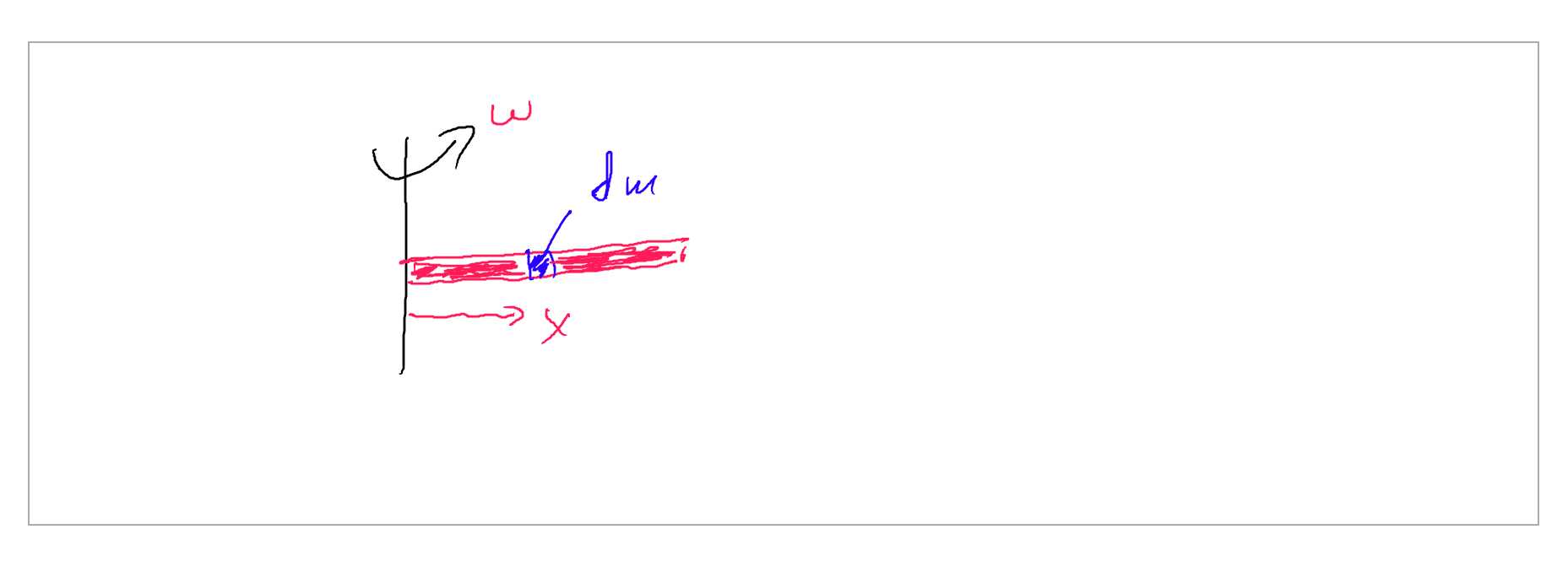
A uniform rope of mass per unit length $\lambda$, length $\ell$ is attached to shaft that is rotating at constant angular velocity $\omega$. Find the tension in the rope as a function of distance from the shaft. You may ignore the effect of gravitation.

In its solution, we can consider any general differential element $dx$ at a distance $x$ from the axis of rotation, and obtain the equation
$$ -dT=\lambda \omega^2 x dx $$
Once, we integrate it, we does it from $x$ to $\ell$ and take the corresponding tension from $T(x)$ to $0$.
But can anyone tell why $T(\ell) = 0$, cause without tension at the extreme end what can provide the the mass $dm$(at the extreme) the required centripetal force?
By the way , final answer is
$$T(x)=\frac{\lambda \omega^2(l^2-x^2)}{2}$$

Answer 1

You can also consider the problem in a global way by considering the piece of rope included between $x$ and $l$.

Its mass is $m(x)=\lambda (l-x)$ and its center of gravity is in ${{x}_{G}}=x+\frac{1}{2}(l-x)=\frac{1}{2}(l+x)$.

Its acceleration is $-m(x){{\omega }^{2}}{{x}_{G}}=-\lambda (l-x){{\omega }^{2}}\frac{1}{2}(l+x)=-\frac{1}{2}\lambda {{\omega }^{2}}({{l}^{2}}-{{x}^{2}})$

The tension of the rope is necessary to maintain this acceleration $T(x)=-\frac{1}{2}\lambda {{\omega }^{2}}({{l}^{2}}-{{x}^{2}})$

Maybe this way to consider the problem may help you to better understand why the tension is $0$ at the extremity or the rope : no mass to accelerate.

Sorry for my poor english !

Answer 2

The last little dm at the end of the rope only needs a proportional dF to keep it moving in a circle.

Since the whole idea of infinitesimals like dm is that they’re, well, infinitesimal, for macroscopic purposes they’re considered zero.

Answer 3

Tension due to the Centrifugal force is:

$T=m\,\omega^2\,x\quad \Rightarrow\quad dT=dm\,\omega^2\,x $

with:

$m(x)=\rho\,V=\rho\,A\,(l-x)\quad \Rightarrow\quad dm=-\rho\,A(x)\,dx$

for a constant area $A(x)=A$ we obtain:

$dm=-\rho\,A\,dx=-\lambda\,dx$

$\Rightarrow$

$dT=dm\,\omega^2\,x=-\omega^2\lambda\,x\,dx$

and

$T(x)=-\omega^2\lambda\int_x^l x'\,dx'=\frac{1}{2}\,\omega^2\lambda\left(x^2-l^2\right)$

Answer 4

If you had a 1 m length and divided into cm lengths you could use a spreadsheet to do the calculations (a good approximation). The cm closest to the shaft has high T due to all the masses attached to it. The piece at the end has no mass attached to it, in the limit (say we go mm size segments) the last piece has a very small λ.