You can't push a rope. No seriously, you can't because its weight is going to make it hang down in a catenary shape and you need to always pull to keep it in place.
The amount you need to pull is given by the catenary equations. Consider the hanging cable with dimensions shown below:
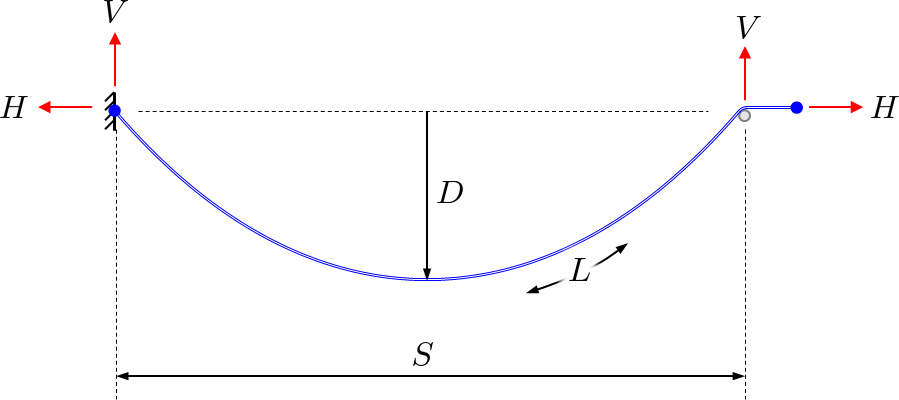
Actually given the pull force $H$, the remaining dimensions and values are derived from the catenary equations. You need a measure of the weight of the rope, and it is given as $w = \frac{m g}{L}$ usually, that is $w$ is weight per unit length. Also you must know $S$, the span where the rope hangs from.
I have summarized the catenary equations below, as well as their parabolic approximations
| Quantity |
Catenary |
Approximation |
| Catenary Constant, $a$ |
$ a = \frac{H}{w} $ |
|
| Catenary Shape |
$y = a \left( \cosh \left( \frac{ x-S/2}{a} \right) - \cosh \left( \frac{S}{2 a} \right) \right) $ |
$y = -\frac{w x (S-x)}{2 H}$ |
| Hanging Length, $L$ |
$L = 2 a \,\sinh \left( \frac{S}{2 a} \right)$ |
$L = S + \frac{w^2 S^3}{24 H^2}$ |
| Maximum Sag, $D$ |
$D = a \left( \cosh \left( \frac{S}{2 a} \right) -1 \right)$ |
$D = \frac{w S^2}{8 H}$ |
| Support Force, $V$ |
$V = H \sinh \left( \frac{S}{2 a} \right) $ |
$V = \frac{w S}{2} + \frac{w^3 S^3}{48 H^2}$ |
| Total Force, $T=\sqrt{H^2+V^2}$ |
$T = H \cosh \left( \frac{S}{2 a} \right) $ |
$T =H + \frac{w^2 S^2}{8 H}$ |
The above assume and inextensible rope.
So for example for a rope of length $L$ and mass $m$, you have $w= \frac{m g}{L}$ and using the parabolic approximation for $L$, you can solve for the pull force
$$ H = \sqrt{ \frac{ m^2 g^2 S^3 }{24 L^2 (L-S)} } $$
If you wanted to find the exact answer, you would need a numerical method to solve the catenary equations. Usually bisection works the fastest, but you can employ single point iteration
$$ H \leftarrow \frac{w S}{2} {\rm asinh}\left( \frac{w L}{2 H} \right) $$
The convergence of the the above isn't great, as after 40 iterations the error was still over 0.5% in a test case I did.
Some additional examinations and you will discover than $V = w \frac{L}{2}$ for both the exact and approx. equations. This makes sense as each $V$ holds up half the total weight $W = m g = w L = 2 V$
Also sag $D$ and total support force $T$ are related with $D = \frac{T-H}{w}$
Now specifically if you want to find the effective mass as seen from then end, what you can do assume a velocity profile for each part of the catenary that results in a given end velocity $v_0$ on the handle and equate the total kinetic energy to that of a lumped mass $m_{\rm eff}$ with velocity $v_0$
$$ KE = \tfrac{1}{2} m_{\rm eff}\,v_0^2 $$
You can consider the distribution of velocities as even along the rope, such that $$v(x) = v_0 \frac{ s(x) }{L} $$ where $s(x) = \int \limits_0^x \sqrt{1 + \left( \frac{ {\rm d}y}{{\rm d}x}\right)^2}\,{\rm d}x$ is the arc length of the rope at position $x$ from the left end.
The you assemble to total kinetic energy as
$$ KE = \int \limits_0^S \tfrac{m}{2 L} v(x)^2 \sqrt{1 + \left( \frac{ {\rm d}y}{{\rm d}x}\right)^2}\,{\rm d}x = \tfrac{1}{3} m v_0^2 $$
So the effective mass felt at the end of the rope under a catenary shape is
$$ \boxed{ m_{\rm eff} = \tfrac{1}{3} m } $$