Generally the path difference between two rays is considered as dsin$\theta and for this generally the two rays are considered parallel. That is shown in diagram 'c'.
My questions - 1. is even without approximation, the path difference looks the same if you look at diagram 'a'?
There are two questions in there. I will answer them in turn.
1) "The path difference looks the same". Not if I magnify the angles a bit:
The dashed line is part of a circle centered on the point P. The two blue legs are the same length (because they are both the radius of the circle). You can clearly see that there is an additional path (marked in red) to the top slit vs the bottom slit.
2) "How did we arrive at the angle marked in red is $\theta$ ?" Perhaps this construction will make that clear to you (there are two right-angled triangles there; the sum of the other two angles in a right-angled triangle is of course 90°).
Answer amended.
Your suspicions are right. It's not (quite) the same angle.
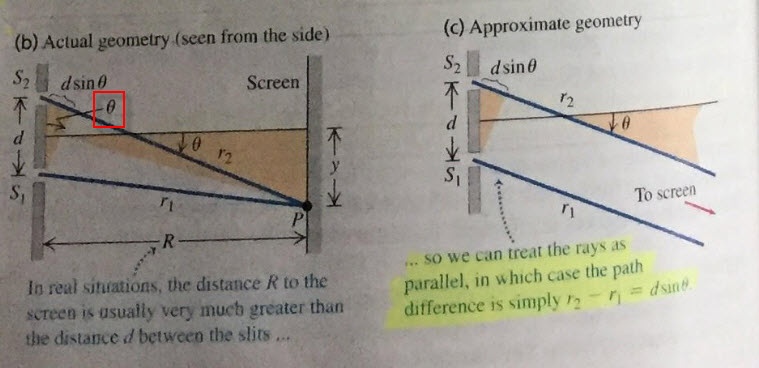
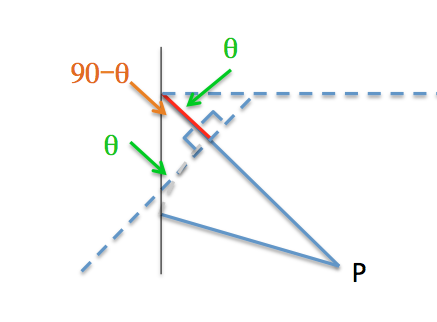
If you're looking for a treatment that is perhaps a more honest fudge, consider this one. In the left hand diagram draw a (dotted) line that goes from P through M, the point midway between the slits, and extend it a bit to the left. Then drop perpendiculars from the slits (S1 and S2) to this dotted line, hitting it at T1 and T2 (say). The distance T1 to T2, you'll be able to show easily, is exactly $d \sin \theta$. Then you need to convince yourself, by looking at the long thin triangles S1PT1 and S2PT2, that S1P is approximately equal to T1P and that S2P is approximately equal to T2P. If this is too hand wavy, try using the cosine formula in both of the long thin triangles!
What I like about this method is that
(a) It brings out the essential similarity between the geometries of Young's fringes and the diffraction grating (even though the first has a viewing plane at finite distance, but the second has a viewing plane effectively at infinity). [There's another, more algebraic, method of deriving the Young's fringes formula, based on Pythagoras, that makes it seem as though Young's fringes requires a totally different treatment from the grating.]
(b) The nature of the approximation being made is quite clear in this method.
Note: The error made by taking the path difference as $d \sin \theta$ is only 1% even if R is as small as 3$d$, if I remember rightly!
