I know that interference pattern maxima occur due to path difference of integer number of wavelengths, causing constructive interference, and minima due non-integer number of wavelengths, causing destructive interference.
What happens to the pattern if the waves from slits have a path difference? That is, two slits with path difference of 0.5 wavelength should arrive at the zeroth order maxima with destructive interference (non-integer wavelength). So will a central bright spot even exist?
As you have already said, what has been the central interference maximum becomes a minimum at 180 degrees phase difference. Generally speaking, the whole interference pattern will just shift under the broader shape of the intensity envelope. If you go all the way from 0 to 180 to 360 degrees phase difference, the interference pattern will have cyclically returned to its original position in the end.
By the way, a simple way to achieve such a phase shift for electron double-slit diffraction is by the Aharonov-Bohm effect. A magnetic field confined to a cylinder which does not significantly overlap with the electron wave functions, does actually change the wave function's phase according to the magnetic vector potential, which is non-zero even in regions of space where the magnetic field vanishes. Hence, in quantum mechanics the vector potential becomes a part of observable physical reality, although it was apparently only a practical construction without deeper meaning in classical physics. I personally find the Aharonov-Bohm effect one of the most exciting aspects of quantum mechanics. And the theory around this is also responsible for some things concerning superconduction.
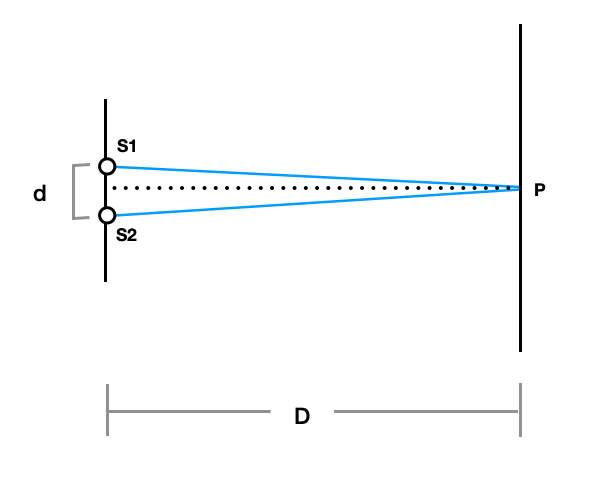
For usual scenario, where initially there is no path difference, the bright spot is obtained at the central point on the screen because the path difference is Zero. In the diagram below $S_1P$ - $S_2P$ = $0$, so at point P central bright spot is obtianed.
Let's say we place glass slab in front of one of the slits, of such a thickness which can introduce a path difference of $\frac{\lambda}{2}$ [To make visualisation easy I did this].
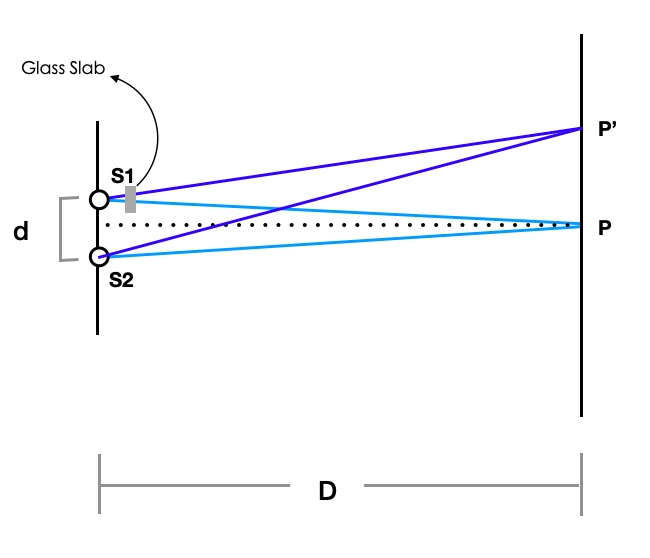
So in this case the bright spot has shifted to point $P'$. Answer to your first question, "What happens to the pattern if the waves from slits have a path difference?", is that wave pattern just shifts its position. As path difference was introduced near slit $S_1$ so, $S_1P'$ - $S_2P'$ = 0, which means light rays from slit $S_2$ will have to travel longer to form bright spot.
From a question may arise, how much did the pattern shift? Not difficult to answer, find $PP'$. $P'$ is the position where the $1st$ minima would have formed if there was no initial path difference.
