3
$\begingroup$

As part of a Geocaching puzzle, I have found these images, which supposedly are Feynman diagrams. I'm supposed to get numbers out of them. I've read some about particle physics but it is way far from my areas of knowledge. From what I've seen on many pages though, I'm starting to think these are bogus, i.e. they're just drawn to look like Feynman diagrams, but they aren't.
Just in case they really are, what do they represent? Is there any way to extract numbers out of them? (I thought something like getting the probability of those cases happening, although I'm not sure that can be calculated).

First diagram:

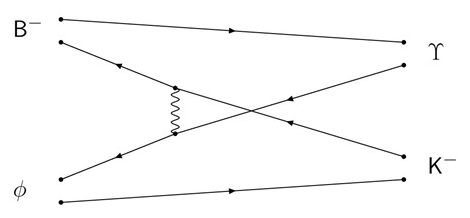
Apparently a Feynman Diagram

And second diagram:

Apparently another Feynman Diagram

EDIT: For the record, I contacted the cache owner, and he agreed than the lower arrow in the second diagram should be pointing backwards as suggested by gj255 on their answer.

Improve this question
$\endgroup$
1
  • $\begingroup$ You could perhaps extract numbers in the sense of discovery dates of the relevant particles? $\endgroup$
    – CDCM
    Commented Apr 10, 2018 at 22:07

1 Answer 1

Reset to default
3
$\begingroup$

The first diagram represents the process of a $B$ meson and a $\phi$ meson interacting to form an $\Upsilon$ meson and a $K$ meson.

In terms of the constituent quarks, this process is $$ b \bar{u} + s \bar{s} \to b \bar{b} + s \bar{u} \,,$$ where the overbar denotes antiparticle. There are many diagrams that lead to this process. We can leave the $b$ and $s$ untouched, if we can find a way to convert $\bar{u}$, $\bar{s}$ to $\bar{b}$, $\bar{u}$. This so-called flavour-changing process can only occur through exchange of a $W$ boson. In exchanging a $W$ boson, the $\bar{u}$ changes to a $\bar{b}$ and the $\bar{s}$ to a $\bar{u}$. These then exchange places in the diagram shown, but they shouldn't. Label the diagram with the quarks to convince yourself of this.

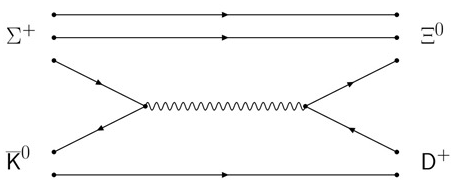
The second diagram represents the process of a $\Sigma$ baryon and a $K$ meson interacting to form a $\Xi$ baryon and a $D$ meson.

In terms of the constituent quarks, this process is

$$uus + s \bar{d} \to uss + c\bar{d} \,. $$ Again, there are many diagrams corresponding to this process. We can leave the $u$, $s$ and $\bar{d}$ untouched, if we can find a way to convert $u$, $s$ to $s$, $c$. Again, this can only occur through exchange of a $W$ boson, and in doing so, $u$ changes to $s$ and $s$ changes to $c$. However, $u$ and $s$ cannot annihilate into a $W$ boson as shown in the diagram – for instance, the electric charges do not add up. Indeed, a backwards pointing arrow in a Feynman diagram typically indicates an antiparticle, which suggests that it is the $\bar{d}$ annihilating with a quark from the $\Sigma$ baryon, not the $s$. But this leaves the $s$ of the $K$ meson to become a constituent of the $D$ meson, which does not make sense, on account that $D^+ = c\bar{d}$.

Conclusion: both of these processes are physically allowed, but the diagrams representing them are flawed. In principle, if the diagrams were drawn correctly, we could use the rules of quantum field theory to calculate the probability amplitudes corresponding to them, as you wish, but these would depend on parameters such as the energy the particles were collided at, and wouldn't yield pure numbers. I suspect you're looking for something less technical.

Improve this answer
$\endgroup$
7
  • $\begingroup$ Probably the solution to the puzzle are the charge mismatches? $\endgroup$
    – Ryan Thorngren
    Commented Apr 10, 2018 at 22:40
  • $\begingroup$ @RyanThorngren Could you please elaborate? $\endgroup$
    – gj255
    Commented Apr 10, 2018 at 22:54
  • $\begingroup$ For instance in the second diagram the missing electric charge is 2/3. $\endgroup$
    – Ryan Thorngren
    Commented Apr 10, 2018 at 23:20
  • $\begingroup$ @gj255 I understood parts of your answer. I see how every line can be labeled with a quark from each of the baryons and mesons. I see too the situation you describe in the last part, where taking a backwards arrow as an antiparticle leads to a contradiction for the s and c quarks for the lower arrow. What I don't understand (maybe this is out of the scope of the question) is how do the W boson interactions work. How do you know , in the first diagram, that $\bar{u}$ must change to $\bar{b}$ for example? I'm trying to understand these diagrams better. $\endgroup$
    – Arturo Vial Arqueros
    Commented Apr 11, 2018 at 4:10
  • 1
    $\begingroup$ @ArturoVialArqueros The $Z$ boson interacts similarly to the $W$ boson, except that it isn't allowed to change flavour. So a $u$ quark can emit a $Z$ boson, but it must stay as a $u$ quark. Likewise, an $s$ and an $\bar{s}$ can annihilate into a $Z$ boson, just as (for example) a $u$ and an $\bar{s}$ can annihilate into a $W$ boson. $\endgroup$
    – gj255
    Commented Apr 11, 2018 at 23:23

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.