3
$\begingroup$

Why is Hubble's Constant exactly the inverse of the age of the universe?

This cannot be coincidence. If according to our laws of physics this is coincidence then surely we have got something worked out wrong.

If this 'coincidence' were to continue, it proves that Hubble's Constant must be decreasing. Furthermore, does it imply that expansion if the universe is asymptotic?

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ The Wikipedia page on the age of the universe essentially holds your answer already. $\endgroup$
    – ACuriousMind ♦
    Commented Apr 23, 2017 at 10:07
  • $\begingroup$ Why is Hubble's Constant exactly the inverse of the age of the universe? It isn’t; it’s just close to it. Your premise is false. $\endgroup$
    – Ghoster
    Commented Nov 10, 2022 at 1:21

3 Answers 3

Reset to default
9
$\begingroup$

Hubble's constant is probably a little larger than the inverse of the age of the Universe. The reason they are close is somewhat of a coincidence, for example in the standard Lambda-CDM model of the Universe, in the far future the age of the Universe will become vastly larger than the inverse of the Hubble constant, because H asymptotically approaches a constant value.

The dynamics of the standard dark energy models, in which over time the accelerated expansion caused by dark energy dominates, but the expansion in the early Universe was decelerating due to the domination of radiation and then matter, means that at some point the inverse of the Hubble constant must equal the age of the Universe. It just so happens that in cosmological terms that point is very close to the present time and so the two are close to equal.

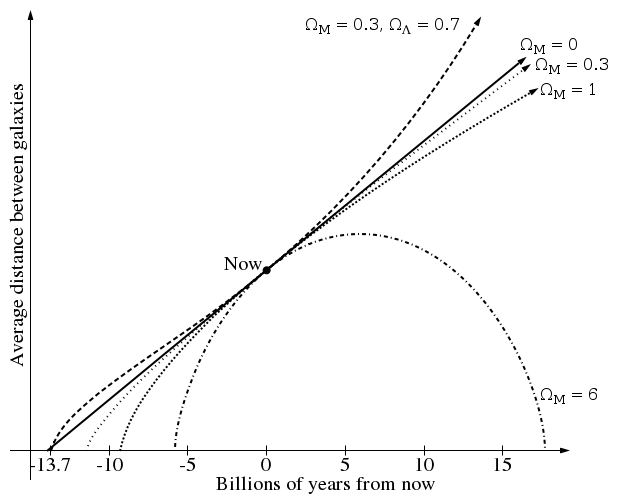
In the below graph from Wikipedia, you can see that the start of the curves representing $\Omega_M = 0$ (the Milne model, where the age of the Universe is the inverse of the Hubble constant) and $\Omega_M = 0.3, \Omega_{\Lambda} = 0.7$ (a standard cosmological constant model of dark energy) very nearly coincide.

enter image description here

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ Thanks in particular for the reference to the Milne model. Very interesting that the model fits GR $\endgroup$
    – Robert Frost
    Commented Nov 27, 2017 at 12:29
4
$\begingroup$

Hubble's law is the rate of expansion of the universe. If you assume it expanded from a point then the inverse is the time since it was zero size.

It's a little more complicated because the rate isn't constant with time , see Estimating age of the universe by Hubble's law?

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ The link is to a completely different question. I've corrected an error in my question. I meant Hubble's constant. $\endgroup$
    – Robert Frost
    Commented Apr 23, 2017 at 6:53
  • 1
    $\begingroup$ @RobertFrost The other question is closely related and the answer there explains why $1/H_0$ is a good estimate for the age of the universe. $\endgroup$
    – ACuriousMind ♦
    Commented Apr 23, 2017 at 10:09
2
$\begingroup$

For a 4-D sphere (or bubble) expanding radially at the speed of light:

r (radius) = 4230.53 Mpc (13.8 billion light years)

Given that c.2PI would also be the expansion around the "skin" (our 3D universe) then Hubble's constant can be calculated as rate of change over total change:

c.2PI / r.2PI = c/r = 70.864 (km/sec)/Mpc (Hubble's constant)

If you look more closely at c/r the units are inverse time.

In fact it simplifies to 1 / age of the universe.

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ sorry but I don't understand this answer. What does a 4D sphere have to do with the question? where did you get the number 13.8 from? are you perhaps assuming what you are actually trying to prove? What does 2PI mean? Why can Hubble's constant be calculated as that particular ratio, and how does that prove that $1/H\sim\text{age of Universe}$? $\endgroup$
    – AccidentalFourierTransform
    Commented Oct 15, 2017 at 12:19
  • 1
    $\begingroup$ @AccidentalFourierTransform I don't know who someguy is and I'm very surprised to see this answer but his answer has been my own personal theory for some time. If we consider space the be the surface of an expanding 4-sphere we get Hubble's law, the exact value of Hubble's constant, AND the big bang all automatically following. It also eliminates the paradox of "where is the edge of space" This ties in to my theory that everything moves at the speed of light; only its direction changes. All in all, by Occam's razor at least it smashes current theory out of the park. $\endgroup$
    – Robert Frost
    Commented Oct 15, 2017 at 15:39
  • 1
    $\begingroup$ The motivation of the question is to challenge this highly unlikely coincidence upon which current theory depends. $\endgroup$
    – Robert Frost
    Commented Oct 15, 2017 at 15:41

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.