3
$\begingroup$

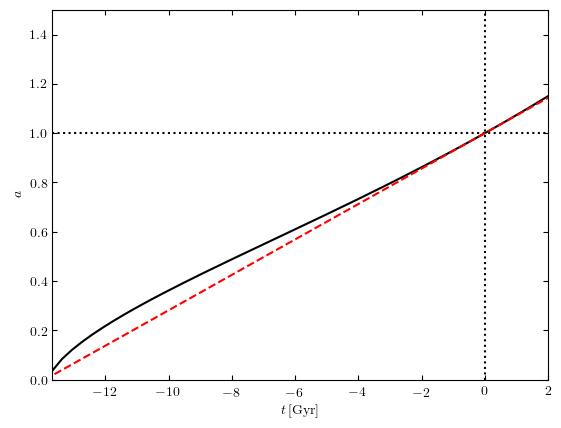
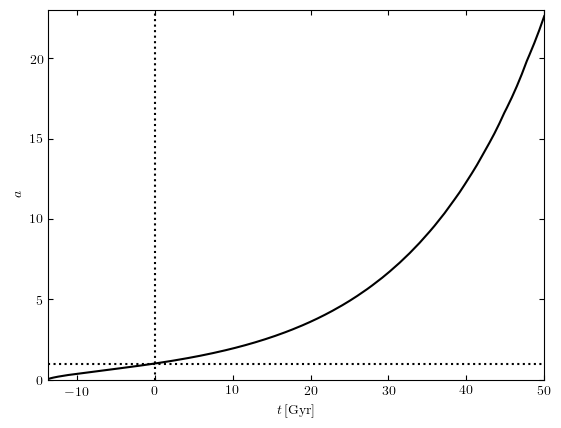
So I know from class that $\frac{1}{H_0}$ is an approximation for the age of the Universe, where $H_0$ is Hubble's Constant. Now let's say that a large amount of time passes - this means that Hubble's constant must fall in order to consistently remain an approximation for the age of the Universe.

However, I also learnt that the universe is expanding at an increasing rate (the expansion of the universe is accelerating). Since the recessional velocity is given by $v = H_0 d$, I would expect that in order for this equation to fit with the idea that the universe is expanding at an increasing rate, $H_0$ would actually increase over time in order to yield a greater recessional velocity and hence show that the expansion of the universe occurs at an increasing rate.

So why is it that $H_0$ falls over time?

Improve this question
$\endgroup$
3
  • $\begingroup$ Write down the full expression for $\dot{v}$. What condition must H satisfy for $\dot{v} > 0$? $\endgroup$
    – bapowell
    Commented Feb 8, 2018 at 22:14
  • $\begingroup$ Close to physics.stackexchange.com/questions/436985/… and others. $\endgroup$
    – ProfRob
    Commented Mar 14, 2019 at 7:22
  • $\begingroup$ It's a coincidence. We are living in a very special cosmic era, with Lady Gaga nominated for the Academy Award for Best Actress, silicon-based live forms beating carbon-based ones at go and chess, and $t_0 =1/H_0$ $\endgroup$
    – MadMax
    Commented Mar 14, 2019 at 13:46

1 Answer 1

Reset to default
4
$\begingroup$

The approximation of the age of the Universe as $t_H=1/H_0$ is valid only as long as the expansion history of the Universe is approximately linear. The black line in the figure below shows the expansion history so far (and $2\,{\rm Gyr}$ into the future) for our Universe, assuming the WMAP7 cosmology. The dotted lines mark today (which I've assigned $t=0$). Approximating the age as $t_H$ corresponds to taking the tangent to the curve today and extrapolating back to $a=0$, which I've shown with the red dashed line. As you can see it's pretty close, because the history is pretty close to linear.

Expansion history of the Universe and Hubble time approximation.

If the expansion history is strongly non-linear, extrapolating the tangent backward can be a very poor estimate of the age. For instance, in the same cosmology as above, look what happens in a few tens of $\rm Gyr$. At $t=50$ (an age of about $63\,{\rm Gyr}$), $t_H$ as the age would be an error by about a factor of $2$!

enter image description here

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.