More weight means more pulling force, and thus more tension force.
Like this answer says, however much force you pulling with is equal in magnitude to the tension force.
If that is case, how then can tensions forces in this photo be equal if their sources are of different weight:
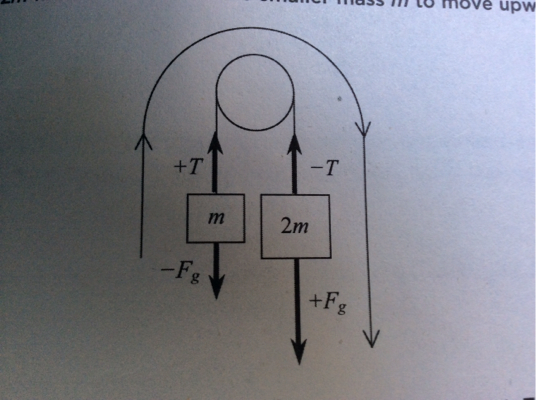
First of all, you say
how then can tensions forces in this photo be equal if their sources are of different weight
This shows a fundamental misunderstanding. The two weights are not the "sources" of the tension. The tension results from the interaction between the whole rope and both masses.
Somewhat expanding on the good answer from @Eeko, you might try the somewhat unusual approach of drawing the free body diagram for a small piece of the rope. Focus on a piece of the rope that isn't in contact with the pulley. What is this piece of rope touching? The only things it touches are the adjacent pieces of rope that it is attached to, and they can only exert tension forces on it (one tension up, the other down). The only other force that could act on this piece of rope is gravity.
Now, taking up as positive Newton's 2nd law reads:
$ma = T_1 - T_2 - mg$,
where $T_1$ and $T_2$ are the two tensions and $m$ here refers to the mass of this piece of rope. We usually approximate ropes as massless. So this gives us
$0 = T_1 - T_2$.
So the tensions exerted above and below this piece of rope have to be equal. Since this has to be true for any part of the rope the tension must be the same throughout the rope.
But look at why we got this result. We had to assume that the rope was massless. (tension the same everywhere in the rope is often called the "massless rope approximation) If you assume (more realistically...) that it isn't massless then $T_1 \neq T_2$. If the rope is heavy compared to the hanging masses then you can't get away with this approximation and the tension isn't the same everywhere in the rope. This makes the problem harder. Usually the massless rope approximation is a pretty good approximation and since it makes life so much easier we use it.
I think you're misunderstanding what it means when by "the pulling force equals the tension force". Imagine pulling on a rope with the other end not fixed to anything. Even if you pull with a large force, the tension in the rope will be zero, since the whole thing is accelerating due to that force. So what's important to understand from this is that tension is determined by pulling on both sides of the rope, not just either side individually.
Another helpful method for understanding why the tension must be the same all the way through, is to think of it as an equilibrium problem. Imagine a rope where the tension varies along it's length, for simplicities sake we'll say it varies evenly from a large T on the left, to a smaller t on the right. If we look at a small piece of the rope, it will have a larger force pulling it to the left, and a smaller force pulling to the right. So that one piece gets pulled to the left, which decreases tension on the left side, and increases it on the right.
Hopefully you can see from that example that having a rope with equal tension throughout is the only stable configuration.
There's no inconsistency because the system isn't in static equilibrium. The net force on the larger mass is $2mg-T$ and it will accelerate downwards. The net force on the smaller mass is $T-mg$ and it will accelerate upwards. If the string is inextensible, the two accelerations have equal magnitude and you can solve for $T$.
BTW, you shouldn't put plus or minus signs on a vector diagram: the arrow shows the direction and the accompanying number or letter shows the magnitude. A negative sign in particular is misleading (does the vector point in the negative coordinate direction or in the opposite sense to the arrow?)
There are two points to note:
Firstly, the tension T1 and T2 is equal only when the pulley and the rope are massless. If the pulley has mass=> it will have considerable inertia momentum. Due to its angular acceleration, its torque will be different from 0 , which implies that T1 and T2 is not equal. Also, if the rope is not massless, the tension will also be different. Imagine each part of the rope then acts as a mass. Then the tension is not the same everywhere in the rope.
Secondly, if all these conditions are satisfied, then T1 and T2 are equal. It is plausible as the two forces have two different functions,one acts as a retarding force, whereas the other is an accelerating force. It is such that the system can run at the same acceleration.
The job of the tension is to keep the masses at a fixed length apart. The weight (and weight difference) plays no direct role in determining the tension. Consider two connected weights in free fall. The tension would be zero because both are moving at the same rate (same acceleration).
So the job of the tension is to keep the rope length fixed, which causes the two objects to move "in lockstep". In your case as much one moves up the other must move down. If there was no rope then both would move down. The same tension value will have different effect on different weights, but that is not the total story. You need to consider the totality of forces applied on each object to determine its motion.
So if positive values point up you have two equations of motion and one constraint
$$ \begin{align} T - m_1 g & = m_1 a_1 \\ T - m_2 g & = m_2 a_2 \\ a_1 + a_2 & = 0 \end{align}$$
These three equations are solvable for the three unknowns, the tension $T$ and the two resulting motions $a_1$ and $a_2$.
